What Is The Length Of Ef
listenit
Apr 01, 2025 · 5 min read
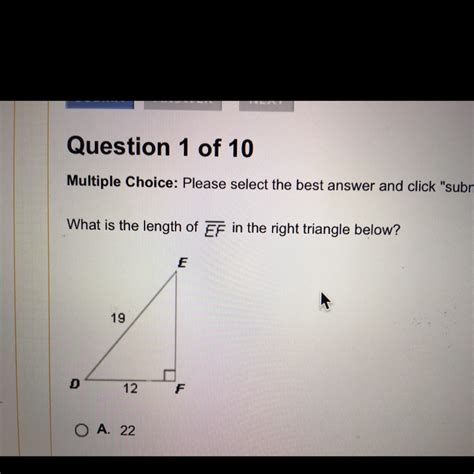
Table of Contents
- What Is The Length Of Ef
- Table of Contents
- Determining the Length of EF: A Comprehensive Guide
- Understanding the Problem's Context: The Importance of Additional Information
- Scenario 1: EF as a Side of a Triangle
- 1. Using the Pythagorean Theorem (Right-Angled Triangles)
- 2. Using the Law of Sines (Any Triangle)
- 3. Using the Law of Cosines (Any Triangle)
- Scenario 2: EF as a Side of a Quadrilateral
- 1. Parallelograms, Rectangles, Squares
- 2. Trapezoids
- 3. Other Quadrilaterals
- Scenario 3: EF as a Chord of a Circle
- Scenario 4: EF in Three-Dimensional Geometry
- Advanced Techniques and Considerations
- Conclusion: A Systematic Approach to Problem Solving
- Latest Posts
- Latest Posts
- Related Post
Determining the Length of EF: A Comprehensive Guide
The question "What is the length of EF?" is deceptively simple. It lacks context, making a definitive answer impossible without further information. This article will explore various scenarios where the length of EF might be asked, providing methods and formulas for calculating it in different geometric contexts. We'll cover various geometric figures, incorporating key mathematical concepts and focusing on effective problem-solving strategies. Understanding these methods will empower you to tackle similar problems with confidence.
Understanding the Problem's Context: The Importance of Additional Information
Before we can determine the length of EF, we need crucial contextual details. This could include:
- The type of geometric figure: Is EF a side of a triangle, a quadrilateral, a circle's chord, or part of another geometric shape? The shape dictates the relevant formulas and theorems.
- Known measurements: What other lengths, angles, or properties of the figure are known? These values are essential for calculations.
- Diagram or illustration: A visual representation is invaluable for understanding the relationships between points and segments.
Let's explore several common scenarios where calculating the length of EF might be necessary.
Scenario 1: EF as a Side of a Triangle
If EF is a side of a triangle, we can use various methods to determine its length, depending on what other information is available.
1. Using the Pythagorean Theorem (Right-Angled Triangles)
If triangle DEF is a right-angled triangle, with the right angle at D, and we know the lengths of the other two sides (DE and DF), we can use the Pythagorean theorem:
EF² = DE² + DF²
Therefore, EF = √(DE² + DF²)
Example: If DE = 3 units and DF = 4 units, then EF = √(3² + 4²) = √(9 + 16) = √25 = 5 units.
2. Using the Law of Sines (Any Triangle)
If we know two angles and the length of one side, or two sides and one angle (excluding the ambiguous case), the Law of Sines can be used:
EF/sin(∠D) = DE/sin(∠F) = DF/sin(∠E)
By rearranging the formula based on the known values, we can solve for EF.
Example: If ∠D = 60°, ∠E = 45°, and DE = 10 units, we can find EF. First, we calculate ∠F (180° - 60° - 45° = 75°). Then:
EF/sin(60°) = 10/sin(45°)
EF = (10 * sin(60°))/sin(45°)
This calculation will yield the length of EF.
3. Using the Law of Cosines (Any Triangle)
If we know the lengths of two sides and the angle between them, the Law of Cosines can be applied:
EF² = DE² + DF² - 2(DE)(DF)cos(∠D)
Therefore, EF = √(DE² + DF² - 2(DE)(DF)cos(∠D))
Example: If DE = 7 units, DF = 5 units, and ∠D = 120°, then:
EF = √(7² + 5² - 2(7)(5)cos(120°)) This calculation will give the length of EF.
Scenario 2: EF as a Side of a Quadrilateral
Determining the length of EF in a quadrilateral depends heavily on the type of quadrilateral.
1. Parallelograms, Rectangles, Squares
In parallelograms, opposite sides are equal. If EF is parallel and equal to another side (let's say, DG), then the length of EF is equal to the length of DG. In rectangles and squares, we have additional properties (right angles), but the fundamental principle of opposite sides being equal still holds.
2. Trapezoids
In trapezoids, only one pair of sides are parallel. Finding EF's length would require additional information, such as the lengths of other sides, the height of the trapezoid, or specific angles.
3. Other Quadrilaterals
For irregular quadrilaterals (those without specific properties), we might need more information about the angles and lengths of other sides to use trigonometric methods or vector calculations to find EF.
Scenario 3: EF as a Chord of a Circle
If EF is a chord of a circle, we need to know the radius of the circle and possibly the distance from the chord to the center of the circle.
If the chord is perpendicular to a radius that bisects it, we can use the Pythagorean theorem, treating the radius, half the chord length, and the distance from the center to the chord as the sides of a right-angled triangle.
Scenario 4: EF in Three-Dimensional Geometry
In three-dimensional space, determining the length of EF requires considering the coordinates of points E and F. We use the distance formula:
EF = √((x₂ - x₁)² + (y₂ - y₁)² + (z₂ - z₁)²)
Where (x₁, y₁, z₁) and (x₂, y₂, z₂) are the coordinates of points E and F, respectively.
Advanced Techniques and Considerations
For more complex scenarios, advanced techniques might be required.
- Coordinate Geometry: If points E and F are defined by coordinates, we can use the distance formula directly, as demonstrated above.
- Vectors: Vector methods can be employed, particularly in more complex geometric situations in two or three dimensions.
- Calculus: In certain cases involving curves, calculus might be necessary to find the length of a segment.
- Software and Tools: Geometric software and computational tools can aid in visualizing and calculating segment lengths in various contexts.
Conclusion: A Systematic Approach to Problem Solving
Determining the length of EF requires a systematic approach. Begin by carefully analyzing the given information and identifying the relevant geometric principles. Sketching a diagram is almost always beneficial. Select the appropriate formulas and techniques based on the information provided and the type of geometric figure involved. Always double-check your calculations and ensure the final answer makes sense within the context of the problem.
By understanding the different methods presented in this article, you'll be well-equipped to approach a wide range of problems involving the calculation of segment lengths, developing your problem-solving skills and deepening your understanding of geometry. Remember that the key to success is recognizing the context and utilizing the most appropriate mathematical tools available.
Latest Posts
Latest Posts
-
How Do I Graph X 2
Apr 05, 2025
-
Foreshadowing In Romeo And Juliet Act 1
Apr 05, 2025
-
5 Is 25 Of What Number
Apr 05, 2025
-
What Is 0 3 Repeating As A Fraction
Apr 05, 2025
-
Common Factors Of 4 And 10
Apr 05, 2025
Related Post
Thank you for visiting our website which covers about What Is The Length Of Ef . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.
