What Is The Inverse Function Of X 3
listenit
Apr 05, 2025 · 6 min read
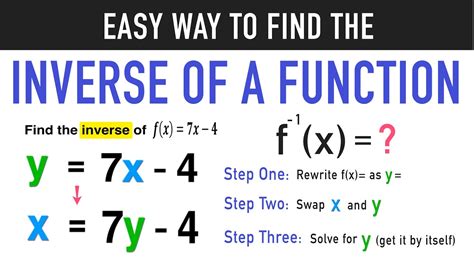
Table of Contents
What is the Inverse Function of x³? A Comprehensive Guide
The inverse function of a function essentially "undoes" what the original function does. If you apply a function and then its inverse function, you'll end up back where you started. This concept is crucial in mathematics, finding applications in various fields like calculus, algebra, and even computer science. Let's delve into the specifics of finding the inverse function of x³, exploring its properties, graph, and practical implications.
Understanding Functions and Their Inverses
Before tackling the inverse of x³, let's establish a solid understanding of functions and their inverses.
A function, denoted as f(x), is a relationship that assigns each input value (x) to a unique output value (y). For example, f(x) = x³ is a function because for every value of x, there's only one corresponding value of y (x cubed).
An inverse function, denoted as f⁻¹(x), reverses this process. If f(a) = b, then f⁻¹(b) = a. In simpler terms, if the function takes a and produces b, the inverse function takes b and produces a.
Not all functions have inverse functions. A function must be one-to-one (also called injective) to possess an inverse. This means each output value corresponds to only one input value. If a function maps multiple input values to the same output value, it's not one-to-one and doesn't have an inverse function. The function f(x) = x², for example, is not one-to-one because both 2 and -2 map to 4. However, by restricting the domain (for example, to only positive numbers), we can create a one-to-one function and find its inverse.
Finding the Inverse Function of x³
The function f(x) = x³ is a one-to-one function. For every input value, there's only one output value. Therefore, it has an inverse function. To find it, we follow these steps:
-
Replace f(x) with y: This gives us y = x³.
-
Swap x and y: This is the crucial step that reverses the function's operation. We get x = y³.
-
Solve for y: We need to isolate y to express the inverse function in terms of x. Taking the cube root of both sides, we have: y = ∛x.
-
Replace y with f⁻¹(x): This gives us the final form of the inverse function: f⁻¹(x) = ∛x.
Therefore, the inverse function of x³ is the cube root of x.
Graphical Representation of x³ and its Inverse
The graphs of a function and its inverse are reflections of each other across the line y = x. This means that if you fold the graph along the line y = x, the graph of the function will perfectly overlap the graph of its inverse.
The graph of f(x) = x³ is a cubic curve that passes through the origin (0,0) and increases steadily as x increases. The graph of its inverse, f⁻¹(x) = ∛x, is also a curve, but it's the reflection of the cubic curve across the line y = x. It also passes through the origin and increases steadily but at a slower rate than the original function. This symmetrical relationship visually confirms the inverse nature of these two functions. Plotting both functions on the same graph will clearly illustrate this reflection.
Properties of x³ and its Inverse
Both f(x) = x³ and f⁻¹(x) = ∛x possess several interesting properties:
-
Domain and Range: The domain and range of f(x) = x³ are both all real numbers (-∞, ∞). Similarly, the domain and range of f⁻¹(x) = ∛x are also all real numbers (-∞, ∞). This signifies that the functions are defined for any real number input, and the outputs can also be any real number.
-
Continuity and Differentiability: Both functions are continuous and differentiable everywhere. This means that their graphs have no breaks or jumps, and their derivatives exist at every point. The continuity and differentiability are preserved under the inverse operation.
-
Monotonicity: Both f(x) = x³ and f⁻¹(x) = ∛x are strictly increasing functions. This means that as x increases, y also increases. This property is preserved under inversion. A strictly increasing function will always have a strictly increasing inverse.
-
Odd Functions: Both functions are odd functions. This means that f(-x) = -f(x). In simpler terms, reflecting the graph across the y-axis and then across the x-axis results in the same graph. This symmetry property also extends to the inverse function.
Applications of Inverse Functions
The concept of inverse functions is not merely a theoretical exercise; it has far-reaching practical applications.
-
Solving Equations: Finding the inverse function is often crucial in solving equations. For example, if you have the equation x³ = 8, you can easily solve it by applying the inverse function: x = ∛8 = 2. This extends beyond simple equations to more complex scenarios involving cubic expressions.
-
Cryptography: Inverse functions play a critical role in cryptography. Encryption often involves applying a function to the data, and decryption uses the inverse function to recover the original data. The security of many encryption systems relies on the difficulty of finding the inverse function of a specific complex function.
-
Calculus: The concept of inverse functions is fundamental to understanding differentiation and integration, particularly when dealing with inverse trigonometric functions and logarithmic functions. Finding derivatives and integrals often requires manipulating functions and their inverses.
-
Data Analysis and Transformation: In data analysis, inverse functions can be used to transform data for easier analysis or visualization. If the data follows a cubic relationship, applying the cube root transformation (the inverse function) can often linearize the relationship, simplifying further analysis.
Beyond the Basics: Complex Numbers and the Cube Root
While the cube root function is well-defined for real numbers, extending it to complex numbers introduces an added layer of complexity. The equation z³ = w, where z and w are complex numbers, has three solutions for z, known as the three cube roots of w. Finding these roots requires employing techniques from complex analysis such as De Moivre's theorem.
Conclusion
Understanding the inverse function of x³, which is ∛x, is fundamental to a deeper grasp of mathematical concepts and their practical applications. The relationship between a function and its inverse, visualized through their graphs and characterized by their properties, allows us to approach problem-solving and data analysis with a more comprehensive understanding. From solving basic equations to more advanced applications in cryptography and calculus, the significance of inverse functions remains undeniable. This detailed exploration provides a comprehensive understanding of the function x³ and its inverse, ready to apply this knowledge to solve various problems and understand advanced mathematical concepts. Remember that exploring related concepts, such as complex numbers and their application to cube roots, provides even richer insights into this fascinating mathematical area.
Latest Posts
Latest Posts
-
Find The Exact Values Of X And Y
Apr 06, 2025
-
A Quadrilateral With All Sides Congruent
Apr 06, 2025
-
Identify The Two Key Factors That Determine Nuclear Stability
Apr 06, 2025
-
What Is Found In Plant Cells But Not Animal
Apr 06, 2025
-
Find The Measure Of Angle G
Apr 06, 2025
Related Post
Thank you for visiting our website which covers about What Is The Inverse Function Of X 3 . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.
