What Is Equivalent To X 2 3
listenit
May 10, 2025 · 5 min read
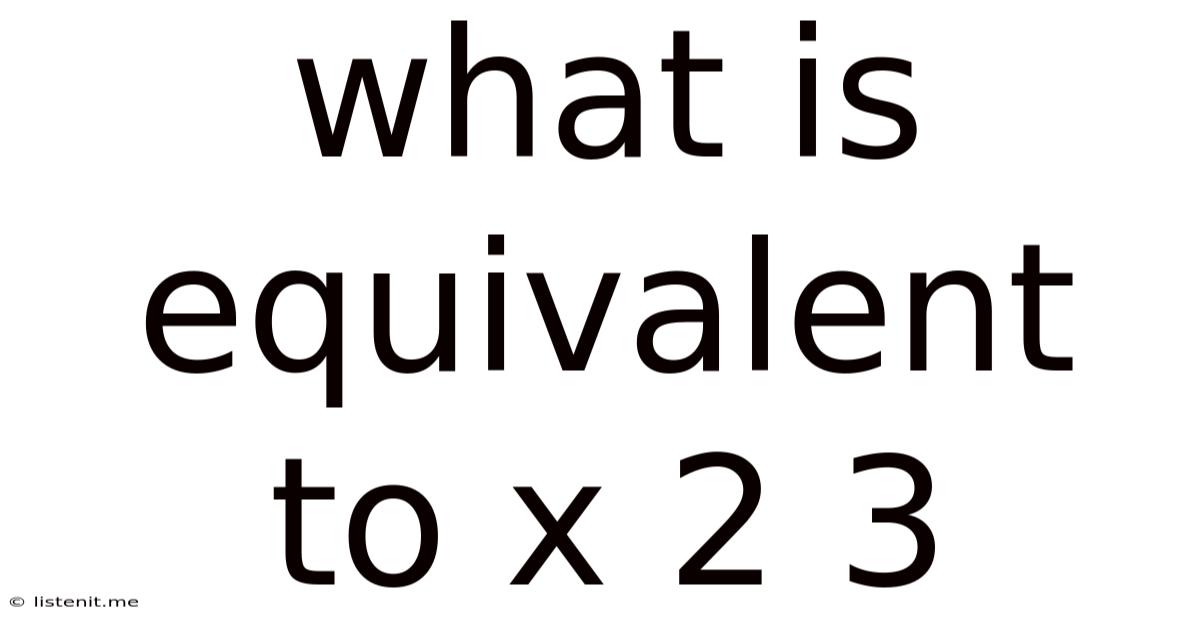
Table of Contents
What is Equivalent to x² + 3? A Deep Dive into Quadratic Expressions
The seemingly simple expression "x² + 3" opens a door to a vast world of mathematical concepts. While it might appear straightforward at first glance, understanding its equivalence and implications requires delving into the fundamentals of algebra, particularly quadratic expressions and their properties. This article will explore various aspects of x² + 3, examining its characteristics, potential manipulations, and its place within broader mathematical contexts.
Understanding the Basics: Quadratic Expressions
Before diving into the specifics of x² + 3, let's establish a foundational understanding of quadratic expressions. A quadratic expression is a polynomial of degree two, meaning the highest power of the variable (in this case, x) is 2. The general form of a quadratic expression is ax² + bx + c, where 'a', 'b', and 'c' are constants, and 'a' is not equal to zero (otherwise, it wouldn't be a quadratic).
Our expression, x² + 3, fits this mold with a = 1, b = 0, and c = 3. The absence of an 'x' term (b = 0) simplifies its appearance, but it doesn't diminish its mathematical significance.
Exploring the Equivalence: What Does "Equivalent" Mean?
In mathematics, equivalence means that two expressions represent the same value for all possible values of the variable(s). This doesn't necessarily mean they look identical; they can be written in different forms while maintaining the same outcome when evaluated. For example, 2 + 2 is equivalent to 4, even though they look different.
Therefore, finding an equivalent to x² + 3 means finding another expression that produces the identical result for any value substituted for 'x'. However, there are different types of equivalence to consider:
1. Equivalent through Algebraic Manipulation
We can't simplify x² + 3 any further through basic algebraic operations like combining like terms or factoring. The expression is already in its simplest form. Therefore, simple algebraic manipulation won't lead to a significantly different but equivalent expression.
However, we can rewrite it using different notation or highlighting specific properties. For example:
- Highlighting the constant term: We could emphasize the constant term by writing it as x² + √3² which highlights the relationship to perfect squares. This form is functionally equivalent but emphasizes the constant's square root relationship.
2. Equivalent through Functional Representation
We can represent x² + 3 as a function: f(x) = x² + 3. This functional notation doesn't change the expression's value but provides a different way of representing it, making it easier to discuss its properties such as its domain, range, and behavior.
3. Equivalent through Graphical Representation
The graphical representation of x² + 3 is a parabola. This parabola is shifted vertically upwards by 3 units compared to the basic parabola y = x². While not an algebraic equivalent, the graph visually represents the function and shows its equivalence in terms of its behavior and values.
Exploring Related Concepts: Parabolas and Quadratic Functions
Understanding the behavior of x² + 3 requires exploring the broader context of parabolas and quadratic functions. As mentioned earlier, its graph is a parabola opening upwards. Key characteristics include:
-
Vertex: The vertex of the parabola is at (0, 3). This is the lowest point on the graph.
-
Axis of Symmetry: The vertical line x = 0 (the y-axis) is the axis of symmetry. The parabola is symmetrical about this line.
-
Roots (or x-intercepts): The roots are the points where the parabola intersects the x-axis (where y = 0). In this case, there are no real roots because the parabola doesn't intersect the x-axis; the equation x² + 3 = 0 has no real solutions. The roots are complex numbers, ±i√3, where 'i' is the imaginary unit (√-1).
Solving Quadratic Equations Related to x² + 3
While x² + 3 itself is not an equation, we can create related quadratic equations and solve them to understand the connections. For example:
-
x² + 3 = 7: Subtracting 3 from both sides gives x² = 4, leading to x = ±2. This equation demonstrates that setting the expression equal to a specific value yields real solutions.
-
x² + 3 = 0: This equation has no real solutions, as already mentioned. The solutions are complex numbers: x = ±i√3. This highlights the concept of complex roots in quadratic equations.
-
ax² + 3 = k: Introducing a coefficient 'a' and a constant 'k' generalizes the equation to solve for 'x' in more complicated scenarios, demonstrating the applicability of solving quadratic equations that involve variations of the original expression.
Applications in Different Fields
The seemingly simple x² + 3 has applications in diverse fields:
-
Physics: Quadratic equations frequently appear in physics problems involving projectile motion, where the height of an object over time follows a parabolic path. A modified version of x² + 3 could represent a simplified model of this type of motion.
-
Engineering: Parabolas are used in the design of bridges, reflectors, and antennas due to their unique reflective properties. Understanding x² + 3 helps in analyzing these structures.
-
Computer Graphics: Parabolas are used in generating curves and shapes in computer graphics and animation. Mathematical expressions like x² + 3 are fundamental to creating these curves.
-
Economics and Finance: Quadratic models are sometimes employed in economic forecasting and financial modeling. While not directly equivalent to x² + 3, the fundamental principles of quadratic equations are applicable.
Expanding on the Concept: Beyond x² + 3
While we've focused on x² + 3, the concepts discussed extend to more complex quadratic expressions. The principles of solving quadratic equations, finding roots, and understanding graphical representations remain applicable, irrespective of the coefficients (a, b, c).
The core ideas of simplifying expressions, finding equivalent forms, and interpreting graphical representations remain vital tools in all mathematical fields. Understanding the nuances of even a simple expression like x² + 3 provides a solid foundation for tackling more advanced mathematical challenges.
Conclusion: A Simple Expression, Vast Implications
Although seemingly uncomplicated, x² + 3 offers valuable insights into the world of quadratic expressions, their properties, and their real-world applications. By exploring its characteristics, analyzing related equations, and considering its graphical representation, we uncover a richer understanding of its mathematical significance. This exploration underscores the importance of understanding even the most basic mathematical concepts, as their implications extend far beyond their initial appearance. The seemingly simple often holds the key to unlocking complex and fascinating mathematical realms.
Latest Posts
Latest Posts
-
Sodium Chloride And Lead Ii Nitrate
May 11, 2025
-
A Skateboarder Shoots Off A Ramp
May 11, 2025
-
50 Is 80 Of What Number
May 11, 2025
-
Draw Five Protons In The Nucleus Of The Atom
May 11, 2025
-
What Is The Slope Of The Line Shown
May 11, 2025
Related Post
Thank you for visiting our website which covers about What Is Equivalent To X 2 3 . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.