What Is 5 Out Of 12 As A Percentage
listenit
May 12, 2025 · 4 min read
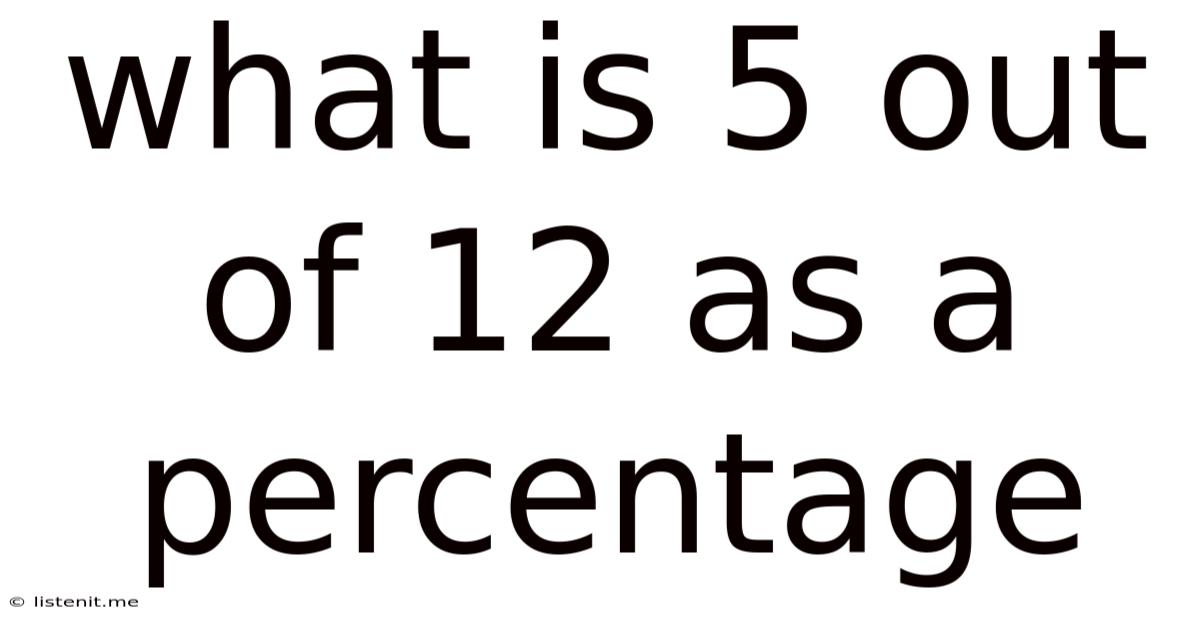
Table of Contents
What is 5 out of 12 as a Percentage? A Comprehensive Guide
Determining percentages is a fundamental skill applicable across numerous fields, from calculating discounts in a store to understanding statistical data in research papers. This comprehensive guide will delve into the process of calculating "5 out of 12 as a percentage," explaining the methodology, providing multiple approaches, and exploring related concepts. We'll also touch upon practical applications and address common misconceptions.
Understanding the Basics of Percentages
Before diving into the calculation, let's refresh our understanding of percentages. A percentage is a fraction or ratio expressed as a number out of 100. The symbol "%" represents "per cent," meaning "out of one hundred." For instance, 50% means 50 out of 100, which simplifies to 1/2 or 0.5.
Method 1: The Fraction Method
This is the most straightforward approach. We can express "5 out of 12" as a fraction: 5/12. To convert this fraction into a percentage, we need to transform the denominator (the bottom number) into 100. We do this by finding a number that, when multiplied by 12, equals 100. However, there's no whole number that satisfies this condition. Therefore, we'll use a different strategy.
We'll convert the fraction to a decimal first, and then multiply by 100 to obtain the percentage.
- Step 1: Divide the numerator by the denominator: 5 ÷ 12 ≈ 0.416666...
- Step 2: Multiply the decimal by 100: 0.416666... × 100 ≈ 41.67%
Therefore, 5 out of 12 is approximately 41.67%. The recurring decimal is rounded to two decimal places for practical purposes.
Method 2: The Proportion Method
This method utilizes the concept of proportions. We set up a proportion where x represents the percentage we are trying to find:
5/12 = x/100
To solve for x, we cross-multiply:
12x = 500
Then, we divide both sides by 12:
x = 500/12 ≈ 41.67%
This confirms the result obtained using the fraction method.
Method 3: Using a Calculator
Most calculators have a percentage function. You can simply input 5 ÷ 12 and then multiply the result by 100 to obtain the percentage. Some calculators even allow direct input of fractions, making the calculation even more straightforward.
Understanding the Significance of Rounding
In the calculations above, we rounded the decimal value to two decimal places (41.67%). The precision required depends on the context. For many practical applications, two decimal places are sufficient. However, in situations requiring higher accuracy, you might need to retain more decimal places or express the answer as a fraction.
Practical Applications of Percentage Calculations
The ability to calculate percentages is vital in numerous situations:
1. Business and Finance:
- Profit margins: Calculating the percentage of profit relative to the cost of goods sold.
- Sales tax: Determining the amount of tax to be added to a purchase.
- Discounts: Calculating the price reduction based on a percentage discount.
- Interest rates: Understanding the percentage of interest charged on loans or earned on investments.
- Financial ratios: Analyzing a company's financial health using various percentage-based ratios.
2. Science and Statistics:
- Data analysis: Representing data in percentage terms for easier understanding and comparison.
- Probability: Expressing the likelihood of an event occurring as a percentage.
- Surveys and polls: Reporting the results of surveys and polls as percentages.
3. Everyday Life:
- Tip calculations: Determining the appropriate tip amount in a restaurant.
- Grade calculations: Calculating your grade percentage in a class.
- Recipe scaling: Adjusting recipe quantities based on percentage increases or decreases.
Common Misconceptions about Percentages
- Adding percentages directly: It's incorrect to directly add percentages without considering the base value. For example, a 10% increase followed by a 10% decrease does not result in the original value.
- Confusing percentage change with absolute change: A percentage change represents the relative change, while an absolute change is the raw difference between two values.
- Improper use of percentages in comparisons: Comparing percentages without considering the base values can lead to misleading conclusions.
Advanced Concepts Related to Percentages
- Percentage increase/decrease: Calculating the percentage change between two values. The formula is: [(New Value - Old Value) / Old Value] x 100.
- Compounding percentages: Calculating the effect of repeated percentage changes over time. This is relevant in finance, particularly with compound interest.
- Percentage points: This refers to the absolute difference between two percentages, not the percentage change. For instance, an increase from 20% to 25% is a 5 percentage point increase, but a 25% increase relative to the initial value.
Conclusion
Calculating "5 out of 12 as a percentage" is a simple yet important skill. Understanding the different methods and their applications allows for accurate calculations and informed decision-making in various fields. By mastering these concepts and avoiding common misconceptions, you can confidently utilize percentages in your daily life and professional endeavors. Remember to always consider the context and the required level of accuracy when working with percentages, especially when dealing with complex scenarios involving compounding or multiple percentage changes. The ability to confidently navigate these calculations is a valuable asset in the modern world, impacting diverse aspects from personal finance to professional analyses.
Latest Posts
Latest Posts
-
Write The Balanced Chemical Equation For Photosynthesis
May 12, 2025
-
How Many Ounces In 1 05 Quarts
May 12, 2025
-
What Is 4 And 1 5 As A Decimal
May 12, 2025
-
Probability Density Function Of Chi Square Distribution
May 12, 2025
-
Do Isotopes Have The Same Chemical Properties
May 12, 2025
Related Post
Thank you for visiting our website which covers about What Is 5 Out Of 12 As A Percentage . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.