What Is 35 Out Of 50 As A Percentage
listenit
May 24, 2025 · 5 min read
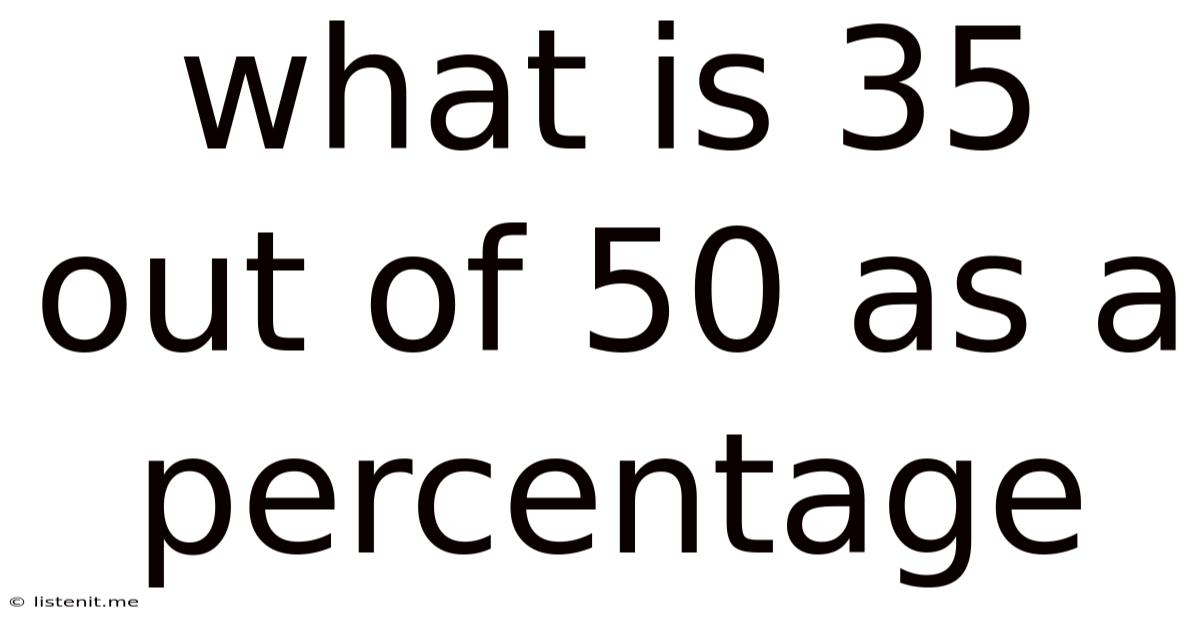
Table of Contents
What is 35 out of 50 as a Percentage? A Comprehensive Guide
Calculating percentages is a fundamental skill in many areas of life, from academics and finance to everyday decision-making. Understanding how to express a fraction as a percentage is crucial for interpreting data, comparing quantities, and understanding proportions. This article delves deep into the question: "What is 35 out of 50 as a percentage?" We'll not only provide the answer but also explore the underlying concepts, various methods of calculation, and practical applications.
Understanding Percentages
Before we dive into the specific calculation, let's establish a firm understanding of what percentages represent. A percentage is simply a fraction where the denominator is 100. It's a way of expressing a proportion or ratio as a number out of 100. The symbol "%" represents "per cent," which literally means "out of one hundred."
For example, 50% means 50 out of 100, which can also be written as the fraction 50/100 or the decimal 0.5. Percentages are used extensively because they provide a standardized way to compare different quantities and proportions, regardless of the original sizes of the quantities.
Calculating 35 out of 50 as a Percentage: The Basic Method
The most straightforward way to calculate what 35 out of 50 represents as a percentage involves two simple steps:
Step 1: Formulate the Fraction
First, we express the given numbers as a fraction: 35/50. This fraction represents the ratio of 35 to 50.
Step 2: Convert the Fraction to a Percentage
To convert a fraction to a percentage, we multiply the fraction by 100%. This is because multiplying by 100% is equivalent to multiplying by 1, and therefore doesn't change the value of the fraction, only its representation.
So, we have:
(35/50) * 100% = 70%
Therefore, 35 out of 50 is 70%.
Alternative Calculation Methods
While the above method is the most direct, several other approaches can be used to arrive at the same answer. These alternative methods can be helpful in different contexts and can enhance your understanding of percentage calculations.
Method 2: Simplifying the Fraction First
Before converting to a percentage, we can simplify the fraction 35/50. Both the numerator (35) and the denominator (50) are divisible by 5. Simplifying the fraction gives us:
35/50 = 7/10
Now, converting 7/10 to a percentage:
(7/10) * 100% = 70%
This method highlights that simplifying fractions can often make the conversion to percentages easier.
Method 3: Using Decimal Conversion
We can convert the fraction 35/50 to a decimal first and then convert the decimal to a percentage.
35/50 = 0.7
To convert a decimal to a percentage, we multiply by 100%:
0.7 * 100% = 70%
This method is particularly useful when working with calculators or when dealing with fractions that are not easily simplified.
Practical Applications of Percentage Calculations
Understanding percentage calculations has wide-ranging applications across various fields. Here are a few examples:
-
Academic Performance: If a student answers 35 out of 50 questions correctly on a test, their score is 70%. This allows for easy comparison of performance across different tests and students.
-
Financial Calculations: Percentages are fundamental in finance. Calculating interest rates, discounts, profit margins, tax rates, and investment returns all rely heavily on percentage calculations. For instance, if an item is discounted by 30%, the calculation of the final price involves percentage calculations.
-
Data Analysis and Statistics: Percentages are essential for summarizing and interpreting data. Representing data as percentages allows for easier comparison and visualization, making it easier to understand trends and patterns. For example, if 35 out of 50 respondents in a survey preferred a particular product, this could be represented as 70% preference.
-
Everyday Life: Percentages are encountered in many everyday scenarios. Tip calculations in restaurants, sale discounts in stores, and understanding nutritional information on food labels all involve percentage calculations.
Beyond the Basics: Advanced Percentage Problems
While calculating 35 out of 50 as a percentage is a relatively simple problem, understanding the underlying principles allows you to tackle more complex percentage problems. These can include:
-
Finding the percentage increase or decrease: This involves calculating the percentage change between two values. For example, if a price increases from $50 to $70, the percentage increase is calculated as [(70-50)/50] * 100% = 40%.
-
Calculating a percentage of a number: For example, finding 70% of 200 requires multiplying 200 by 0.7, resulting in 140.
-
Finding the original value after a percentage change: If a value has increased by 20% to become 60, the original value can be calculated using the equation: Original Value * 1.20 = 60. Solving this equation gives the original value as 50.
-
Solving problems involving multiple percentages: This can involve successive percentage changes, such as applying a discount and then adding a tax.
Mastering Percentage Calculations: Tips and Tricks
To improve your proficiency in percentage calculations, consider these tips:
-
Practice Regularly: Consistent practice is crucial for mastering any mathematical concept. Work through various percentage problems of varying complexity.
-
Understand the Concepts: Don't just memorize formulas; strive to understand the underlying principles of percentage calculations. This will enable you to approach different problems with confidence.
-
Utilize Online Resources: Many online resources, including websites and educational videos, provide practice problems and explanations of percentage calculations.
-
Use a Calculator Effectively: Calculators can be invaluable for complex percentage calculations, but understanding the underlying concepts remains important for checking your answers and avoiding errors.
Conclusion: The Power of Percentages
In conclusion, 35 out of 50 is equal to 70%. This seemingly simple calculation highlights the fundamental importance of understanding percentages in various aspects of life. From academic assessments to financial decisions and data analysis, the ability to confidently calculate and interpret percentages is a valuable skill that will serve you well in numerous contexts. By mastering the techniques and understanding the underlying concepts, you'll be well-equipped to tackle a wide range of percentage problems and confidently interpret the world around you. Remember, practice makes perfect, so keep working through problems and expanding your knowledge.
Latest Posts
Latest Posts
-
11 Out Of 25 As A Percent
May 25, 2025
-
What Is 3 Divided By 6
May 25, 2025
-
4 Divided By 12 In Fraction
May 25, 2025
-
26 2 Miles In 3 Hours Pace
May 25, 2025
-
What Is The Percentage Of 10 Out Of 25
May 25, 2025
Related Post
Thank you for visiting our website which covers about What Is 35 Out Of 50 As A Percentage . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.