What Is 30 Out Of 35 In Percentage
listenit
May 24, 2025 · 4 min read
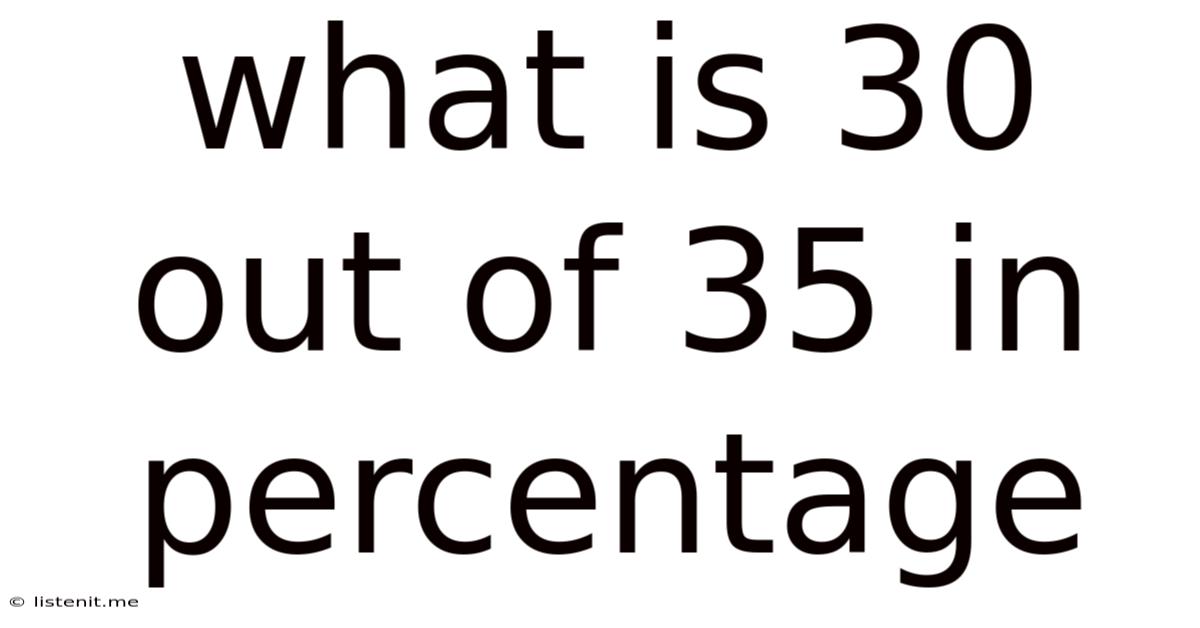
Table of Contents
What is 30 out of 35 in Percentage? A Comprehensive Guide
Calculating percentages is a fundamental skill applicable across numerous fields, from academic assessments to financial transactions and everyday life. Understanding how to determine the percentage one number represents of another is crucial for interpreting data, making informed decisions, and effectively communicating quantitative information. This article will delve into the specifics of calculating "30 out of 35 as a percentage," providing a detailed explanation, various calculation methods, and practical applications. We'll also explore related concepts and offer tips for mastering percentage calculations.
Understanding Percentages
A percentage is a way of expressing a number as a fraction of 100. The word "percent" itself is derived from the Latin "per centum," meaning "out of a hundred." Therefore, 50% means 50 out of 100, or 50/100, which simplifies to 1/2 or 0.5. Percentages provide a standardized and readily understandable way to represent proportions and ratios.
Calculating 30 out of 35 as a Percentage: The Basic Method
The most straightforward method involves using a simple formula:
(Part / Whole) x 100% = Percentage
In this case:
- Part: 30 (the number we're interested in)
- Whole: 35 (the total number)
Substituting these values into the formula:
(30 / 35) x 100% = 85.71% (approximately)
Therefore, 30 out of 35 is approximately 85.71%.
Alternative Calculation Methods
While the basic method is effective, other approaches can be equally useful, particularly when dealing with more complex scenarios or when aiming for a quicker calculation.
Method 1: Using Decimals
- Divide the part by the whole: 30 ÷ 35 = 0.8571 (approximately)
- Multiply the decimal by 100: 0.8571 x 100 = 85.71%
This method is especially efficient when using a calculator.
Method 2: Simplifying the Fraction
- Express the values as a fraction: 30/35
- Simplify the fraction: Both 30 and 35 are divisible by 5, resulting in 6/7.
- Convert the fraction to a decimal: 6 ÷ 7 ≈ 0.8571
- Multiply by 100%: 0.8571 x 100% ≈ 85.71%
Practical Applications of Percentage Calculations
Understanding how to calculate percentages is essential in a wide array of real-world situations. Here are some examples:
- Academic Performance: If a student scores 30 out of 35 on a test, their percentage score is 85.71%, providing a clear indication of their performance.
- Sales and Discounts: Determining the percentage discount on an item is crucial for consumers. For example, a 20% discount on a $100 item equates to a $20 reduction.
- Financial Analysis: Percentages are fundamental in understanding financial statements, including profit margins, return on investment (ROI), and interest rates.
- Data Analysis and Statistics: Representing data in percentage form makes it easier to compare and analyze trends and patterns. For instance, the percentage of respondents who prefer a particular product can be easily visualized and interpreted.
- Everyday Budgeting: Tracking expenses and savings as percentages of income facilitates better financial planning.
Beyond the Basics: Working with More Complex Percentages
The principles discussed above can be extended to more complex percentage calculations. For example:
- Calculating Percentage Increase or Decrease: This involves determining the percentage change between two values. The formula is: [(New Value - Old Value) / Old Value] x 100%.
- Finding the Original Value: If you know the percentage and the final value, you can work backward to determine the original value.
- Compounding Percentages: This is relevant in areas like compound interest calculations, where the percentage is applied repeatedly over time.
Tips for Mastering Percentage Calculations
- Practice Regularly: The more you practice, the more comfortable you'll become with these calculations. Try working through different examples.
- Use a Calculator: For more complex calculations, a calculator can significantly speed up the process and reduce the chance of errors.
- Understand the Concepts: Focusing on the underlying principles of percentages will allow you to approach problems systematically.
- Check Your Work: Always verify your answers to ensure accuracy.
Conclusion: The Significance of Percentage Calculations
The ability to calculate percentages is a valuable and versatile skill. Understanding how to determine "30 out of 35 as a percentage," and mastering various calculation methods, empowers individuals to analyze data effectively, make informed decisions, and communicate quantitative information clearly. The applications of percentage calculations extend far beyond simple arithmetic problems and are integral to academic success, financial literacy, and navigating the complexities of the modern world. By practicing and understanding the underlying concepts, you can confidently tackle percentage calculations in any context.
Latest Posts
Latest Posts
-
40 Of 35 Is What Number
May 24, 2025
-
How Old Is Someone From 1981
May 24, 2025
-
Greatest Common Factor Of 48 And 80
May 24, 2025
-
If Born In 1999 How Old Are You
May 24, 2025
-
How To Find 2 Sides Of A Right Triangle
May 24, 2025
Related Post
Thank you for visiting our website which covers about What Is 30 Out Of 35 In Percentage . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.