What Is 3 4 Times 5
listenit
May 09, 2025 · 5 min read
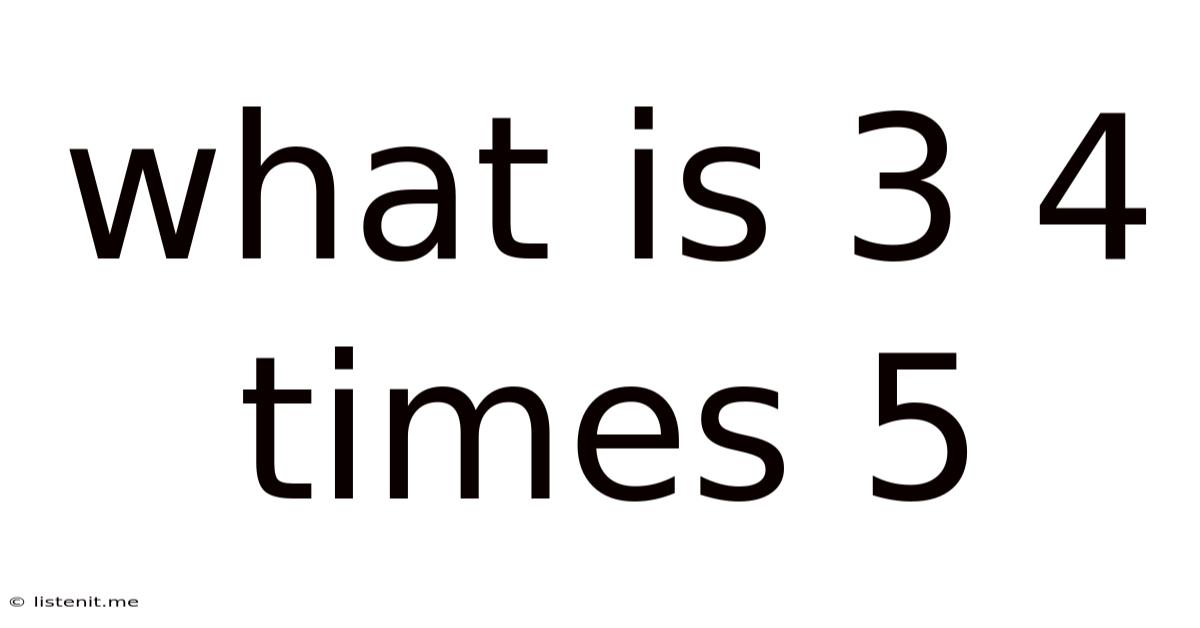
Table of Contents
What is 3/4 Times 5? A Deep Dive into Fraction Multiplication
This seemingly simple question, "What is 3/4 times 5?", opens the door to a deeper understanding of fraction multiplication, its applications in real-world scenarios, and the underlying mathematical principles. While the calculation itself is straightforward, exploring its nuances offers valuable insights for students and adults alike. This comprehensive guide will break down the process step-by-step, delve into the conceptual understanding, and provide examples to solidify your grasp of this fundamental mathematical operation.
Understanding Fractions: A Foundation for Multiplication
Before tackling the multiplication, let's refresh our understanding of fractions. A fraction represents a part of a whole. It consists of two numbers: the numerator, which sits on top and indicates the number of parts we have, and the denominator, which sits on the bottom and indicates the total number of equal parts the whole is divided into. In the fraction 3/4, 3 is the numerator and 4 is the denominator. This means we have 3 out of 4 equal parts.
Visualizing Fractions
Visualizing fractions helps solidify understanding. Imagine a pizza cut into four equal slices. The fraction 3/4 represents having three of those four slices. This visual representation makes it easier to grasp the concept and perform calculations.
Multiplying Fractions: The Method
Multiplying fractions is a relatively simple operation. It involves multiplying the numerators together and then multiplying the denominators together. The resulting fraction is the product.
The Formula:
(a/b) * (c/d) = (a * c) / (b * d)
Where 'a', 'b', 'c', and 'd' represent numbers.
Solving "3/4 Times 5"
Now, let's apply this to our question: "What is 3/4 times 5?"
First, we need to express the whole number 5 as a fraction. Any whole number can be written as a fraction with a denominator of 1. Therefore, 5 can be written as 5/1.
Now our equation becomes:
(3/4) * (5/1)
Following the formula for multiplying fractions:
- Multiply the numerators: 3 * 5 = 15
- Multiply the denominators: 4 * 1 = 4
This gives us the fraction 15/4.
Simplifying Fractions
The fraction 15/4 is an improper fraction because the numerator (15) is larger than the denominator (4). Improper fractions are often converted into mixed numbers for easier understanding. A mixed number combines a whole number and a proper fraction.
To convert 15/4 to a mixed number, we divide the numerator (15) by the denominator (4):
15 ÷ 4 = 3 with a remainder of 3.
This means that 15/4 is equivalent to 3 and 3/4. Therefore, 3/4 times 5 is 3 and 3/4 or 3.75 in decimal form.
Real-World Applications of Fraction Multiplication
Fraction multiplication isn't just a theoretical concept; it has numerous practical applications in everyday life:
- Cooking and Baking: Recipes often require fractions of ingredients. If a recipe calls for 3/4 cup of flour and you want to double the recipe, you'll need to multiply 3/4 by 2.
- Measurement and Construction: In construction and engineering, precise measurements are crucial. Fraction multiplication is used to calculate dimensions and quantities of materials.
- Finance and Budgeting: Understanding fractions is essential for managing finances. Calculating percentages, interest rates, and portions of budgets all involve fraction multiplication.
- Data Analysis: In statistics and data analysis, fractions are used to represent proportions and probabilities. Understanding fraction multiplication is vital for interpreting data accurately.
- Geometry and Area Calculations: Calculating the area of shapes often involves fractions, especially when dealing with irregular shapes or parts of shapes.
Beyond the Basics: Expanding Our Understanding
While we've solved the initial problem, let's explore some related concepts to further enhance our understanding:
Multiplying Fractions by Whole Numbers: Alternative Approaches
Instead of converting the whole number to a fraction, we can also solve the problem by considering the multiplication as repeated addition. 3/4 times 5 means adding 3/4 five times:
3/4 + 3/4 + 3/4 + 3/4 + 3/4 = 15/4 = 3 3/4
This approach can be particularly helpful for visualizing the multiplication process, especially for beginners.
Multiplying More Than Two Fractions
The principles of fraction multiplication extend to multiplying more than two fractions. We simply multiply all the numerators together and all the denominators together. For example:
(1/2) * (2/3) * (3/4) = (1 * 2 * 3) / (2 * 3 * 4) = 6/24
This fraction can then be simplified by dividing both the numerator and the denominator by their greatest common divisor (6), resulting in 1/4.
Cancelling Common Factors: Simplifying Before Multiplication
Before multiplying the numerators and denominators, it's often beneficial to cancel out any common factors. This simplifies the calculation and reduces the risk of working with large numbers. For example:
(3/4) * (4/5)
Notice that there's a '4' in both the numerator and the denominator. We can cancel these out:
(3/<s>4</s>) * (<s>4</s>/5) = 3/5
This makes the calculation much easier.
Conclusion: Mastering Fraction Multiplication
Understanding fraction multiplication is a cornerstone of mathematical proficiency. From its simple application in solving "3/4 times 5" to its complex utilization in various fields, mastering this concept is crucial for navigating numerous real-world situations. By understanding the underlying principles, employing visualization techniques, and practicing regularly, you can build a solid foundation in fraction manipulation and unlock a deeper appreciation for the power of mathematics. Remember to practice regularly with different examples, and don't hesitate to explore different methods to find the approach that works best for your learning style. The more you practice, the more confident and proficient you’ll become in working with fractions.
Latest Posts
Latest Posts
-
50 Is 80 Of What Number
May 11, 2025
-
Draw Five Protons In The Nucleus Of The Atom
May 11, 2025
-
What Is The Slope Of The Line Shown
May 11, 2025
-
Why Do Elements In A Group Have Similar Properties
May 11, 2025
-
How Many Faces In Rectangular Prism
May 11, 2025
Related Post
Thank you for visiting our website which covers about What Is 3 4 Times 5 . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.