What Is 3 4 Of 40
listenit
May 10, 2025 · 4 min read
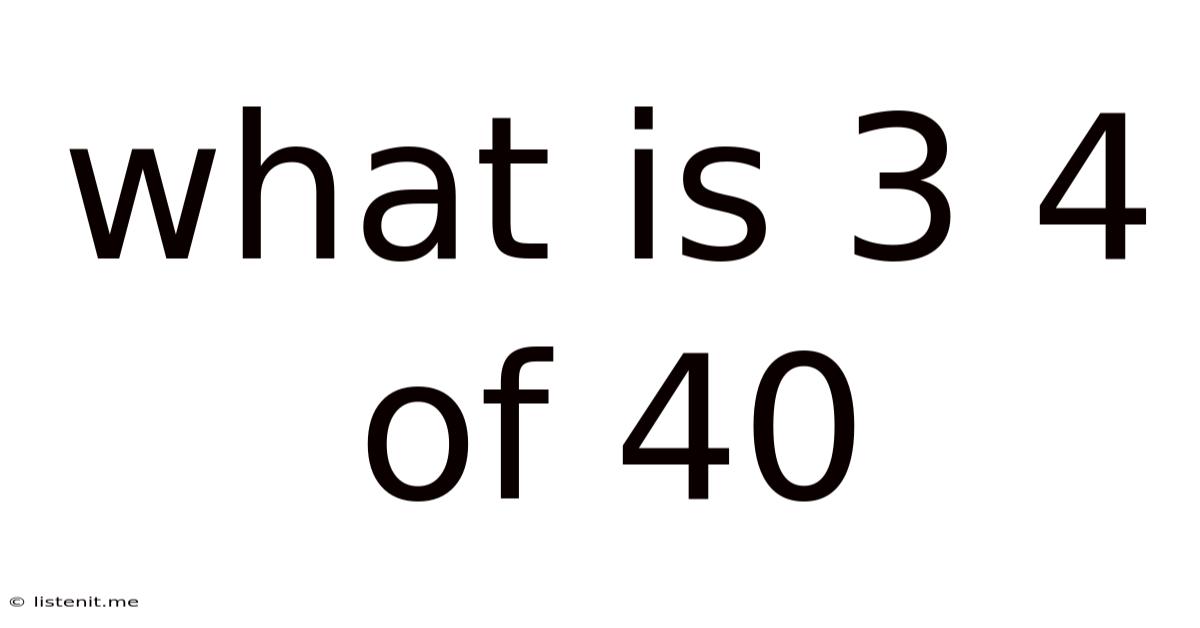
Table of Contents
What is ¾ of 40? A Deep Dive into Fractions and Their Applications
Finding ¾ of 40 might seem like a simple arithmetic problem, but it opens the door to understanding fundamental mathematical concepts with broad applications across various fields. This article will not only solve this specific problem but also delve into the underlying principles of fractions, percentages, decimals, and their practical uses in everyday life and specialized professions.
Understanding Fractions: The Building Blocks
A fraction represents a part of a whole. It's expressed as a ratio of two numbers: the numerator (top number) and the denominator (bottom number). The denominator indicates the total number of equal parts the whole is divided into, while the numerator indicates how many of those parts are being considered. In our case, ¾ represents three parts out of a total of four equal parts.
Key Fraction Concepts:
- Proper Fractions: The numerator is smaller than the denominator (e.g., ¾, ½).
- Improper Fractions: The numerator is greater than or equal to the denominator (e.g., 5/4, 6/6).
- Mixed Numbers: Combine a whole number and a proper fraction (e.g., 1 ¾).
- Equivalent Fractions: Fractions that represent the same value, despite having different numerators and denominators (e.g., ½ = 2/4 = 3/6).
Calculating ¾ of 40: Step-by-Step
There are several ways to calculate ¾ of 40. Let's explore the most common methods:
Method 1: Direct Multiplication
The simplest approach is to multiply the fraction by the whole number:
(¾) * 40 = (3/4) * 40
To perform this calculation, multiply the numerator (3) by 40, and then divide the result by the denominator (4):
(3 * 40) / 4 = 120 / 4 = 30
Therefore, ¾ of 40 is 30.
Method 2: Finding One-Fourth and Multiplying
Another approach involves finding one-fourth (¼) of 40 first and then multiplying the result by 3:
¼ of 40 = 40 / 4 = 10
Since ¾ is three times ¼, we multiply the result by 3:
10 * 3 = 30
Again, we arrive at the answer: ¾ of 40 is 30.
Expanding the Concept: Fractions, Decimals, and Percentages
Fractions, decimals, and percentages are interconnected and interchangeable representations of parts of a whole. Understanding their relationships is crucial for broader mathematical competency.
Converting Fractions to Decimals:
To convert a fraction to a decimal, divide the numerator by the denominator:
¾ = 3 ÷ 4 = 0.75
Converting Fractions to Percentages:
To convert a fraction to a percentage, multiply the decimal equivalent by 100:
0.75 * 100 = 75%
Therefore, ¾ is equivalent to 0.75 or 75%. This means ¾ of 40 is the same as 75% of 40. We can calculate this as:
(75/100) * 40 = 0.75 * 40 = 30
The result remains the same: 30.
Real-World Applications of Fractions, Decimals, and Percentages
These mathematical concepts aren't confined to textbooks; they are integral to numerous real-world scenarios:
Everyday Life:
- Cooking and Baking: Recipes often use fractions (e.g., ½ cup of sugar, ¾ teaspoon of salt).
- Shopping: Calculating discounts (e.g., 25% off), comparing prices, and understanding unit costs.
- Measurement: Working with inches, feet, meters, and other units involving fractions and decimals.
- Sharing: Dividing resources fairly among individuals or groups.
Professional Applications:
- Engineering: Precise calculations for building structures, designing machinery, and creating blueprints. Fractional dimensions are critical for accuracy.
- Finance: Calculating interest rates, determining loan repayments, analyzing investment returns, and understanding financial statements. Percentages and decimals are crucial in financial modeling and risk assessment.
- Science: Measuring quantities, interpreting experimental data, and expressing results. Scientific research often uses very precise decimal numbers.
- Data Analysis: Interpreting statistical data, creating charts and graphs, calculating probabilities and statistical significance.
- Computer Science: Representing numbers, images, and sounds using binary representations which is essentially a fractional system (base 2).
Beyond the Basics: Advanced Fraction Operations
While finding ¾ of 40 is a straightforward calculation, understanding more complex fraction operations expands mathematical capabilities significantly.
Adding and Subtracting Fractions:
To add or subtract fractions, they must have a common denominator. If they don't, find the least common multiple (LCM) of the denominators and convert the fractions accordingly.
For example: ½ + ¼ = (2/4) + (1/4) = 3/4
Multiplying and Dividing Fractions:
Multiplying fractions is simpler; multiply the numerators together and the denominators together.
For example: (½) * (¼) = 1/8
Dividing fractions involves inverting the second fraction (reciprocal) and multiplying.
For example: (½) ÷ (¼) = (½) * (4/1) = 2
Conclusion: The Significance of a Simple Calculation
While seemingly trivial, the calculation of ¾ of 40 underscores the importance of understanding fractions, decimals, and percentages. These concepts are fundamental to numerous aspects of life, from everyday tasks to complex professional applications. Mastering these basic mathematical operations lays the foundation for advanced mathematical concepts and successful problem-solving in various fields. The ability to confidently perform these calculations empowers individuals to tackle challenges, make informed decisions, and participate fully in a quantitatively driven world. Remember, even the simplest calculations can unlock doors to deeper understanding and broader applications.
Latest Posts
Latest Posts
-
What Is The Organelle Containing Digestive Enzymes
May 10, 2025
-
4x Y 1 In Slope Intercept Form
May 10, 2025
-
What Is 8 15 As A Decimal
May 10, 2025
-
Are Energy And Frequency Directly Proportional
May 10, 2025
-
How Do You Find The Density Of A Rock
May 10, 2025
Related Post
Thank you for visiting our website which covers about What Is 3 4 Of 40 . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.