What Is 25 Percent Off 50
listenit
May 23, 2025 · 5 min read
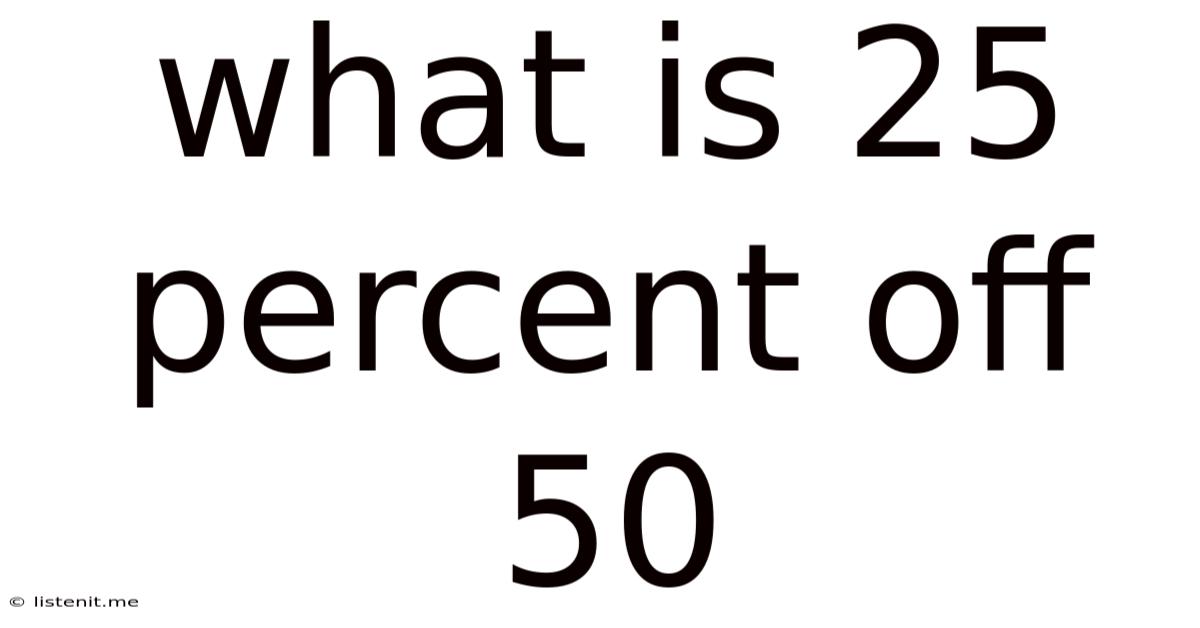
Table of Contents
What is 25 Percent Off 50? A Comprehensive Guide to Percentage Calculations
Calculating discounts is a fundamental skill in everyday life, whether you're shopping for groceries, comparing prices, or negotiating deals. Understanding how to determine the final price after a percentage discount is crucial for making informed financial decisions. This article will delve into the specifics of calculating 25 percent off 50, explaining the process step-by-step and exploring various methods to arrive at the answer. We'll also explore the broader context of percentage calculations and provide practical applications to help you master this essential skill.
Understanding Percentage Discounts
A percentage discount represents a reduction in the original price of an item. It's expressed as a fraction of 100, indicating how much less you'll pay. For example, a 25% discount means you'll pay 75% (100% - 25%) of the original price. In the case of "25 percent off 50," we're dealing with a 25% reduction from an initial price of 50 units (which could be dollars, pounds, euros, or any other currency).
Method 1: Calculating the Discount Amount
The most straightforward way to calculate the discount is to find 25% of 50 and then subtract that amount from the original price.
Step 1: Find 25% of 50
To find 25% of 50, we can convert the percentage to a decimal by dividing it by 100:
25% = 25/100 = 0.25
Now, multiply this decimal by the original price:
0.25 * 50 = 12.5
This calculation reveals that the discount amount is 12.5 units.
Step 2: Subtract the Discount from the Original Price
Subtract the discount amount (12.5) from the original price (50):
50 - 12.5 = 37.5
Therefore, 25 percent off 50 is 37.5.
Method 2: Calculating the Remaining Percentage
This method involves calculating the percentage of the original price you will pay after the discount.
Step 1: Determine the Remaining Percentage
If you receive a 25% discount, you will pay 100% - 25% = 75% of the original price.
Step 2: Calculate 75% of 50
Convert 75% to a decimal:
75% = 75/100 = 0.75
Multiply this decimal by the original price:
0.75 * 50 = 37.5
This directly gives you the final price after the discount, which is 37.5.
Method 3: Using Fractions
Percentages can also be expressed as fractions. 25% is equivalent to ¼ (one-quarter).
Step 1: Express the Percentage as a Fraction
25% = ¼
Step 2: Calculate the Discount using the Fraction
Multiply the original price by the fraction representing the discount:
¼ * 50 = 12.5
This gives you the discount amount (12.5).
Step 3: Subtract the Discount
Subtract the discount from the original price:
50 - 12.5 = 37.5
The final price after the discount is 37.5.
Practical Applications and Real-World Examples
Understanding percentage discounts is valuable in various real-world situations:
-
Shopping: Identifying the best deals while shopping requires quick percentage calculations. Websites and stores frequently advertise discounts, and being able to quickly calculate the final price saves time and prevents impulse purchases.
-
Sales Tax: Calculating the final price including sales tax involves adding a percentage to the original price (or discounted price).
-
Tips and Gratuities: Calculating tips in restaurants or expressing appreciation for services often involves determining a percentage of the total bill.
-
Investment Returns: Understanding percentage increases and decreases is critical when tracking investment performance. Annual growth or loss is usually expressed as a percentage.
-
Finance: Percentage calculations are fundamental in understanding interest rates on loans, mortgages, and savings accounts.
Advanced Percentage Calculations
While the example of "25 percent off 50" is relatively simple, the same principles apply to more complex scenarios:
-
Multiple Discounts: Sometimes you encounter multiple discounts applied sequentially (e.g., 20% off, then an additional 10% off). In such cases, apply the discounts one after another to the progressively reduced price.
-
Discounts on Discounted Prices: Be careful when interpreting promotional language. A phrase like "25% off the already reduced price" means the discount is applied to a lower price, not the original price.
-
Compound Interest: Calculating compound interest involves repeatedly applying a percentage increase to a principal amount over time. This is a more complex application of percentage calculations.
Mastering Percentage Calculations: Tips and Tricks
Here are some useful tips for improving your ability to calculate percentages quickly and accurately:
-
Memorize common percentages: Learning the decimal and fractional equivalents of common percentages (e.g., 10%, 25%, 50%, 75%) will significantly speed up your calculations.
-
Use a calculator: For complex calculations or multiple discounts, using a calculator ensures accuracy.
-
Practice regularly: The more you practice calculating percentages, the faster and more comfortable you'll become. Try working through various examples, including different discount amounts and original prices.
-
Break down complex problems: If faced with a complicated percentage problem, break it down into smaller, manageable steps. This will make the process less daunting and help you avoid errors.
Conclusion
Understanding how to calculate 25 percent off 50, and percentages in general, is an essential life skill. This article has detailed various methods for achieving this, from simple subtraction to using fractions and decimal conversions. By mastering these techniques and practicing regularly, you'll be equipped to handle various percentage calculations with confidence, enabling you to make informed decisions in your daily life and beyond. Remember, the key is to understand the underlying principles and to break down complex problems into simpler steps. With practice, these calculations will become second nature.
Latest Posts
Latest Posts
-
4 Divided By 3 In Fraction
May 23, 2025
-
What Is 80 000 After Taxes
May 23, 2025
-
What Is 20 Percent Of 76
May 23, 2025
-
6 Is What Percent Of 22
May 23, 2025
-
5 8 Divided By 1 2
May 23, 2025
Related Post
Thank you for visiting our website which covers about What Is 25 Percent Off 50 . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.