What Is 16 Of 43 Minutes
listenit
May 25, 2025 · 5 min read
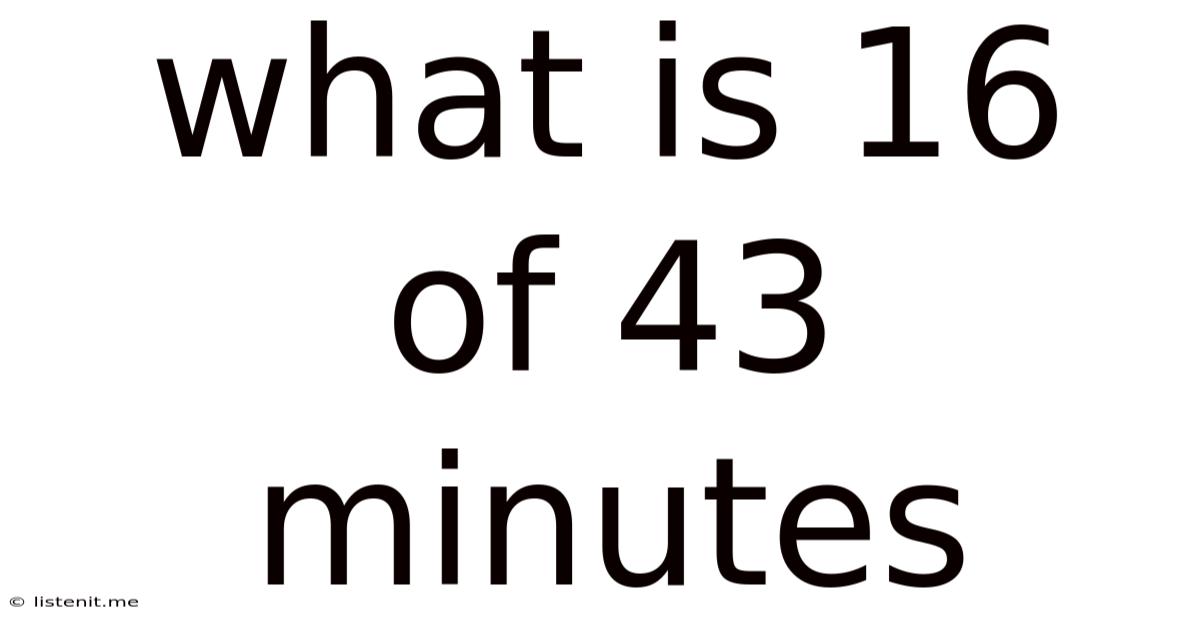
Table of Contents
What is 16 of 43 Minutes? A Deep Dive into Fractions and Time
Understanding fractions is a fundamental skill, applicable across numerous areas of life, from baking and cooking to complex mathematical equations and, as we'll explore today, even time management. This article tackles the seemingly simple question: "What is 16 of 43 minutes?" But instead of simply providing the answer, we will delve into the underlying principles, explore different methods of calculation, and uncover the broader implications of this type of problem. This comprehensive guide will equip you with the tools to confidently tackle similar fractional time calculations in the future.
Understanding the Problem: Fractions and Time
The question, "What is 16 of 43 minutes?", presents a classic fractional calculation problem involving time. It essentially asks us to determine a portion (1/6th) of a specific duration (43 minutes). This type of problem requires a clear understanding of fractions and their application in real-world scenarios.
Key Terms:
- Fraction: A number expressed as a quotient, in which a numerator is divided by a denominator. In our case, 1/6 is the fraction, with 1 being the numerator and 6 being the denominator.
- Numerator: The top number in a fraction, indicating how many parts we're considering.
- Denominator: The bottom number in a fraction, indicating the total number of parts in a whole.
- Time: The duration or interval measured in minutes, hours, seconds, etc. In this case, our time unit is minutes.
Method 1: Direct Calculation using Multiplication
The most straightforward method to solve "What is 1/6 of 43 minutes?" is through direct multiplication:
-
Convert the fraction to a decimal: 1/6 ≈ 0.1667 (We'll use a rounded decimal for simplicity. For higher accuracy, use more decimal places).
-
Multiply the decimal by the total time: 0.1667 * 43 minutes ≈ 7.1671 minutes
-
Convert the decimal portion of the minutes into seconds: 0.1671 minutes * 60 seconds/minute ≈ 10 seconds (Rounding again for practicality)
Therefore, 1/6 of 43 minutes is approximately 7 minutes and 10 seconds.
Method 2: Fraction Manipulation and Simplification
A more precise method avoids early rounding by keeping the fraction intact until the final step.
-
Express the problem mathematically: (1/6) * 43 minutes
-
Perform the multiplication: This gives us 43/6 minutes.
-
Convert the improper fraction to a mixed number: Divide 43 by 6. This results in 7 with a remainder of 1. Therefore, 43/6 = 7 1/6 minutes.
-
Convert the fractional part of the minutes into seconds: (1/6) * 60 seconds = 10 seconds.
This method yields the same result: 7 minutes and 10 seconds. Note the precision advantage; we only rounded at the very end.
Method 3: Using Proportions
The concept of proportions provides another powerful approach. We can set up a proportion to solve the problem:
1/6 = x / 43
Where 'x' represents the unknown portion (in minutes) of 43 minutes that constitutes 1/6.
To solve for x, we cross-multiply:
6x = 43
x = 43/6 minutes
This leads us back to the same mixed number, 7 1/6 minutes, which we convert to minutes and seconds as described in Method 2.
Extending the Concept: Applications and Further Exploration
Understanding how to calculate fractions of time is crucial in various contexts:
-
Project Management: Allocating time for tasks within a project. If a project takes 43 minutes and one sub-task represents 1/6 of the total time, then you know this sub-task will take roughly 7 minutes and 10 seconds.
-
Scheduling: Determining the duration of specific events within a schedule. For example, allocating a portion of a meeting for a particular topic.
-
Recipe Scaling: Adjusting cooking times based on recipe scaling. If a recipe calls for 43 minutes of cooking time, and you only want to make 1/6 of the recipe, you'll need about 7 minutes and 10 seconds of cooking time.
-
Data Analysis: Working with time-series data involving fractions of time units.
-
Travel Planning: Estimating travel times based on fractional portions of a journey.
Further Exploration:
-
More complex fractions: Practicing with more complex fractions will improve your understanding and speed. Try calculating 3/8 of 43 minutes or 2/5 of an hour.
-
Different time units: Extend your understanding by working with problems involving hours, seconds, days, etc. For example, calculate 1/6 of 2 hours and 15 minutes. This requires converting the time to a consistent unit (e.g., minutes) before performing the calculation.
-
Real-world problem solving: Apply your skills to practical scenarios. Imagine you're planning a road trip. You estimate the whole trip will take 43 minutes and you need to factor in a 1/6th break. Knowing this equates to approximately 7 minutes and 10 seconds lets you plan more effectively.
Conclusion: Mastering Fractions and Time Calculation
The seemingly simple question of "What is 1/6 of 43 minutes?" unveils a wealth of mathematical concepts and practical applications. By mastering the methods outlined above, you gain a robust skillset applicable across numerous domains. Remember, consistent practice is key to building confidence and fluency in fractional calculations involving time. Through understanding the fundamentals and exploring various solution methods, you’re equipped to tackle similar problems with ease and accuracy. This foundational knowledge will serve you well in numerous academic, professional, and everyday situations. So, next time you encounter a fractional time calculation, remember the techniques and steps outlined in this comprehensive guide, and conquer the challenge with confidence!
Latest Posts
Latest Posts
-
Least Common Multiple Of 10 And 7
May 25, 2025
-
Car Payoff Calculator With Extra Payments
May 25, 2025
-
28 What Year Was I Born
May 25, 2025
-
24 Is What Percent Of 200
May 25, 2025
-
Buy 2 Get 1 Free Discount Percentage
May 25, 2025
Related Post
Thank you for visiting our website which covers about What Is 16 Of 43 Minutes . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.