What Is 1 4 Of 30
listenit
May 24, 2025 · 5 min read
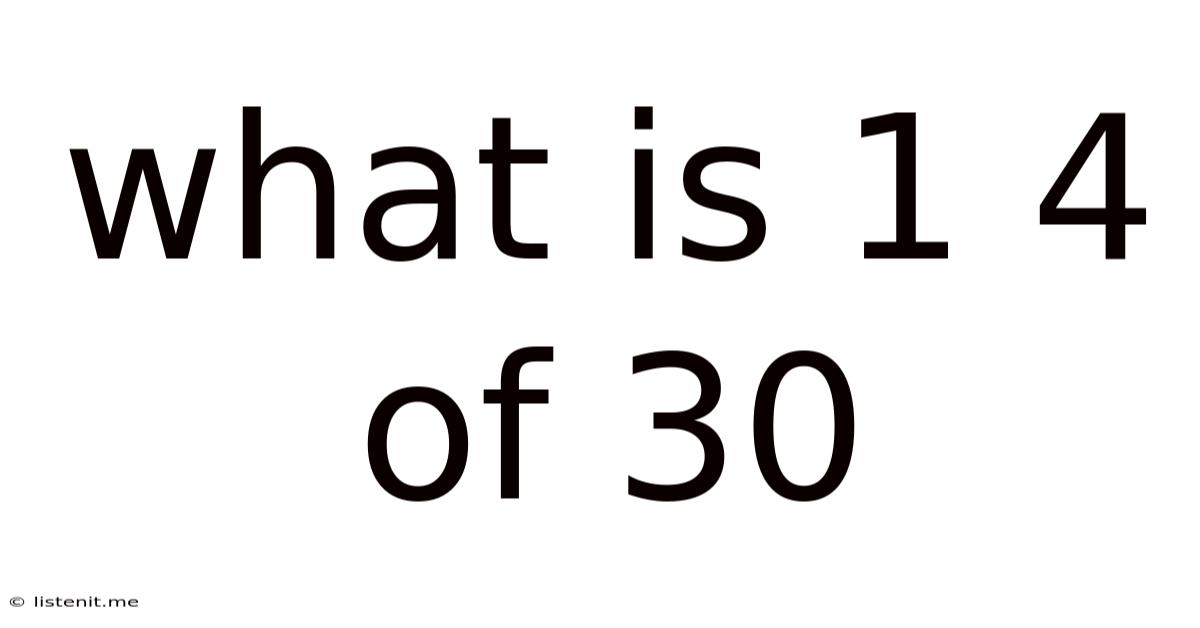
Table of Contents
What is 1/4 of 30? A Deep Dive into Fractions and Their Applications
Understanding fractions is fundamental to mathematics and everyday life. This seemingly simple question, "What is 1/4 of 30?", opens the door to exploring various mathematical concepts and their practical applications. This article will not only answer the question directly but will also delve into the underlying principles, provide multiple methods for solving similar problems, and explore real-world scenarios where fractional calculations are essential.
Understanding the Question: 1/4 of 30
The phrase "1/4 of 30" translates directly into a multiplication problem: (1/4) * 30. This signifies finding one-quarter (or 25%) of the total value 30. The core concept is to divide 30 into four equal parts and then take one of those parts.
Method 1: Direct Multiplication
The most straightforward approach is to perform the multiplication directly:
(1/4) * 30 = 30/4
Now, simplify the fraction by dividing both the numerator (30) and the denominator (4) by their greatest common divisor, which is 2:
30/4 = 15/2
Finally, convert the improper fraction to a mixed number or decimal:
15/2 = 7.5
Therefore, 1/4 of 30 is 7.5.
Method 2: Division First
Alternatively, we can first divide 30 by the denominator (4) and then multiply the result by the numerator (1):
30 ÷ 4 = 7.5
7.5 * 1 = 7.5
This method yields the same result: 1/4 of 30 is 7.5. This approach is particularly helpful when dealing with larger numbers or more complex fractions.
Method 3: Using Percentages
Since 1/4 is equivalent to 25%, we can calculate 25% of 30:
25% of 30 = (25/100) * 30 = 0.25 * 30 = 7.5
This method highlights the relationship between fractions and percentages, demonstrating that they are simply different ways of representing the same proportion.
Real-World Applications of Fractional Calculations
Fractions and their related calculations are ubiquitous in daily life. Here are some examples:
1. Cooking and Baking:
Recipes frequently use fractions. For instance, a recipe might call for 1/2 cup of sugar or 3/4 teaspoon of salt. Understanding fractions is crucial for accurate measurements and successful cooking. Scaling recipes up or down also relies heavily on fractional calculations. If a recipe makes 12 cookies and you only want 6, you need to halve all the ingredients, which involves fractional division.
2. Shopping and Budgeting:
Sales and discounts are often expressed as fractions or percentages. For example, a "1/4 off" sale means you'll pay 75% of the original price. Calculating the final price requires understanding and applying fractional calculations. Similarly, budgeting involves allocating a portion of your income to different expenses. This often involves working with fractions to ensure that all your expenses add up to your total income.
3. Construction and Engineering:
Precision is paramount in construction and engineering. Measurements are often expressed in fractions of inches or centimeters. Calculations involving dimensions, materials, and angles heavily rely on fractional mathematics. Calculating the amount of materials required for a project based on its dimensions necessitates using fractions and decimals efficiently.
4. Time Management:
Time can be divided into fractions. For example, 1/4 of an hour is 15 minutes. Effective time management involves breaking down tasks into smaller, manageable units and allocating specific amounts of time to each, often utilizing fractions of an hour or day.
5. Data Analysis and Statistics:
Fractions and percentages are essential for representing proportions and probabilities in data analysis. Analyzing data sets, interpreting survey results, or understanding probabilities in various contexts frequently requires calculations with fractions and decimals. For instance, calculating the percentage of a population holding a particular view requires understanding fractional representation and conversion.
Expanding the Concept: Working with More Complex Fractions
The principle of finding a fraction of a number remains the same even when dealing with more complex fractions. For example, let's consider finding 3/8 of 40:
(3/8) * 40 = 120/8 = 15
The calculation involves multiplying the numerator (3) by 40 and then dividing by the denominator (8). This can be simplified by canceling common factors before performing the multiplication. In this case, 8 divides into 40 five times, simplifying the calculation to 3 * 5 = 15.
Tackling Fractions with Mixed Numbers
When dealing with mixed numbers, convert them to improper fractions before performing the multiplication. For example, let's find 1 1/2 of 12:
First, convert 1 1/2 to an improper fraction: (1 * 2 + 1)/2 = 3/2
Then, multiply: (3/2) * 12 = 36/2 = 18
Therefore, 1 1/2 of 12 is 18.
Conclusion: Mastering Fractions for a Brighter Future
Understanding fractions is a cornerstone of mathematical literacy. The seemingly simple question, "What is 1/4 of 30?" serves as a gateway to explore the diverse applications of fractions in everyday life, from cooking and budgeting to construction and data analysis. Mastering these calculations empowers individuals to solve real-world problems efficiently and confidently, enhancing their analytical and problem-solving skills. By consistently practicing and applying these concepts, one can build a strong foundation in mathematics and navigate various challenges with ease. The ability to readily perform these calculations is invaluable in various aspects of life and will be consistently needed throughout one's academic and professional careers. So, next time you face a problem involving fractions, remember the fundamental principles and the various approaches to solve them efficiently and accurately. Remember that consistent practice and the application of these skills in diverse situations will strengthen your understanding and improve your confidence in tackling complex mathematical scenarios.
Latest Posts
Latest Posts
-
What Is 17 Percent Of 100
May 24, 2025
-
5am To 12am Is How Many Hours
May 24, 2025
-
What Is 2 1 As A Fraction
May 24, 2025
-
What Is 4 Divided By 1 2
May 24, 2025
-
Cash On Cash Return Calculator Real Estate
May 24, 2025
Related Post
Thank you for visiting our website which covers about What Is 1 4 Of 30 . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.