What Is 1 4 Of 25
listenit
May 24, 2025 · 5 min read
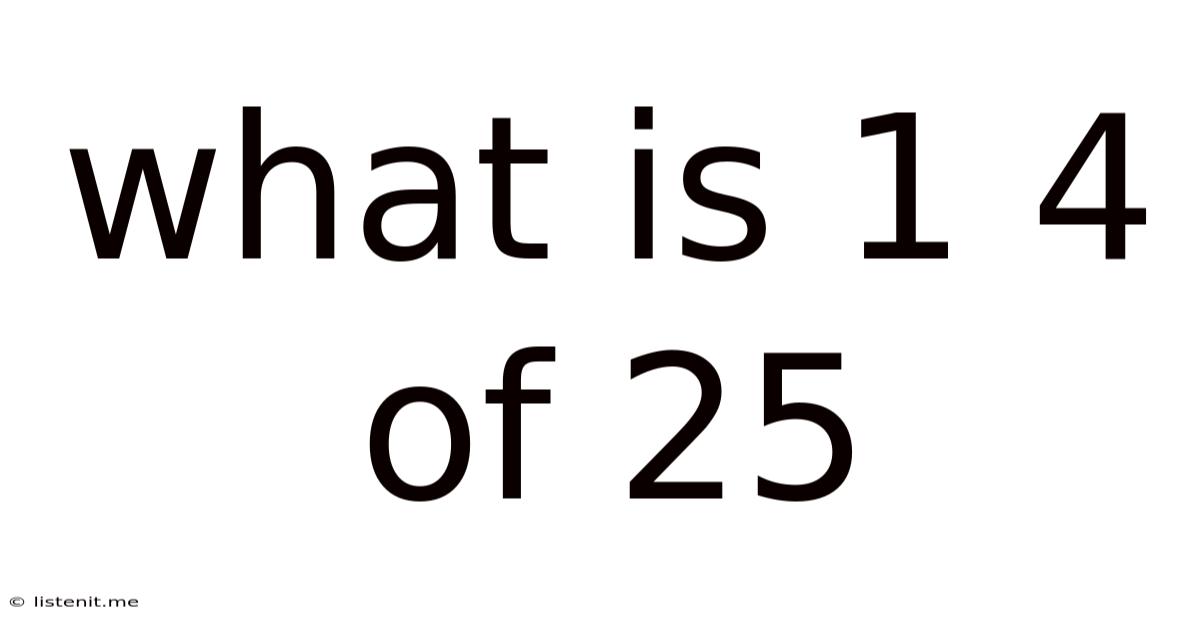
Table of Contents
What is 1/4 of 25? A Deep Dive into Fractions and Their Applications
This seemingly simple question, "What is 1/4 of 25?", opens the door to a fascinating exploration of fractions, their fundamental role in mathematics, and their widespread applications in everyday life. While the answer itself is straightforward, understanding the underlying concepts provides a strong foundation for more complex mathematical operations and problem-solving.
Understanding Fractions: The Building Blocks of Numbers
Before we tackle the specific problem, let's solidify our understanding of fractions. A fraction represents a part of a whole. It's expressed as a ratio of two numbers: the numerator (the top number) and the denominator (the bottom number). The numerator indicates how many parts we have, while the denominator indicates how many equal parts the whole is divided into.
In the fraction 1/4, the numerator is 1 and the denominator is 4. This means we're considering one part out of a total of four equal parts. Visualizing this can be helpful. Imagine a pizza cut into four equal slices. 1/4 of the pizza represents one of those slices.
Calculating 1/4 of 25: Multiple Approaches
There are several ways to calculate 1/4 of 25. Let's explore the most common methods:
Method 1: Direct Multiplication
The most straightforward approach involves multiplying the fraction (1/4) by the whole number (25):
(1/4) * 25 = 25/4
This results in an improper fraction (where the numerator is greater than the denominator). To convert this to a mixed number (a whole number and a fraction), we perform division:
25 ÷ 4 = 6 with a remainder of 1.
Therefore, 25/4 is equal to 6 1/4.
Method 2: Division
Since finding 1/4 of a number is equivalent to dividing the number by 4, we can directly divide 25 by 4:
25 ÷ 4 = 6.25
This gives us the decimal equivalent of 6 1/4.
Method 3: Finding Equivalent Fractions
We can simplify the calculation by finding an equivalent fraction with a denominator that easily divides into 25. While not always applicable, this method highlights the flexibility of fractions. Let's convert 1/4 to an equivalent fraction with a denominator that's a factor of 25:
We know that 25 is divisible by 5, so let's convert the fraction:
(1/4) * (5/5) = 5/20
Now, we can calculate:
(5/20) * 25 = 125/20
Simplifying this improper fraction gives us:
125/20 = 6 5/20 = 6 1/4
This approach demonstrates that manipulating fractions to find equivalent forms can sometimes simplify calculations.
Real-World Applications: Where Fractions Matter
Fractions are not just abstract mathematical concepts; they are fundamental to numerous aspects of everyday life. Understanding them is crucial for:
1. Cooking and Baking: Recipes often call for fractional amounts of ingredients, such as 1/2 cup of flour or 1/4 teaspoon of salt. Accurate measurements are essential for achieving desired results.
2. Finance and Budgeting: Fractions are used extensively in finance. Calculating interest rates, understanding discounts, and managing budgets all involve fractional calculations. For example, if you receive a 25% discount on a $100 item, you're essentially calculating 1/4 of $100.
3. Construction and Engineering: Precision is critical in construction and engineering. Fractions are essential for precise measurements and calculations to ensure structural integrity and functionality. Think of blueprints and architectural plans, where dimensions are often expressed in fractions of an inch or meter.
4. Time Management: We use fractions to express parts of an hour (e.g., 1/4 hour = 15 minutes, 1/2 hour = 30 minutes). Effective time management requires understanding and utilizing these fractional representations.
5. Data Analysis and Statistics: Fractions and their decimal equivalents play a key role in data analysis and statistics. Representing proportions, percentages, and probabilities often involves fractional calculations.
6. Measurement Systems: Both metric and imperial systems use fractions extensively. Understanding how to convert between fractions and decimals is essential for accurate conversions and measurements.
7. Geometry and Spatial Reasoning: Calculating areas, volumes, and other geometric properties often involves fractions and proportions. For instance, finding the area of a triangle might involve multiplying base and height and then dividing by 2.
8. Everyday Shopping: Understanding discounts, sales, and unit pricing often involves calculating fractions. For example, if an item is marked down by 1/3, you need to calculate the actual saving.
Beyond the Basics: Expanding our Understanding
The simple question, "What is 1/4 of 25?", serves as a springboard to explore more complex concepts:
1. Percentage Calculation: 1/4 is equivalent to 25%. Understanding the relationship between fractions and percentages is crucial for various applications, including financial calculations and data analysis. This highlights that the problem can also be framed as "What is 25% of 25?".
2. Decimal Representation: The answer, 6.25, emphasizes the interrelationship between fractions and decimals. Converting between fractions and decimals is a fundamental skill in mathematics.
3. Ratios and Proportions: The problem can also be viewed as a ratio or proportion problem: 1/4 = x/25. Solving for x gives us the same answer.
4. Advanced Fraction Operations: Once comfortable with basic fraction calculations, one can move on to more complex operations, including addition, subtraction, multiplication, and division of fractions.
Conclusion: The Importance of Fractional Understanding
The seemingly simple question, "What is 1/4 of 25?", unveils a rich tapestry of mathematical concepts and their widespread applicability. From basic arithmetic to advanced calculations, fractions are an indispensable tool in various fields. Mastering fractional operations empowers individuals to navigate a variety of challenges in academic, professional, and everyday contexts. Therefore, a strong understanding of fractions is not just a mathematical skill but a vital life skill that enhances problem-solving abilities and opens doors to a broader understanding of the world around us.
Latest Posts
Latest Posts
-
5am To 12am Is How Many Hours
May 24, 2025
-
What Is 2 1 As A Fraction
May 24, 2025
-
What Is 4 Divided By 1 2
May 24, 2025
-
Cash On Cash Return Calculator Real Estate
May 24, 2025
-
If You Was Born In 1968 How Old Are You
May 24, 2025
Related Post
Thank you for visiting our website which covers about What Is 1 4 Of 25 . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.