Is 40 A Prime Or Composite Number
listenit
May 10, 2025 · 5 min read
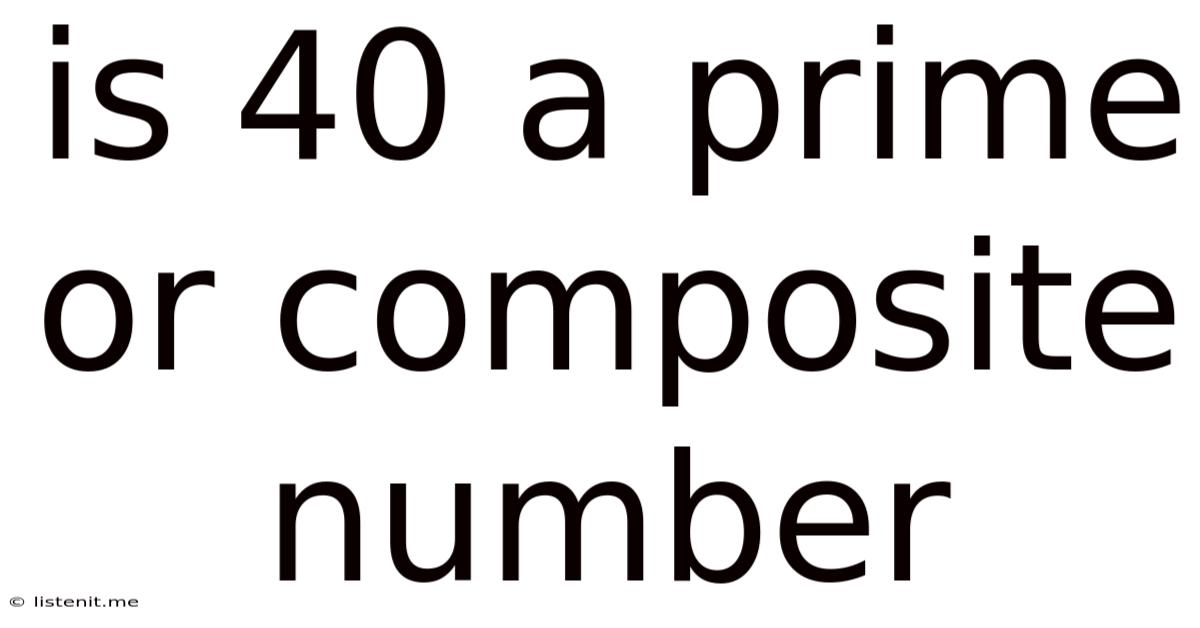
Table of Contents
Is 40 a Prime or Composite Number? A Deep Dive into Number Theory
The question, "Is 40 a prime or composite number?" might seem simple at first glance. However, understanding the answer requires a foundational grasp of number theory, specifically the definitions of prime and composite numbers. This article will not only answer this question definitively but also explore the broader concepts of prime factorization, divisibility rules, and the significance of prime numbers in mathematics.
Understanding Prime and Composite Numbers
Before we delve into whether 40 is prime or composite, let's solidify our understanding of these crucial number classifications.
Prime Numbers: The Building Blocks of Arithmetic
A prime number is a natural number greater than 1 that has only two distinct positive divisors: 1 and itself. This means it's only divisible without a remainder by 1 and itself. Examples include 2, 3, 5, 7, 11, and so on. Prime numbers are the fundamental building blocks of all other numbers, a concept we'll explore further with prime factorization. They are crucial in cryptography, ensuring secure online transactions and data protection. The study of prime numbers, their distribution, and their properties is a central area of mathematical research. The seemingly simple definition hides profound complexities, making prime numbers a fascinating subject for mathematicians and enthusiasts alike.
Composite Numbers: Products of Primes
A composite number, on the other hand, is a natural number greater than 1 that has more than two positive divisors. In simpler terms, it's divisible by at least one number other than 1 and itself. Examples include 4 (divisible by 1, 2, and 4), 6 (divisible by 1, 2, 3, and 6), 9 (divisible by 1, 3, and 9), and so on. Every composite number can be expressed as a unique product of prime numbers – a concept known as the fundamental theorem of arithmetic. This unique representation is a cornerstone of number theory.
The Number 1: Neither Prime Nor Composite
It's important to note that the number 1 is neither prime nor composite. This is a deliberate exclusion based on the definitions. Including 1 would break the fundamental theorem of arithmetic, rendering unique prime factorization impossible.
Determining if 40 is Prime or Composite
Now, let's address the central question: Is 40 a prime or composite number?
The answer is unequivocally: 40 is a composite number.
To understand why, we need only find one divisor of 40 other than 1 and 40. We can easily see that 40 is divisible by 2, 4, 5, 8, 10, and 20. The presence of even one divisor beyond 1 and itself immediately disqualifies it from being a prime number.
Furthermore, we can perform prime factorization of 40:
40 = 2 x 20 = 2 x 2 x 10 = 2 x 2 x 2 x 5 = 2<sup>3</sup> x 5
This demonstrates that 40 is composed of the prime factors 2 and 5. The presence of multiple prime factors further solidifies its composite nature.
Divisibility Rules: A Quick Check for Composite Numbers
Identifying composite numbers can often be simplified using divisibility rules. These rules provide shortcuts to determine if a number is divisible by specific small prime numbers without performing long division. Some key divisibility rules include:
- Divisibility by 2: A number is divisible by 2 if its last digit is even (0, 2, 4, 6, or 8).
- Divisibility by 3: A number is divisible by 3 if the sum of its digits is divisible by 3.
- Divisibility by 5: A number is divisible by 5 if its last digit is 0 or 5.
- Divisibility by 10: A number is divisible by 10 if its last digit is 0.
Applying these rules to 40:
- Divisibility by 2: The last digit of 40 is 0, which is even, so 40 is divisible by 2.
- Divisibility by 5: The last digit of 40 is 0, so 40 is divisible by 5.
- Divisibility by 10: The last digit of 40 is 0, so 40 is divisible by 10.
The fact that 40 satisfies these divisibility rules immediately indicates its composite nature. The existence of these divisors other than 1 and 40 conclusively proves that 40 is not a prime number.
The Significance of Prime Numbers
The importance of prime numbers extends far beyond elementary number theory. They play a crucial role in various fields, including:
Cryptography: Securing Online Transactions
Prime numbers are fundamental to modern cryptography, particularly in public-key cryptography systems like RSA. The security of these systems relies on the computational difficulty of factoring very large numbers into their prime components. The larger the prime numbers used, the more secure the encryption becomes.
Coding Theory: Error Detection and Correction
Prime numbers are utilized in coding theory for error detection and correction in data transmission and storage. They help create efficient codes that can identify and correct errors introduced during transmission.
Hashing Algorithms: Data Integrity
Prime numbers are incorporated into hashing algorithms, which are crucial for ensuring data integrity and security. Hash functions produce a fixed-size output (hash) from any input data. The use of prime numbers within these algorithms helps to minimize collisions and ensure that different inputs generate distinct hashes.
Random Number Generation: Simulation and Modeling
Prime numbers contribute to efficient and robust methods for generating pseudo-random numbers. These numbers are widely used in computer simulations, modeling, and statistical analysis.
Conclusion: 40 is Definitely Composite
In conclusion, the question "Is 40 a prime or composite number?" is easily answered: 40 is a composite number. This determination can be made by simply identifying divisors beyond 1 and 40, performing prime factorization, or applying divisibility rules. Understanding the distinction between prime and composite numbers is crucial not only in basic mathematics but also in various advanced fields where these concepts underpin significant technological advancements and theoretical breakthroughs. The seemingly simple number 40, therefore, serves as a valuable example illustrating the fundamental principles of number theory and its wider implications.
Latest Posts
Latest Posts
-
How Do You Know If A Chemical Equation Is Balanced
May 10, 2025
-
Chi Square Test For Homogeneity Vs Independence
May 10, 2025
-
Draw All Stereoisomers Of 1 2 Dichlorocyclohexane
May 10, 2025
-
Erica And Anna Started Biking In Opposite Directions
May 10, 2025
-
Which Is A Graph For The Inequality X 2
May 10, 2025
Related Post
Thank you for visiting our website which covers about Is 40 A Prime Or Composite Number . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.