How To Subtract Negative And Positive Fractions
listenit
Mar 31, 2025 · 6 min read
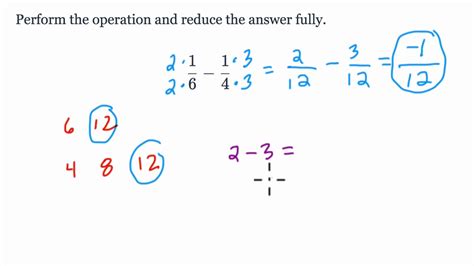
Table of Contents
How to Subtract Negative and Positive Fractions: A Comprehensive Guide
Subtracting fractions, especially those involving negative numbers, can seem daunting. However, with a systematic approach and a solid understanding of the underlying principles, it becomes a manageable and even enjoyable mathematical exercise. This comprehensive guide will walk you through the process step-by-step, covering various scenarios and providing ample examples to solidify your understanding. We'll explore the rules of subtraction with positive and negative fractions, address common pitfalls, and offer tips for mastering this essential skill.
Understanding the Fundamentals: Positive and Negative Fractions
Before diving into subtraction, let's refresh our understanding of positive and negative fractions. A fraction represents a part of a whole, expressed as a numerator (the top number) divided by a denominator (the bottom number). A positive fraction indicates a portion above zero on the number line, while a negative fraction indicates a portion below zero.
For example:
- 1/2 represents one-half and is positive.
- -1/2 represents negative one-half and is located to the left of zero on the number line.
The sign (positive or negative) precedes the entire fraction, affecting its position relative to zero. This sign is crucial when performing subtraction.
Subtracting Fractions with the Same Denominator
When subtracting fractions with the same denominator (the bottom number), the process is straightforward:
- Subtract the numerators: Simply subtract the second numerator from the first numerator.
- Keep the denominator the same: The denominator remains unchanged.
- Determine the sign: The sign of the result depends on the relative magnitudes of the numerators. If the first numerator is larger than the second, the result is positive. If the second numerator is larger, the result is negative.
Examples:
-
1/4 - 3/4 = -2/4 = -1/2 (The numerator 3 is larger than 1, resulting in a negative fraction. We then simplify the fraction.)
-
5/8 - 2/8 = 3/8 (The numerator 5 is larger than 2, resulting in a positive fraction.)
-
-3/5 - 1/5 = -4/5 (Subtracting a positive fraction from a negative one makes the negative fraction even more negative)
Subtracting Fractions with Different Denominators
Subtracting fractions with different denominators requires an extra step – finding a common denominator. This is the least common multiple (LCM) of the two denominators.
- Find the least common denominator (LCD): The LCD is the smallest number that both denominators divide into evenly. You can find this through prime factorization or by listing multiples.
- Convert fractions to equivalent fractions with the LCD: Multiply the numerator and denominator of each fraction by the appropriate factor to achieve the LCD.
- Subtract the numerators: Subtract the numerators of the equivalent fractions.
- Keep the denominator the same (the LCD): The denominator remains the LCD.
- Simplify the result: Reduce the fraction to its simplest form if possible.
Examples:
-
1/3 - 1/2:
- The LCD of 3 and 2 is 6.
- Convert fractions: (1/3) * (2/2) = 2/6 and (1/2) * (3/3) = 3/6
- Subtract numerators: 2/6 - 3/6 = -1/6
-
-2/5 - 3/10:
- The LCD of 5 and 10 is 10.
- Convert fractions: (-2/5) * (2/2) = -4/10
- Subtract numerators: -4/10 - 3/10 = -7/10
-
5/6 - (-1/4):
- The LCD of 6 and 4 is 12.
- Convert fractions: (5/6) * (2/2) = 10/12 and (-1/4) * (3/3) = -3/12
- Subtract numerators: 10/12 - (-3/12) = 13/12 (Note: subtracting a negative is the same as adding a positive)
Subtracting Mixed Numbers
Mixed numbers contain a whole number and a fraction (e.g., 2 1/3). To subtract mixed numbers:
- Convert mixed numbers to improper fractions: Multiply the whole number by the denominator and add the numerator. Keep the same denominator.
- Find the LCD (if necessary): If the denominators are different, find the least common denominator.
- Convert to equivalent fractions with the LCD: Convert the improper fractions to equivalent fractions with the LCD.
- Subtract the numerators: Subtract the numerators of the equivalent fractions.
- Keep the denominator the same (the LCD): The denominator remains the LCD.
- Simplify and convert back to a mixed number (if desired): Simplify the resulting fraction and convert it back to a mixed number by dividing the numerator by the denominator.
Examples:
-
2 1/2 - 1 1/4:
- Convert to improper fractions: 5/2 - 5/4
- The LCD is 4.
- Convert to equivalent fractions: 10/4 - 5/4 = 5/4
- Convert back to mixed number: 1 1/4
-
3 2/3 - (-1 1/6):
- Convert to improper fractions: 11/3 - (-7/6)
- The LCD is 6.
- Convert to equivalent fractions: 22/6 - (-7/6) = 29/6
- Convert back to mixed number: 4 5/6
Common Mistakes to Avoid
- Incorrectly finding the LCD: Carefully determine the least common denominator. Using an incorrect LCD will lead to an inaccurate result.
- Forgetting to change the signs when subtracting negative fractions: Subtracting a negative number is equivalent to adding a positive number.
- Errors in simplifying fractions: Always reduce fractions to their simplest form by dividing the numerator and denominator by their greatest common divisor (GCD).
- Miscalculating when converting between mixed numbers and improper fractions: Double-check your calculations during the conversion process to prevent errors in your final answer.
Mastering Fraction Subtraction: Practice and Resources
Consistent practice is key to mastering fraction subtraction. Start with simple problems and gradually increase the complexity. Use online resources, textbooks, and workbooks to find additional practice problems. You can also create your own problems using different combinations of positive and negative fractions and mixed numbers. Remember that understanding the underlying principles is crucial; rote memorization alone isn't sufficient. Focus on grasping the "why" behind each step rather than just the "how."
Real-World Applications of Fraction Subtraction
Fraction subtraction isn't just an abstract mathematical concept; it has many practical applications in everyday life. For example:
- Cooking and Baking: Adjusting recipes often involves subtracting fractions of ingredients.
- Construction and Engineering: Precise measurements in construction and engineering rely heavily on fractional calculations.
- Finance and Budgeting: Managing budgets and calculating expenses often involves working with fractions and decimals.
- Science: Many scientific measurements and experiments involve fractions and require subtraction to analyze data.
By developing a strong understanding of fraction subtraction, you'll be better equipped to handle these and other real-world challenges.
Conclusion
Subtracting negative and positive fractions might initially seem challenging, but with a clear understanding of the rules and consistent practice, it becomes a readily manageable skill. Remember to focus on finding the common denominator, carefully handle the signs, and always simplify your final answer. By mastering this essential mathematical concept, you open up a wide range of opportunities for solving problems in various fields, both academic and practical. So, grab your pencil and paper, and start practicing! You'll be surprised at how quickly your skills improve.
Latest Posts
Latest Posts
-
Is Soil An Abiotic Or Biotic Factor
Apr 02, 2025
-
Two Or More Atoms Chemically Combined
Apr 02, 2025
-
What Is The Percentage Of 7
Apr 02, 2025
-
All Atoms Of The Same Element Have
Apr 02, 2025
-
Animals That Feed Exclusively On Plants Are Called
Apr 02, 2025
Related Post
Thank you for visiting our website which covers about How To Subtract Negative And Positive Fractions . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.
