How To Find Inverse Of An Exponential Function
listenit
Mar 29, 2025 · 5 min read
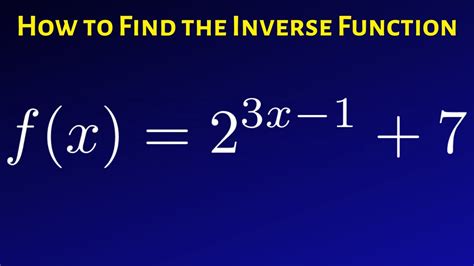
Table of Contents
How to Find the Inverse of an Exponential Function
Finding the inverse of an exponential function is a crucial skill in mathematics, with applications spanning various fields like calculus, statistics, and engineering. This comprehensive guide will walk you through the process, explaining the concepts clearly and providing numerous examples to solidify your understanding. We'll cover both simple and more complex cases, ensuring you're equipped to handle a wide range of exponential functions.
Understanding Exponential Functions and Their Inverses
Before diving into the mechanics of finding inverses, let's establish a firm understanding of what exponential functions are and why their inverses are important.
An exponential function is a function of the form:
f(x) = a<sup>x</sup>
where 'a' is a positive constant (base), and 'x' is the exponent (independent variable). The base 'a' cannot be 1, as 1 raised to any power is always 1, resulting in a constant function.
The inverse of a function essentially "undoes" the original function. If we have a function f(x) and its inverse f<sup>-1</sup>(x), then applying f(x) and then f<sup>-1</sup>(x) (or vice versa) will return the original input. Graphically, the inverse of a function is its reflection across the line y = x.
The inverse of an exponential function is a logarithmic function. Logarithmic functions are crucial for solving equations involving exponents and for analyzing data where exponential growth or decay is observed.
Finding the Inverse: A Step-by-Step Guide
The process of finding the inverse of an exponential function involves several key steps:
-
Replace f(x) with y: This helps simplify the notation and makes the process more manageable.
-
Swap x and y: This step reflects the function across the line y = x, which is the geometric representation of finding the inverse.
-
Solve for y: This involves using logarithmic properties to isolate 'y'. This is the most crucial step and often requires careful algebraic manipulation.
-
Replace y with f<sup>-1</sup>(x): This final step denotes the inverse function.
Examples: From Simple to Complex
Let's work through several examples, starting with straightforward cases and gradually increasing the complexity.
Example 1: A Simple Exponential Function
Find the inverse of f(x) = 2<sup>x</sup>
-
Replace f(x) with y: y = 2<sup>x</sup>
-
Swap x and y: x = 2<sup>y</sup>
-
Solve for y: To isolate 'y', we use the logarithm base 2. Applying the logarithm base 2 to both sides, we get:
log<sub>2</sub>(x) = log<sub>2</sub>(2<sup>y</sup>)
Since log<sub>b</sub>(b<sup>x</sup>) = x, this simplifies to:
log<sub>2</sub>(x) = y
-
Replace y with f<sup>-1</sup>(x): f<sup>-1</sup>(x) = log<sub>2</sub>(x)
Therefore, the inverse of f(x) = 2<sup>x</sup> is f<sup>-1</sup>(x) = log<sub>2</sub>(x).
Example 2: Including a Constant Term
Find the inverse of f(x) = e<sup>x</sup> + 3
-
Replace f(x) with y: y = e<sup>x</sup> + 3
-
Swap x and y: x = e<sup>y</sup> + 3
-
Solve for y: First, subtract 3 from both sides:
x - 3 = e<sup>y</sup>
Then, take the natural logarithm (ln) of both sides:
ln(x - 3) = ln(e<sup>y</sup>)
This simplifies to:
ln(x - 3) = y
-
Replace y with f<sup>-1</sup>(x): f<sup>-1</sup>(x) = ln(x - 3)
The inverse of f(x) = e<sup>x</sup> + 3 is f<sup>-1</sup>(x) = ln(x - 3). Note the restriction on the domain of the inverse function: x must be greater than 3, as the natural logarithm is only defined for positive arguments.
Example 3: A More Complex Exponential Function
Find the inverse of f(x) = 3<sup>2x-1</sup> + 5
-
Replace f(x) with y: y = 3<sup>2x-1</sup> + 5
-
Swap x and y: x = 3<sup>2y-1</sup> + 5
-
Solve for y: Subtract 5 from both sides:
x - 5 = 3<sup>2y-1</sup>
Take the logarithm base 3 of both sides:
log<sub>3</sub>(x - 5) = 2y - 1
Add 1 to both sides and divide by 2:
(log<sub>3</sub>(x - 5) + 1) / 2 = y
-
Replace y with f<sup>-1</sup>(x): f<sup>-1</sup>(x) = (log<sub>3</sub>(x - 5) + 1) / 2
This illustrates that finding the inverse of even more complex exponential functions is possible with careful application of logarithmic properties and algebraic manipulation.
Handling Different Bases
The examples above highlight the importance of understanding logarithmic properties. Remember that:
-
log<sub>a</sub>(b) = c is equivalent to a<sup>c</sup> = b
-
log<sub>a</sub>(x<sup>n</sup>) = n * log<sub>a</sub>(x)
-
log<sub>a</sub>(x) = log<sub>b</sub>(x) / log<sub>b</sub>(a) (Change of base formula – useful for calculating logarithms with bases not readily available on calculators)
Practical Applications and Importance
The ability to find the inverse of exponential functions is fundamental in many areas:
-
Solving Exponential Equations: Inverse functions are essential for isolating the variable in exponential equations.
-
Modeling Exponential Growth and Decay: In fields like biology, finance, and physics, exponential functions model various phenomena. Their inverses allow us to determine the time it takes to reach a specific value.
-
Data Analysis: Analyzing data exhibiting exponential trends often requires using logarithmic transformations to linearize the data, simplifying analysis.
-
Calculus: Finding derivatives and integrals of exponential functions often involves manipulating their inverses.
Conclusion
Finding the inverse of an exponential function is a valuable mathematical skill. By mastering the steps outlined above and practicing with a variety of examples, you can confidently tackle more complex exponential functions and utilize this skill in various practical applications. Remember to always check for domain restrictions in your inverse functions. Consistent practice is key to building a strong understanding and proficiency in this area.
Latest Posts
Latest Posts
-
What Do Coefficients Represent In A Chemical Equation
Mar 31, 2025
-
Why Do Chemical Equations Have To Be Balanced
Mar 31, 2025
-
What Percent Is A 26 Out Of 30
Mar 31, 2025
-
Which Is Denser Oceanic Or Continental Crust
Mar 31, 2025
-
Root 3 Divided By Root 3
Mar 31, 2025
Related Post
Thank you for visiting our website which covers about How To Find Inverse Of An Exponential Function . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.
