How To Find Concentration From Dilution
listenit
Apr 02, 2025 · 5 min read
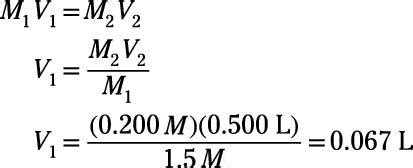
Table of Contents
- How To Find Concentration From Dilution
- Table of Contents
- How to Find Concentration from Dilution: A Comprehensive Guide
- Understanding Dilution
- Calculating Concentration After Dilution: Step-by-Step Guide
- Different Units and Conversions
- Dealing with More Complex Scenarios
- Serial Dilutions
- Dilutions involving different solutes
- Dilutions with density considerations
- Practical Applications of Dilution Calculations
- Troubleshooting Common Mistakes
- Advanced Topics in Dilution Calculations
- Conclusion
- Latest Posts
- Latest Posts
- Related Post
How to Find Concentration from Dilution: A Comprehensive Guide
Finding the concentration of a diluted solution is a fundamental concept in chemistry and many other scientific fields. Whether you're working in a laboratory, analyzing environmental samples, or simply tackling a chemistry problem, understanding how to perform these calculations is crucial. This comprehensive guide will walk you through various methods, providing clear explanations and practical examples to help you master this essential skill.
Understanding Dilution
Dilution is the process of decreasing the concentration of a solute in a solution by adding more solvent. The amount of solute remains constant; only the volume of the solution changes. This principle is governed by the fundamental equation:
M1V1 = M2V2
Where:
- M1 represents the initial concentration (molarity) of the solution.
- V1 represents the initial volume of the solution.
- M2 represents the final concentration (molarity) of the diluted solution.
- V2 represents the final volume of the diluted solution.
This equation is based on the principle of conservation of mass – the amount of solute remains constant throughout the dilution process.
Calculating Concentration After Dilution: Step-by-Step Guide
Let's break down the process of calculating the concentration of a diluted solution using the M1V1 = M2V2 equation. We'll illustrate with a detailed example:
Problem: You have 50 mL of a 2.0 M solution of sodium chloride (NaCl). You dilute this solution to a final volume of 250 mL. What is the concentration of the diluted NaCl solution?
Step 1: Identify the known variables.
- M1 (Initial concentration): 2.0 M
- V1 (Initial volume): 50 mL
- V2 (Final volume): 250 mL
- M2 (Final concentration): This is what we need to find.
Step 2: Apply the dilution equation (M1V1 = M2V2).
Substitute the known values into the equation:
(2.0 M)(50 mL) = M2(250 mL)
Step 3: Solve for the unknown variable (M2).
To isolate M2, divide both sides of the equation by 250 mL:
M2 = [(2.0 M)(50 mL)] / 250 mL
Step 4: Calculate the final concentration.
M2 = 0.4 M
Therefore, the concentration of the diluted NaCl solution is 0.4 M.
Different Units and Conversions
The M1V1 = M2V2 equation works seamlessly with various units of concentration and volume, provided you maintain consistency throughout the calculation. However, you might encounter situations where unit conversion is necessary.
Example using different units:
You have 100 cm³ of a 0.5 mol/dm³ solution. You add 150 cm³ of solvent. What is the new concentration?
Step 1: Understand the Units
Notice that we have cubic centimeters (cm³) and cubic decimeters (dm³). We need to convert one to match the other. Remember, 1 dm³ = 1000 cm³.
Step 2: Convert Units
It's often easier to convert to the smaller unit, so we'll convert dm³ to cm³:
0.5 mol/dm³ * (1000 cm³/1 dm³) = 500 mol/cm³
Step 3: Apply M1V1 = M2V2
Now we can use the equation:
(500 mol/cm³)(100 cm³) = M2(250 cm³)
Step 4: Solve for M2
M2 = 200 mol/cm³
Step 5: Convert Back (if necessary)
If you need the final answer in mol/dm³, convert back:
200 mol/cm³ * (1 dm³/1000 cm³) = 0.2 mol/dm³
Dealing with More Complex Scenarios
While the M1V1 = M2V2 equation is versatile, some situations require a more nuanced approach. Let's explore a few:
Serial Dilutions
Serial dilutions involve a series of dilutions, where a portion of the diluted solution is further diluted. This is frequently used to create solutions with very low concentrations. You simply apply the M1V1 = M2V2 equation iteratively for each dilution step.
Dilutions involving different solutes
When multiple solutes are present, the dilution equation applies to each solute independently. You need to calculate the final concentration of each solute separately.
Dilutions with density considerations
In some cases, particularly with concentrated solutions, density changes significantly upon dilution. In such cases, the simple M1V1 = M2V2 equation might not be accurate. More sophisticated calculations involving mass and volume changes may be necessary.
Practical Applications of Dilution Calculations
The ability to calculate dilutions is essential in various fields:
- Analytical Chemistry: Preparing standard solutions for calibrating instruments and conducting quantitative analyses.
- Biochemistry: Diluting enzymes, proteins, and other biological samples for experiments.
- Pharmacology: Preparing solutions for drug delivery and testing.
- Environmental Science: Diluting samples for analysis of pollutants and other substances.
- Food Science: Preparing solutions for food processing and quality control.
Troubleshooting Common Mistakes
- Unit Inconsistencies: Ensure all units are consistent before applying the M1V1 = M2V2 equation.
- Incorrect Variable Identification: Double-check you've correctly identified the initial and final concentrations and volumes.
- Mathematical Errors: Carefully review your calculations to avoid simple arithmetic mistakes.
- Neglecting Serial Dilutions: When performing serial dilutions, remember to apply the M1V1 = M2V2 equation for each step.
Advanced Topics in Dilution Calculations
While the M1V1 = M2V2 equation provides a solid foundation, advanced dilution calculations might involve more complex factors:
- Non-ideal solutions: For solutions that deviate significantly from ideal behavior, the M1V1 = M2V2 equation might not be accurate. More sophisticated thermodynamic models are needed in such cases.
- Temperature effects: Temperature changes can affect the volume and concentration of solutions. Corrections may be necessary for precise calculations at different temperatures.
- Activity coefficients: For concentrated solutions, activity coefficients should be considered to account for the non-ideal interactions between solute particles.
Conclusion
Mastering dilution calculations is a fundamental skill for anyone working with solutions. Understanding the M1V1 = M2V2 equation, along with the ability to handle unit conversions and address more complex scenarios, is crucial for accurate and reliable results in various scientific and practical applications. By practicing the steps outlined in this guide and understanding the underlying principles, you'll confidently tackle dilution problems and contribute to the success of your endeavors. Remember to always double-check your work and consider the limitations of the simple dilution equation when dealing with non-ideal or complex systems.
Latest Posts
Latest Posts
-
Where Are The Most Asteroids Located
Apr 05, 2025
-
Graph Of Y 4x X 2
Apr 05, 2025
-
How Many 1 3 In 1 2
Apr 05, 2025
-
What Is 8 Percent Of 4000
Apr 05, 2025
-
Is Milk Turning Sour A Chemical Change
Apr 05, 2025
Related Post
Thank you for visiting our website which covers about How To Find Concentration From Dilution . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.
