C 5 F 32 9 Solve For F
listenit
Mar 30, 2025 · 5 min read
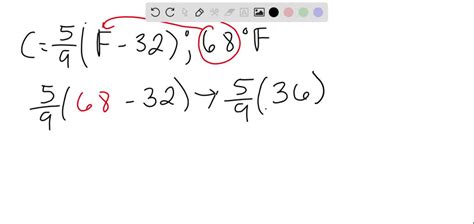
Table of Contents
Decoding the Celsius-Fahrenheit Conversion: Solving for F in C = 5/9(F - 32)
The equation C = 5/9(F - 32) is a fundamental formula in physics and everyday life, representing the conversion between Celsius (°C) and Fahrenheit (°F) temperature scales. Understanding how to manipulate this equation, particularly solving for F, is crucial for anyone working with temperature measurements or simply wanting a deeper understanding of mathematical problem-solving. This comprehensive guide will walk you through the process step-by-step, exploring different approaches and highlighting key concepts along the way.
Understanding the Equation: A Foundation for Solving
Before diving into the solution, let's dissect the equation itself: C = 5/9(F - 32). This equation tells us that the Celsius temperature (C) is calculated by taking the Fahrenheit temperature (F), subtracting 32, and then multiplying the result by 5/9. This fractional multiplier accounts for the different scales and intervals between the freezing and boiling points of water in each system.
The challenge, and the focus of this article, is to rearrange this equation to solve for F, meaning to express F in terms of C. This allows us to directly calculate the Fahrenheit equivalent given a Celsius value.
Method 1: Algebraic Manipulation - The Standard Approach
This method uses standard algebraic techniques to isolate F on one side of the equation. It's the most common and straightforward approach.
-
Eliminate the Fraction: The fraction 5/9 is multiplying the parenthesis (F - 32). To remove it, we multiply both sides of the equation by its reciprocal, which is 9/5:
(9/5)C = (9/5) * (5/9)(F - 32)
This simplifies to:
(9/5)C = F - 32
-
Isolate the Variable: Now, we need to isolate F. Add 32 to both sides of the equation:
(9/5)C + 32 = F
-
Final Solution: We've successfully solved for F! The equation for converting Celsius to Fahrenheit is:
F = (9/5)C + 32
This is the standard formula for converting Celsius to Fahrenheit. Let's illustrate this with an example.
Example: Convert 20°C to Fahrenheit.
Substitute C = 20 into the equation:
F = (9/5)(20) + 32 = 36 + 32 = 68°F
Therefore, 20°C is equivalent to 68°F.
Method 2: Working Backwards from a Known Conversion
This method leverages a known conversion point to derive the formula. We know that 0°C is equivalent to 32°F. Let's use this knowledge to work backward.
-
Start with the Original Equation: C = 5/9(F - 32)
-
Substitute a Known Value: Let's plug in C = 0:
0 = 5/9(F - 32)
-
Solve for F: Multiply both sides by 9/5:
0 = F - 32
Add 32 to both sides:
F = 32
This confirms that 0°C equals 32°F, as expected. However, this method doesn't directly provide the general formula. It demonstrates a point of verification but lacks the generality of the algebraic manipulation.
Method 3: Using Dimensional Analysis (A More Advanced Approach)
Dimensional analysis is a powerful technique used in physics and engineering. It helps ensure the correctness of equations by checking the units. While it doesn't directly solve for F, it provides a valuable perspective on the equation's structure.
The equation C = 5/9(F - 32) can be viewed dimensionally as follows:
- C has units of degrees Celsius (°C).
- F has units of degrees Fahrenheit (°F).
- The term (F - 32) also has units of °F.
- The fraction 5/9 is dimensionless (it's a ratio).
The equation suggests that we're transforming a quantity in °F to a quantity in °C. The dimensionless fraction 5/9 acts as a scaling factor between the two scales.
While dimensional analysis doesn't directly solve the equation, it reinforces that the units are consistent and that the formula correctly translates between the two temperature scales.
Practical Applications and Importance
The ability to convert between Celsius and Fahrenheit is vital in many fields:
- Science and Engineering: Many scientific experiments and engineering designs involve temperature measurements, requiring accurate conversions between the two scales.
- Meteorology: Weather reports often use both Celsius and Fahrenheit, making conversion essential for understanding weather patterns.
- Cooking: Recipes might specify temperatures in either Celsius or Fahrenheit, making conversion a necessary skill for cooks.
- Medicine: Body temperature is often measured in both Celsius and Fahrenheit, requiring accurate conversion for medical diagnosis.
Common Mistakes to Avoid
When solving for F, or using the formula generally, some common mistakes to avoid include:
- Incorrect Order of Operations: Remember the order of operations (PEMDAS/BODMAS): Parentheses/Brackets, Exponents/Orders, Multiplication and Division (from left to right), Addition and Subtraction (from left to right).
- Fractional Errors: Be cautious when working with fractions. Ensure correct multiplication and division, particularly when multiplying or dividing by the reciprocal.
- Unit Errors: Always be mindful of the units. Make sure you're working with Celsius when using C and Fahrenheit when using F.
Beyond the Basics: Extending Your Understanding
Beyond simply solving for F, there are other ways to deepen your understanding of this equation:
- Graphing the Equation: Plotting the equation C = 5/9(F - 32) on a graph can visually represent the linear relationship between Celsius and Fahrenheit. The slope of the line reflects the conversion factor (5/9).
- Exploring the Intersection Point: There's a single temperature at which the Celsius and Fahrenheit scales are numerically equal. Finding this intersection point involves setting C = F in the equation and solving for the temperature.
- Developing Intuition: With practice, you'll develop an intuitive sense for approximate conversions. For instance, you'll quickly recognize that a temperature around 20°C is roughly equivalent to a temperature in the low 70s°F.
Conclusion: Mastering Temperature Conversions
The ability to solve for F in the equation C = 5/9(F - 32) is more than just a mathematical exercise. It's a practical skill with widespread applications across various disciplines. By understanding the algebraic manipulation, utilizing alternative methods (such as the dimensional analysis), and avoiding common pitfalls, you'll gain a firm grasp of this fundamental formula and confidently navigate temperature conversions in any situation. This comprehensive guide aimed to provide a deep understanding of the process, allowing you to not only solve the equation but also appreciate the underlying principles and practical implications of temperature scale conversion.
Latest Posts
Latest Posts
-
Solve This Equation 4y 228 352
Apr 01, 2025
-
Oxidation Reactions Are Coupled With Reactions
Apr 01, 2025
-
Whats Half Of 1 And 1 2
Apr 01, 2025
-
Why Is Water Liquid At Room Temperature
Apr 01, 2025
-
How To Determine The Density Of A Solid
Apr 01, 2025
Related Post
Thank you for visiting our website which covers about C 5 F 32 9 Solve For F . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.
