60 Of What Number Is 20
listenit
Mar 27, 2025 · 4 min read
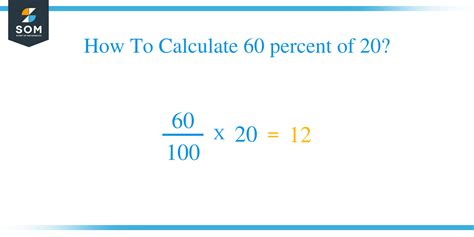
Table of Contents
60% of What Number is 20? Understanding Percentages and Solving Equations
Finding the answer to "60% of what number is 20?" involves understanding percentages and translating the problem into a solvable equation. This seemingly simple question opens the door to a broader understanding of percentage calculations, their applications in everyday life, and even more complex mathematical concepts. This article will thoroughly explore the solution, provide multiple approaches, and delve into the practical applications of percentage problems.
Understanding Percentages
Percentages are a way of expressing a number as a fraction of 100. The symbol "%" signifies "per cent," or "out of 100." Therefore, 60% can be written as 60/100 or 0.6 in decimal form. This fundamental understanding is crucial for solving percentage problems.
Translating the Problem into an Equation
The statement "60% of what number is 20?" can be translated into an algebraic equation. Let's represent the unknown number as 'x'. The equation becomes:
0.6 * x = 20
This equation states that 60% (or 0.6) multiplied by an unknown number (x) equals 20. Solving for 'x' will give us the answer.
Method 1: Solving the Equation Directly
The simplest method is to solve the equation directly using algebraic manipulation:
-
Divide both sides by 0.6: This isolates 'x' on one side of the equation. x = 20 / 0.6
-
Perform the division: x = 33.333...
Therefore, 60% of 33.333... is approximately 20. The recurring decimal indicates that the answer is not a whole number.
Method 2: Using Fractions
Instead of using decimals, we can use fractions. Remember that 60% is equal to 60/100, which simplifies to 3/5. The equation becomes:
(3/5) * x = 20
-
Multiply both sides by 5: This eliminates the fraction from the left side. 3x = 100
-
Divide both sides by 3: This isolates 'x'. x = 100/3
-
Simplify the fraction: x = 33.333...
This method yields the same result as the decimal method, confirming our answer.
Method 3: Cross-Multiplication
This method is particularly useful when dealing with more complex percentage problems. We can rewrite the equation as a proportion:
60/100 = 20/x
Now, we can cross-multiply:
60x = 2000
Then, divide both sides by 60:
x = 2000/60 = 100/3 = 33.333...
Again, we arrive at the same solution.
Understanding the Answer and its Implications
The answer, 33.333..., means that 60% of approximately 33.33 is 20. This demonstrates the inverse relationship between percentages and the whole number. If we know a percentage and its corresponding part, we can determine the original whole.
Real-World Applications of Percentage Calculations
Percentage calculations are ubiquitous in daily life. Here are some examples:
-
Sales and Discounts: Retail stores frequently advertise discounts as percentages (e.g., "20% off"). Understanding percentages allows consumers to calculate the actual savings.
-
Taxes: Sales tax, income tax, and other taxes are often expressed as percentages. Calculating the tax amount on a purchase requires percentage knowledge.
-
Finance: Interest rates on loans and savings accounts are expressed as percentages. Understanding these percentages is crucial for making informed financial decisions.
-
Statistics and Data Analysis: Percentages are essential for interpreting data and presenting findings in a clear and concise manner. Charts and graphs frequently use percentages to represent proportions and trends.
-
Science and Engineering: Many scientific and engineering calculations involve percentages, such as calculating efficiency, error margins, or concentrations.
Expanding on Percentage Problems: More Complex Scenarios
While the initial problem was relatively straightforward, percentage problems can become more complex. Consider these variations:
-
Finding the Percentage: Instead of finding the whole number, we might need to find the percentage one number represents of another. For example: "What percentage of 50 is 10?"
-
Finding the Part: Determining a specific part of a whole, given a percentage. For example: "What is 25% of 80?"
-
Multiple Percentage Changes: Problems involving successive percentage increases or decreases, such as compound interest calculations, require a deeper understanding of percentage manipulation.
Solving More Complex Percentage Problems
Let's explore a more challenging scenario:
Problem: A shirt is originally priced at $50. It's discounted by 20%, then an additional 10% is taken off the discounted price. What is the final price?
Solution:
-
First Discount: 20% of $50 is (0.20 * $50) = $10. The price after the first discount is $50 - $10 = $40.
-
Second Discount: 10% of $40 is (0.10 * $40) = $4. The price after the second discount is $40 - $4 = $36.
Therefore, the final price of the shirt is $36. This problem demonstrates the importance of understanding the order of operations and the impact of successive percentage changes.
Conclusion: Mastering Percentages for Everyday Success
Understanding percentages is a fundamental skill with widespread applications. From everyday shopping to complex financial calculations, the ability to solve percentage problems effectively is crucial for informed decision-making. The seemingly simple question "60% of what number is 20?" serves as a gateway to mastering this vital mathematical concept and its numerous practical applications. By understanding the various methods of solving percentage problems and exploring more complex scenarios, individuals can enhance their mathematical proficiency and navigate the quantitative aspects of daily life with greater confidence and accuracy. The more you practice, the more intuitive and effortless these calculations will become, empowering you to handle percentages with ease and precision.
Latest Posts
Latest Posts
-
How Does An Igneous Rock Become A Sedimentary Rock
Mar 30, 2025
-
The Mass Of One Mole Of Carbon Dioxide Is
Mar 30, 2025
-
20 Is 85 Of What Number
Mar 30, 2025
-
Protons Neutrons And Electrons Of Bromine
Mar 30, 2025
-
The Half Life Of Carbon 14 Is 5730 Years
Mar 30, 2025
Related Post
Thank you for visiting our website which covers about 60 Of What Number Is 20 . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.
