6 Divided By 1 2 As A Fraction
listenit
May 25, 2025 · 4 min read
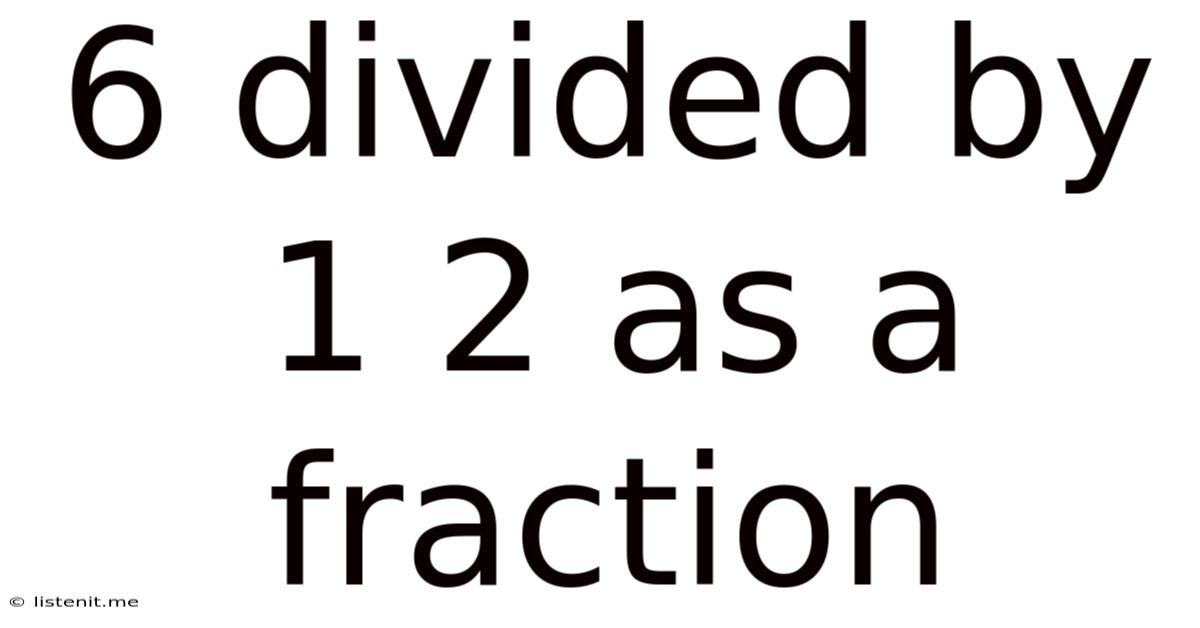
Table of Contents
6 Divided by 1 2 as a Fraction: A Comprehensive Guide
Understanding fractions and division is fundamental to mathematics. This in-depth guide explores the process of dividing 6 by the mixed number 1 2/3, explaining the steps clearly and providing practical examples to solidify your understanding. We’ll delve into the concept of converting mixed numbers into improper fractions, performing the division, and simplifying the resulting fraction to its lowest terms. We'll also explore related concepts and potential applications.
Understanding the Problem: 6 ÷ 1 ⅔
The problem, "6 divided by 1 ⅔," presents a common mathematical challenge. It involves dividing a whole number (6) by a mixed number (1 ⅔). A mixed number combines a whole number and a proper fraction. To solve this, we must first convert the mixed number into an improper fraction, a fraction where the numerator is greater than the denominator.
Step 1: Converting the Mixed Number to an Improper Fraction
The mixed number 1 ⅔ represents one whole unit and two-thirds of another unit. To convert it into an improper fraction, we follow these steps:
- Multiply the whole number by the denominator: 1 * 3 = 3
- Add the numerator to the result: 3 + 2 = 5
- Keep the same denominator: 3
Therefore, 1 ⅔ is equivalent to the improper fraction ⁵⁄₃.
Step 2: Rewriting the Division Problem
Now we can rewrite the original problem using the improper fraction:
6 ÷ ⁵⁄₃
Step 3: The Reciprocal Method
Dividing by a fraction is the same as multiplying by its reciprocal. The reciprocal of a fraction is obtained by switching the numerator and the denominator. The reciprocal of ⁵⁄₃ is ³⁄₅. So, our problem becomes:
6 * ³⁄₅
Step 4: Multiplying a Whole Number by a Fraction
To multiply a whole number by a fraction, we can rewrite the whole number as a fraction with a denominator of 1:
⁶⁄₁ * ³⁄₅
Step 5: Multiplying Fractions
Multiply the numerators together and the denominators together:
(6 * 3) / (1 * 5) = 18/5
Step 6: Simplifying the Resulting Fraction
The fraction 18/5 is an improper fraction. To express it as a mixed number, we perform division:
18 ÷ 5 = 3 with a remainder of 3
This means that 18/5 is equal to 3 ³⁄₅.
Therefore, 6 divided by 1 ⅔ is equal to 3 ³⁄₅.
Alternative Method: Using Decimal Representation
While the fraction method is generally preferred for accuracy and understanding of fractional concepts, we can also solve this using decimal approximations.
- Convert the mixed number 1 ⅔ into a decimal: 1 + (2/3) ≈ 1 + 0.6667 = 1.6667
- Divide 6 by the decimal approximation: 6 ÷ 1.6667 ≈ 3.6
This decimal approximation (3.6) is close to our fractional answer (3 ³⁄₅), demonstrating the consistency of both methods. However, the fractional method provides a more precise and mathematically rigorous solution, avoiding potential rounding errors inherent in decimal approximations.
Further Exploration: Related Concepts and Applications
Understanding this division process extends beyond a single problem. Let's explore related concepts:
1. Working with Different Mixed Numbers: The process remains the same for dividing any whole number by a mixed number. Simply convert the mixed number into an improper fraction and proceed with the multiplication using reciprocals.
2. Dividing Fractions by Fractions: If you were dividing a fraction by a mixed number (or another fraction), the process would remain similar. Convert both to improper fractions and then multiply by the reciprocal of the divisor.
3. Real-World Applications: This type of calculation is frequently applied in various real-world scenarios:
- Recipe Scaling: If a recipe calls for 1 ⅔ cups of flour and you want to triple the recipe, you would multiply 1 ⅔ by 3, utilizing the same principles.
- Construction and Measurement: In construction, precise measurements are crucial. Dividing lengths or quantities often involves working with fractions and mixed numbers.
- Resource Allocation: Fairly distributing resources among individuals or groups might require dividing a whole quantity by a fraction or mixed number.
- Financial Calculations: Dividing profits or assets often involves working with fractions and decimals.
Troubleshooting Common Mistakes:
- Incorrect Conversion to Improper Fraction: Ensure you correctly convert the mixed number to an improper fraction. A common mistake is incorrectly multiplying or adding during this step.
- Forgetting the Reciprocal: Remember to multiply by the reciprocal of the divisor (the number you are dividing by), not by the original fraction.
- Improper Simplification: Always simplify the final fraction to its lowest terms for the most accurate and concise answer.
Conclusion:
Dividing 6 by 1 ⅔, resulting in 3 ³⁄₅, is a clear demonstration of fundamental mathematical operations involving fractions and mixed numbers. By understanding the process of converting mixed numbers to improper fractions, applying the reciprocal method, and simplifying fractions, you can confidently solve similar problems and apply these skills to various real-world situations. Mastering these concepts builds a strong foundation for more advanced mathematical work. Remember to practice regularly to solidify your understanding and improve your problem-solving skills. The more you practice, the more intuitive these operations will become. This comprehensive guide provides a step-by-step approach, making the seemingly complex problem easily manageable and understandable for all levels of mathematical proficiency.
Latest Posts
Latest Posts
-
What Is The Highest Common Factor Of 20
May 25, 2025
-
Common Factor Of 24 And 33
May 25, 2025
-
What Is 60 Off Of 90
May 25, 2025
-
12000 Btu Air Conditioner Square Footage
May 25, 2025
-
Whats 5 Percent Of 1 Million
May 25, 2025
Related Post
Thank you for visiting our website which covers about 6 Divided By 1 2 As A Fraction . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.