Whats 5 Percent Of 1 Million
listenit
May 25, 2025 · 5 min read
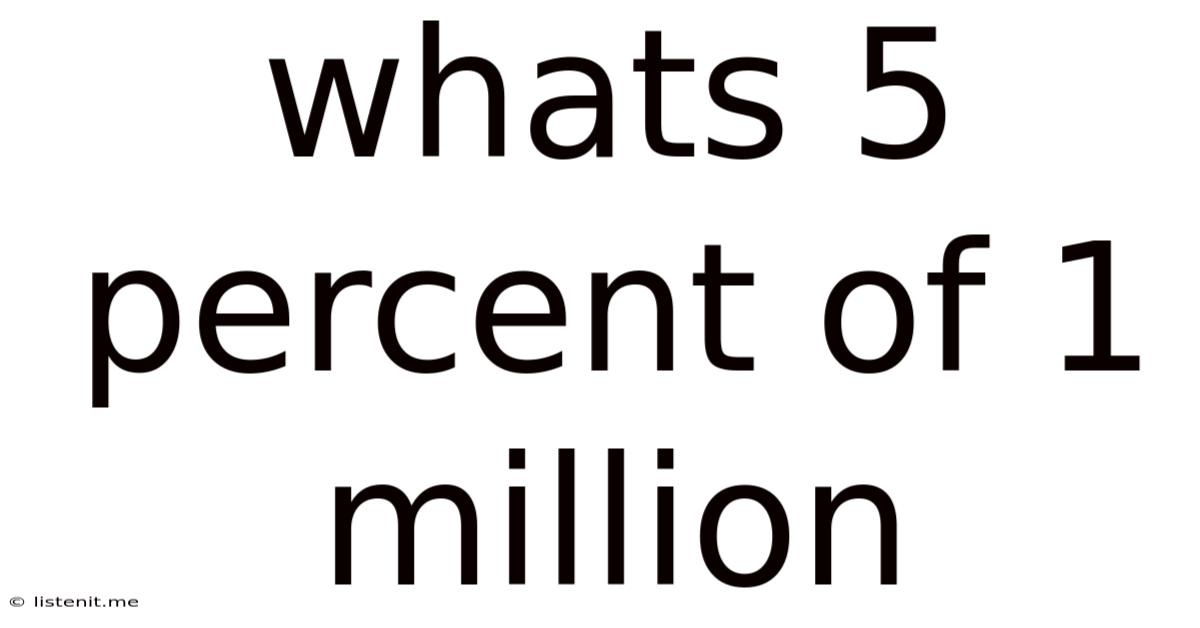
Table of Contents
What's 5 Percent of 1 Million? A Comprehensive Guide to Percentages
Calculating percentages is a fundamental skill with wide-ranging applications, from everyday budgeting to complex financial analyses. Understanding how to determine percentages is crucial for making informed decisions in various aspects of life. This article dives deep into the question, "What's 5 percent of 1 million?", providing a step-by-step explanation, practical examples, and exploring the broader context of percentage calculations.
Understanding Percentages
A percentage is a fraction or ratio expressed as a part of 100. The symbol "%" represents "percent," indicating a portion out of a hundred. For example, 50% means 50 out of 100, which is equivalent to 1/2 or 0.5. Understanding this fundamental concept is key to solving percentage problems.
Key Terms:
- Percentage: The rate, number, or amount in each hundred.
- Base: The whole amount or total value from which the percentage is calculated.
- Part: The portion of the base represented by the percentage.
Calculating 5% of 1 Million: The Methods
There are several ways to calculate 5% of 1 million. Let's explore the most common and straightforward approaches:
Method 1: Using the Decimal Equivalent
The most efficient method involves converting the percentage to its decimal equivalent. To do this, divide the percentage by 100. 5% divided by 100 is 0.05. Now, simply multiply this decimal by the base value (1 million):
0.05 * 1,000,000 = 50,000
Therefore, 5% of 1 million is 50,000.
Method 2: Using Fractions
Percentages can also be expressed as fractions. 5% is equivalent to 5/100, which simplifies to 1/20. To find 5% of 1 million using this fraction, multiply the fraction by the base value:
(1/20) * 1,000,000 = 50,000
This confirms that 5% of 1 million is 50,000.
Method 3: Proportion Method
The proportion method is a versatile approach useful for various percentage problems. We can set up a proportion:
5/100 = x/1,000,000
Where 'x' represents the unknown value (5% of 1 million). To solve for 'x', cross-multiply:
100x = 5,000,000
Now, divide both sides by 100:
x = 50,000
Again, we arrive at the answer: 5% of 1 million is 50,000.
Practical Applications and Real-World Examples
Understanding how to calculate percentages, especially in scenarios involving large numbers like 1 million, is vital in various contexts:
1. Finance and Investments:
- Investment Returns: If you invest $1 million and earn a 5% annual return, you'll gain $50,000.
- Interest Calculations: Calculating simple interest on a $1 million loan at a 5% interest rate involves the same calculation.
- Tax Calculations: Determining the amount of tax owed on a $1 million income with a 5% tax bracket requires this type of calculation.
2. Business and Sales:
- Sales Commissions: A salesperson earning a 5% commission on $1 million in sales would receive $50,000.
- Profit Margins: Calculating a 5% profit margin on a $1 million revenue requires similar calculations.
- Discount Calculations: Offering a 5% discount on a $1 million product results in a $50,000 reduction in price.
3. Data Analysis and Statistics:
- Population Studies: Determining 5% of a population of 1 million provides a useful segment for analysis.
- Market Research: Analyzing 5% of survey respondents from a sample size of 1 million provides valuable insights.
- Scientific Studies: In many scientific fields, calculating percentages from large datasets is common.
4. Everyday Life:
- Tip Calculations: Calculating a 5% tip on a $1,000 bill is a simplified version of this calculation.
- Budgeting: Allocating 5% of your annual income for savings involves a percentage calculation.
- Comparing Prices: Determining the percentage difference between two prices often uses percentage calculations.
Beyond the Basics: Advanced Percentage Calculations
While calculating 5% of 1 million is relatively straightforward, understanding more complex percentage problems is crucial for various applications. Here are some advanced scenarios:
1. Calculating Percentage Increase or Decrease:
Suppose a value increases from $1 million to $1.05 million. To calculate the percentage increase, first find the difference: $1.05 million - $1 million = $50,000. Then, divide the difference by the original value and multiply by 100: ($50,000 / $1,000,000) * 100 = 5%. This indicates a 5% increase. Percentage decrease calculations follow a similar method.
2. Finding the Original Value Given a Percentage:
If you know that a value increased by 5% to reach $1.05 million, you can work backward to find the original value. Let's denote the original value as 'x'. The equation would be: x + 0.05x = $1,050,000. Combining like terms, we have 1.05x = $1,050,000. Dividing both sides by 1.05 gives x = $1,000,000.
3. Dealing with Multiple Percentages:
Calculating consecutive percentages requires careful attention. For example, if a value increases by 5% and then decreases by 5%, the final value will not be the same as the initial value. This is because the second percentage is calculated based on the new value after the first change.
Conclusion: The Power of Percentage Calculations
Understanding how to calculate percentages, particularly involving large numbers like 1 million, is a valuable skill applicable across various fields. Whether you're managing finances, analyzing data, or making everyday decisions, the ability to accurately calculate and interpret percentages provides crucial insights and informs sound judgments. Mastering these calculations empowers you to navigate numerical data with confidence and make informed choices in various aspects of life. The seemingly simple question, "What's 5 percent of 1 million?", opens a door to a wider world of mathematical understanding and practical application. Remember the different methods outlined above – decimal equivalents, fractions, and proportions – to choose the approach that best suits your needs and mathematical comfort level. The key is to practice and apply these methods regularly to build a strong foundation in percentage calculations.
Latest Posts
Latest Posts
-
What Is The Lcm Of 12 And 22
May 25, 2025
-
Gcf Of 16 24 And 40
May 25, 2025
-
Convert 54 2 7 To A Fraction In Lowest Terms
May 25, 2025
-
What Is 58 Kph In Mph
May 25, 2025
-
10 Out Of 35 As A Percentage
May 25, 2025
Related Post
Thank you for visiting our website which covers about Whats 5 Percent Of 1 Million . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.