5 Is What Percent Of 60
listenit
May 24, 2025 · 4 min read
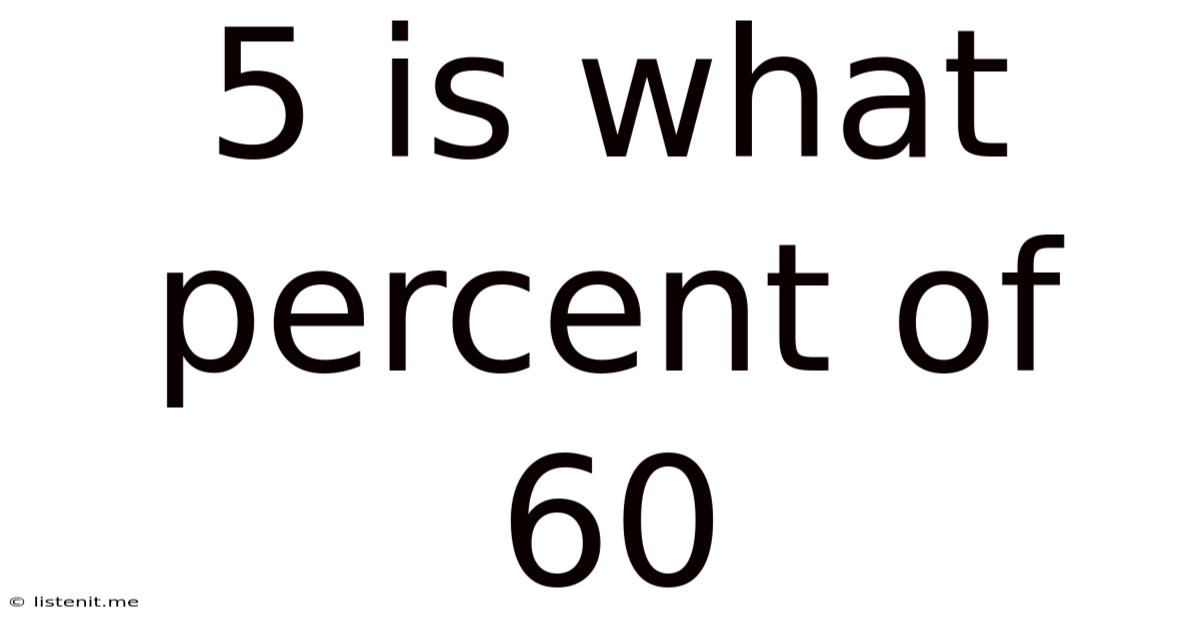
Table of Contents
5 is What Percent of 60? A Comprehensive Guide to Percentage Calculations
Understanding percentages is a fundamental skill in various aspects of life, from calculating discounts and taxes to analyzing data and understanding statistics. This comprehensive guide will delve into the question, "5 is what percent of 60?", providing a step-by-step solution, exploring different calculation methods, and showcasing real-world applications. We'll also touch upon related percentage problems and offer tips for mastering percentage calculations.
Understanding the Fundamentals of Percentages
A percentage is a fraction or ratio expressed as a number out of 100. The symbol "%" represents "percent." Essentially, percentages represent parts of a whole, where the whole is considered 100%.
For example, 50% means 50 out of 100, or 50/100, which simplifies to 1/2. Similarly, 25% means 25 out of 100, or 25/100, which simplifies to 1/4.
Calculating "5 is What Percent of 60?"
The core problem we're tackling is finding what percentage 5 represents when compared to 60. We can approach this problem using two primary methods:
Method 1: Using the Formula
The fundamental formula for percentage calculations is:
(Part / Whole) * 100% = Percentage
In our case:
- Part: 5
- Whole: 60
Substituting these values into the formula:
(5 / 60) * 100% = 8.333...%
Therefore, 5 is approximately 8.33% of 60.
Method 2: Using Proportions
We can also solve this using proportions. We set up a proportion where 'x' represents the unknown percentage:
5/60 = x/100
To solve for 'x', we cross-multiply:
60x = 500
Then, we divide both sides by 60:
x = 500/60 = 8.333...
Again, we find that 5 is approximately 8.33% of 60.
Rounding and Precision
In the calculations above, we encountered a repeating decimal (8.333...). The level of precision required depends on the context. For general purposes, rounding to two decimal places (8.33%) is often sufficient. However, in situations requiring greater accuracy, more decimal places might be necessary.
Real-World Applications
Understanding percentage calculations has widespread applications in various scenarios, including:
1. Discounts and Sales:
Imagine a store offering a 20% discount on an item originally priced at $60. To calculate the discount amount, we use the percentage formula:
(20/100) * $60 = $12
The discount is $12, and the final price would be $60 - $12 = $48.
2. Taxes and Gratuities:
Calculating sales tax or service charges involves similar percentage calculations. If the sales tax rate is 8%, the tax on a $60 item would be:
(8/100) * $60 = $4.80
3. Financial Calculations:
Percentages are crucial in finance. Calculating interest rates, returns on investments, and loan repayments all rely on percentage calculations. Understanding these calculations is essential for making informed financial decisions.
4. Data Analysis and Statistics:
In statistics, percentages are frequently used to represent proportions, such as the percentage of respondents who answered "yes" to a survey question or the percentage change in a stock's value.
5. Grade Calculations:
In education, grades are often expressed as percentages. If a student scores 45 out of 60 on a test, their percentage score would be:
(45/60) * 100% = 75%
Variations and Related Problems
While we've focused on "5 is what percent of 60?", understanding the core concepts allows you to solve similar problems. Here are a few variations:
-
What is 8.33% of 60? This is the reverse of our original problem. Using the formula, (8.33/100) * 60 ≈ 5.
-
60 is what percent of 120? (60/120) * 100% = 50%
-
What number is 20% of 60? (20/100) * 60 = 12
Mastering Percentage Calculations: Tips and Tricks
Here are a few tips to help you master percentage calculations:
-
Memorize common percentages: Familiarize yourself with common percentages like 10%, 20%, 25%, 50%, and 75% to perform mental calculations quickly.
-
Practice regularly: Consistent practice is key to mastering any mathematical skill. Solve various percentage problems to improve your understanding and speed.
-
Use online calculators and resources: While understanding the method is crucial, online calculators can be helpful for verifying your answers or tackling complex calculations.
-
Break down complex problems: If you encounter a complex percentage problem, break it down into smaller, more manageable steps.
-
Check your work: Always double-check your calculations to ensure accuracy.
Conclusion
Understanding how to calculate percentages is a valuable skill with far-reaching implications in daily life and various professional fields. By mastering the fundamental formula, utilizing different methods, and practicing regularly, you can confidently tackle various percentage problems, including determining that 5 is approximately 8.33% of 60. Remember to always consider the level of precision needed when rounding decimals and to apply your knowledge to real-world scenarios to further solidify your understanding. The more you practice, the easier and more intuitive percentage calculations will become.
Latest Posts
Latest Posts
-
How Much Is 1 3 Plus 1 3
May 24, 2025
-
How To Calculate Value Of Pension
May 24, 2025
-
Cap Rate Calculator For Real Estate
May 24, 2025
-
1 Is What Percent Of 14
May 24, 2025
-
What Is 70 Out Of 100
May 24, 2025
Related Post
Thank you for visiting our website which covers about 5 Is What Percent Of 60 . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.