4 To The Power Of Negative 1
listenit
Mar 28, 2025 · 4 min read
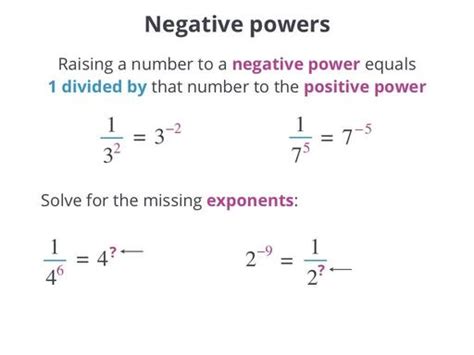
Table of Contents
4 to the Power of Negative 1: A Deep Dive into Negative Exponents
Understanding exponents is fundamental to mathematics and numerous applications across science and engineering. While positive exponents represent repeated multiplication, negative exponents introduce a fascinating twist, representing the reciprocal. This article delves deep into the meaning and implications of 4 to the power of negative 1 (4⁻¹), exploring its calculation, applications, and its broader context within the wider world of exponents and powers.
What Does 4⁻¹ Mean?
At its core, 4⁻¹ signifies the reciprocal of 4. The negative exponent indicates that we're dealing with the multiplicative inverse. In simpler terms, we're looking for the number that, when multiplied by 4, equals 1. This number is, of course, 1/4 or 0.25.
Therefore, 4⁻¹ = 1/4 = 0.25
This concept applies to any base raised to a negative power. For any non-zero number 'a' and any integer 'n', a⁻ⁿ = 1/aⁿ.
Calculating 4⁻¹: A Step-by-Step Guide
Calculating 4⁻¹ is straightforward. The process is summarized below:
- Identify the base and the exponent: In 4⁻¹, the base is 4 and the exponent is -1.
- Apply the rule for negative exponents: Recall that a⁻ⁿ = 1/aⁿ. Therefore, 4⁻¹ becomes 1/4¹.
- Simplify: 4¹ is simply 4, so the expression simplifies to 1/4.
- Convert to decimal (optional): 1/4 can be expressed as the decimal 0.25.
The Broader Context: Negative Exponents and Their Properties
Understanding 4⁻¹ requires a solid grasp of negative exponents in general. Let's explore some key properties:
1. The Reciprocal Relationship:
The most crucial aspect is the reciprocal relationship. A negative exponent essentially "flips" the base into its reciprocal. This means:
- a⁻ⁿ = 1/aⁿ (For any non-zero 'a' and integer 'n')
2. Product Rule with Negative Exponents:
When multiplying numbers with the same base but different exponents (both positive and negative), we add the exponents:
- aᵐ * aⁿ = aᵐ⁺ⁿ
This rule holds true even when 'm' or 'n' are negative. For example:
4² * 4⁻¹ = 4⁽²⁻¹⁾ = 4¹ = 4
3. Quotient Rule with Negative Exponents:
When dividing numbers with the same base and different exponents, we subtract the exponents:
- aᵐ / aⁿ = aᵐ⁻ⁿ
Again, this rule works flawlessly with negative exponents:
4³ / 4⁻² = 4⁽³⁻(-²)⁾ = 4⁵ = 1024
4. Power of a Power Rule with Negative Exponents:
When raising a power to another power, we multiply the exponents:
- (aᵐ)ⁿ = aᵐⁿ
This rule remains consistent even with negative exponents:
(4⁻²)³ = 4⁽⁻²*³⁾ = 4⁻⁶ = 1/4⁶ = 1/4096
Applications of Negative Exponents
Negative exponents are not just abstract mathematical concepts; they have practical applications across various fields:
1. Scientific Notation:
Scientific notation uses powers of 10 to represent very large or very small numbers. Negative exponents are essential for expressing small numbers. For instance, 0.000001 can be written as 10⁻⁶. This concise representation simplifies calculations and improves readability in scientific contexts.
2. Unit Conversions:
In unit conversions, negative exponents often appear when converting between units of different scales. For example, converting kilometers to millimeters would involve a power of 10 with a negative exponent.
3. Compound Interest Calculations:
In finance, particularly when dealing with compound interest calculations over time, negative exponents can show the present value of future amounts. This is crucial for financial planning, investment analysis and loan amortization calculations.
4. Physics and Engineering:
Negative exponents frequently appear in physics and engineering formulas. For example, in inverse square laws (like the inverse square law of gravity), the distance is raised to a negative exponent, indicating that the force or intensity decreases as the square of the distance increases.
5. Computer Science and Data Storage:
In computer science, especially when dealing with data storage and memory management, negative exponents often represent the scaling factors of exponentially large or small values.
Beyond 4⁻¹: Exploring Other Negative Exponents
While we've focused on 4⁻¹, the concepts discussed extend to any base raised to a negative exponent. Let's consider a few examples:
- 2⁻³ = 1/2³ = 1/8 = 0.125
- 10⁻² = 1/10² = 1/100 = 0.01
- (1/3)⁻¹ = 3¹ = 3 (Note that the reciprocal of a fraction flips the numerator and denominator)
Common Mistakes to Avoid
When working with negative exponents, it’s crucial to avoid common errors:
- Confusing negative exponents with negative numbers: A negative exponent does not make the result negative. It simply indicates a reciprocal.
- Incorrectly applying the rules of exponents: Always ensure you correctly apply the product, quotient, and power of a power rules, especially when dealing with a mixture of positive and negative exponents.
- Misinterpreting the order of operations: Remember to follow the order of operations (PEMDAS/BODMAS) to ensure calculations are accurate.
Conclusion: Mastering Negative Exponents
Understanding 4⁻¹ is a stepping stone to mastering negative exponents more broadly. This concept, while seemingly simple at first glance, unlocks a powerful tool for solving problems across various disciplines. By grasping the reciprocal relationship and applying the rules of exponents consistently, you'll be well-equipped to tackle more complex mathematical problems and deepen your understanding of mathematical concepts that govern many aspects of our physical world. Remember to practice regularly and use various examples to reinforce your understanding. The more you work with negative exponents, the more intuitive they will become. The journey of learning never stops, and continued exploration will solidify your mastery of this essential mathematical concept.
Latest Posts
Latest Posts
-
What Is The Name Of This Hydrocarbon
Mar 31, 2025
-
Columns Of Periodic Table Are Called
Mar 31, 2025
-
Difference Between Animal Mitosis And Plant Mitosis
Mar 31, 2025
-
What Is Half Of One And A Half Teaspoons
Mar 31, 2025
-
What Is 4 12 In Simplest Form
Mar 31, 2025
Related Post
Thank you for visiting our website which covers about 4 To The Power Of Negative 1 . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.
