39 Is What Percent Of 12.5
listenit
Mar 28, 2025 · 5 min read
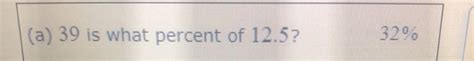
Table of Contents
39 is What Percent of 12.5? Unpacking Percentage Calculations and Their Applications
This seemingly simple question – "39 is what percent of 12.5?" – opens a door to a vast world of mathematical concepts and real-world applications. Understanding how to solve this, and similar percentage problems, is crucial for numerous aspects of life, from budgeting and finance to data analysis and scientific research. This article will not only provide the solution but delve deep into the underlying principles, explore different calculation methods, and showcase the diverse applications of percentage calculations.
Understanding Percentages: A Foundation
Before tackling the specific problem, let's solidify our understanding of percentages. A percentage is simply a fraction expressed as a part of 100. The symbol "%" represents "per hundred" or "out of 100". For example, 50% means 50 out of 100, which is equivalent to the fraction 50/100 or the decimal 0.5.
Method 1: Using the Percentage Formula
The most straightforward method to solve "39 is what percent of 12.5?" is using the fundamental percentage formula:
(Part / Whole) * 100% = Percentage
In our problem:
- Part: 39 (the value we're considering as a part of the whole)
- Whole: 12.5 (the total value)
Plugging these values into the formula:
(39 / 12.5) * 100% = 312%
Therefore, 39 is 312% of 12.5.
Method 2: Solving with Proportions
Another effective method involves setting up a proportion. We can represent the problem as:
39 / 12.5 = x / 100
Where 'x' represents the unknown percentage. To solve for 'x', we cross-multiply:
39 * 100 = 12.5 * x
3900 = 12.5x
x = 3900 / 12.5
x = 312
Again, we find that 39 is 312% of 12.5.
Method 3: Using Decimal Conversion
This method involves converting the percentage into a decimal and then solving an equation. We can represent the problem as:
39 = x * 12.5
Where 'x' is the decimal representation of the percentage. Solving for 'x':
x = 39 / 12.5
x = 3.12
To convert this decimal back into a percentage, we multiply by 100%:
3.12 * 100% = 312%
Interpreting the Result: Percentages Greater Than 100%
It's important to note that the result, 312%, is a percentage greater than 100%. This is perfectly valid and simply means that the "part" (39) is larger than the "whole" (12.5). This often occurs in scenarios involving growth, increase, or comparisons where the final value exceeds the initial value.
Real-World Applications of Percentage Calculations
Percentage calculations are incredibly versatile and find applications in numerous fields:
-
Finance: Calculating interest rates, loan repayments, profit margins, discounts, tax rates, and investment returns all rely heavily on percentage calculations. For instance, understanding compound interest, a concept involving repeated percentage calculations, is crucial for long-term financial planning.
-
Data Analysis: Percentages are essential for interpreting data, expressing proportions, and comparing different datasets. For example, in market research, percentages are used to represent market share, customer satisfaction rates, and conversion rates. In scientific studies, percentages are often used to report statistical significance and experimental results.
-
Retail and Sales: Calculating discounts, markups, sales tax, and profit margins are fundamental to retail operations. Understanding percentage change is critical for tracking sales performance and making informed business decisions.
-
Science and Engineering: Percentages are used to express concentrations (e.g., percentage composition of a solution), efficiency (e.g., engine efficiency), and error margins in scientific experiments and engineering calculations.
-
Everyday Life: From calculating tips at restaurants to understanding nutrition labels (percentage of daily value), percentages are integral parts of our daily lives.
Beyond the Basics: Further Exploration of Percentage Problems
While this article focused on a specific percentage problem, the principles extend to a wide range of related calculations:
-
Percentage Increase/Decrease: Calculating the percentage change between two values. For example, determining the percentage increase in sales from one year to the next.
-
Finding the Whole Given a Percentage and Part: If you know a percentage and the corresponding part, you can use the percentage formula to calculate the whole.
-
Finding the Part Given a Percentage and Whole: This is the most common type of percentage problem, similar to the one we solved initially.
-
Compound Percentages: Calculating percentages on top of previous percentages, often found in scenarios like compound interest.
-
Percentage Points: Distinguishing between percentage change and percentage points, which represent absolute differences in percentages rather than relative changes.
Mastering Percentage Calculations: Tips and Practice
Developing proficiency in percentage calculations requires consistent practice. Here are some tips to improve your skills:
-
Understand the Fundamentals: Ensure you have a solid grasp of the basic principles of percentages, fractions, and decimals.
-
Practice Regularly: Solve various percentage problems to build confidence and improve your problem-solving skills. Utilize online resources, textbooks, or practice worksheets to access a wide range of problems.
-
Use Different Methods: Become comfortable using multiple methods (formula, proportions, decimals) to solve percentage problems. This allows you to choose the most efficient approach for each specific problem.
-
Check Your Answers: Always verify your answers to ensure accuracy. Use calculators or online tools to check your calculations.
Conclusion: The Power of Percentages
The seemingly simple question, "39 is what percent of 12.5?", provides a springboard to explore the multifaceted world of percentage calculations and their pervasive applications. By understanding the underlying principles and mastering different calculation methods, you'll be equipped to tackle numerous real-world problems involving percentages, thereby enhancing your analytical skills and decision-making abilities across various aspects of life. The ability to confidently work with percentages is a valuable skill that will serve you well in your academic pursuits, professional endeavors, and daily life.
Latest Posts
Latest Posts
-
Do Exponential Functions Have Vertical Asymptotes
Mar 31, 2025
-
How Many Ounces Is 1 4 Pound
Mar 31, 2025
-
What Is 65 As A Fraction
Mar 31, 2025
-
What Number Is 60 Of 145
Mar 31, 2025
-
The Ability Of An Organism To Survive And Reproduce
Mar 31, 2025
Related Post
Thank you for visiting our website which covers about 39 Is What Percent Of 12.5 . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.
