35 Out Of 60 As A Percentage
listenit
May 25, 2025 · 5 min read
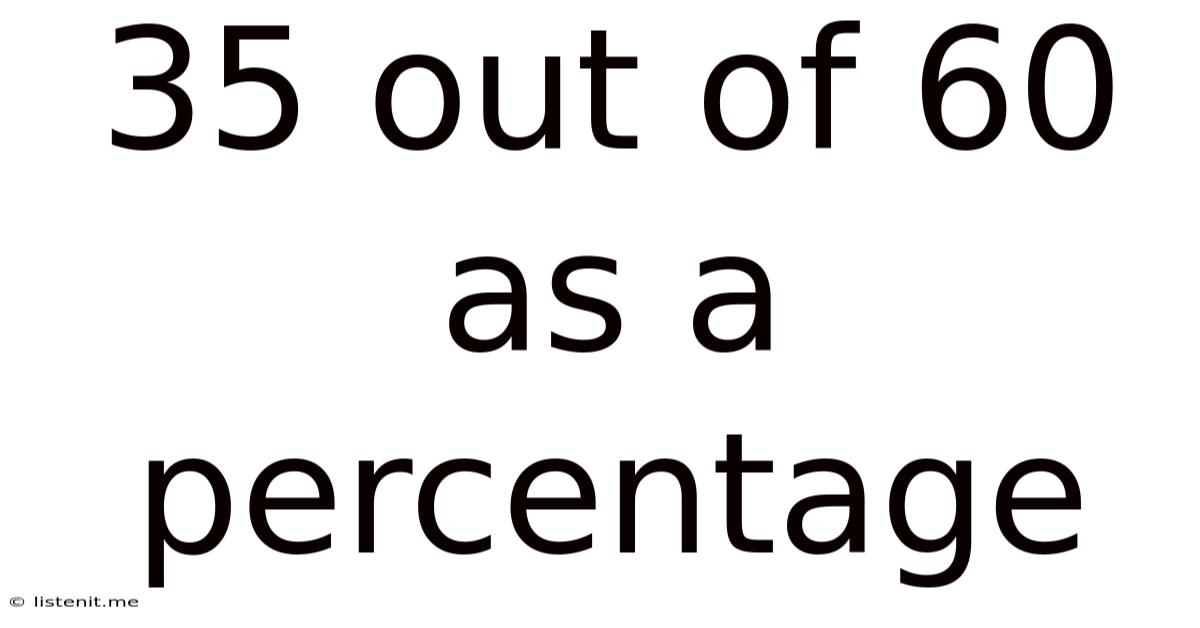
Table of Contents
Decoding 35 out of 60 as a Percentage: A Comprehensive Guide
Understanding percentages is a fundamental skill in various aspects of life, from calculating discounts and taxes to analyzing data and assessing performance. This comprehensive guide delves into the process of converting fractions into percentages, specifically focusing on how to calculate 35 out of 60 as a percentage. We'll explore different methods, discuss the applications of this calculation, and provide you with a firm grasp of this essential mathematical concept.
Understanding Percentages: The Basics
A percentage is a way of expressing a number as a fraction of 100. The word "percent" literally means "per hundred." For example, 50% means 50 out of 100, or 50/100, which simplifies to 1/2. Percentages are useful because they allow for easy comparison of different proportions.
Key Terms and Concepts
Before we dive into the calculation, let's clarify some crucial terms:
- Numerator: The top number in a fraction (in this case, 35). It represents the part.
- Denominator: The bottom number in a fraction (in this case, 60). It represents the whole.
- Percentage: The expression of the fraction as a number out of 100.
Calculating 35 out of 60 as a Percentage: Three Methods
There are several methods to calculate 35 out of 60 as a percentage. Let's explore three common approaches:
Method 1: The Fraction Method
This method involves converting the fraction 35/60 into a percentage. Here's how:
-
Form the fraction: Express the problem as a fraction: 35/60.
-
Convert to a decimal: Divide the numerator (35) by the denominator (60): 35 ÷ 60 = 0.58333...
-
Multiply by 100: Multiply the decimal by 100 to convert it to a percentage: 0.58333... × 100 = 58.333...%
-
Round (optional): Round the percentage to the desired level of precision. Rounding to two decimal places, we get 58.33%.
Method 2: The Proportion Method
This method uses proportions to solve for the percentage. It's particularly useful when dealing with more complex percentage problems.
-
Set up a proportion: We can set up a proportion as follows:
35/60 = x/100
where 'x' represents the percentage we're trying to find.
-
Cross-multiply: Cross-multiply the terms: 35 × 100 = 60 × x
-
Solve for x: This simplifies to 3500 = 60x. Divide both sides by 60: x = 3500/60 = 58.333...
-
Express as a percentage: Therefore, x ≈ 58.33%.
Method 3: Using a Calculator
The easiest method involves using a calculator. Simply divide 35 by 60 and then multiply the result by 100. Most calculators have a percentage function (%) that simplifies this process even further.
Applications of Percentage Calculations: Real-World Examples
Understanding percentage calculations is crucial in numerous real-world scenarios. Here are some examples where this skill proves invaluable:
1. Academic Performance:
Imagine a student scored 35 out of 60 on a test. Calculating the percentage (58.33%) helps assess their performance relative to the total marks. This allows for a clear understanding of their strengths and weaknesses and helps identify areas needing improvement. Furthermore, schools and universities often use percentages to represent grade point averages (GPAs), providing a standardized way to evaluate academic progress.
2. Sales and Discounts:
Retailers frequently offer discounts expressed as percentages. For instance, a "30% off" sale means you pay 70% of the original price. Knowing how to calculate percentages is essential for determining the final price after a discount. Similarly, understanding sales tax (typically expressed as a percentage) is vital for calculating the total cost of a purchase.
3. Financial Calculations:
Percentages are fundamental in finance. Interest rates on loans and savings accounts are expressed as percentages, impacting the amount you pay or earn over time. Calculating percentage change is crucial for tracking investment performance, analyzing market trends, and understanding the growth or decline of assets. Return on investment (ROI), a key metric for evaluating investment success, is also expressed as a percentage.
4. Data Analysis and Statistics:
Percentage calculations are essential for data analysis. Researchers and analysts often use percentages to represent proportions within datasets, making it easier to interpret and compare different groups or categories. For example, the percentage of a population with a specific characteristic, the success rate of a particular treatment, or the market share of a company are all commonly expressed as percentages.
5. Everyday Life:
Even in everyday life, understanding percentages is beneficial. Calculating tips in restaurants, determining the percentage of ingredients in recipes, or figuring out the discount on sale items are all examples where percentage knowledge comes in handy.
Beyond the Calculation: Understanding the Context
While calculating 35 out of 60 as a percentage yields 58.33%, the significance of this number depends heavily on the context. A score of 58.33% on a math test might be considered acceptable, but a 58.33% success rate for a critical surgery would be alarming.
Therefore, always consider the context when interpreting percentage results. The numerical value alone doesn't tell the whole story; understanding the implications within the specific situation is paramount.
Improving Percentage Calculation Skills
Mastering percentage calculations can significantly improve your numerical literacy and problem-solving abilities. Here are some tips to enhance your skills:
-
Practice regularly: The more you practice, the more comfortable and efficient you'll become. Try solving various percentage problems from different contexts.
-
Use different methods: Experiment with the various methods discussed in this guide (fraction, proportion, calculator) to find the approach that suits your preference and the problem's complexity.
-
Understand the underlying concepts: Don't just memorize formulas; strive to grasp the fundamental principles behind percentage calculations. This will enable you to apply your knowledge more effectively in diverse situations.
-
Seek help when needed: If you're struggling with specific aspects of percentage calculations, don't hesitate to seek assistance from teachers, tutors, or online resources. There are numerous tutorials and practice exercises available that can help solidify your understanding.
Conclusion: The Power of Percentages
The ability to calculate and interpret percentages is a valuable skill applicable to numerous aspects of life. By understanding the different methods and their applications, you can confidently tackle percentage problems and leverage this knowledge to make informed decisions across various domains. Remember that the significance of a percentage isn't solely determined by the numerical value, but also by the context in which it is presented. Understanding this nuance is vital for making sound judgments and extracting meaningful insights from data. Mastering percentages equips you with a powerful tool for navigating a data-driven world.
Latest Posts
Latest Posts
-
What Is 1 5 Of 80
May 25, 2025
-
Greatest Common Factor Of 22 And 88
May 25, 2025
-
Least Common Multiple Of 10 And 11
May 25, 2025
-
Whats 20 Percent Of 30 Dollars
May 25, 2025
-
Personal Loan Lump Sum Repayment Calculator
May 25, 2025
Related Post
Thank you for visiting our website which covers about 35 Out Of 60 As A Percentage . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.