1/3 Divided By 1/2 As A Fraction
listenit
Apr 01, 2025 · 5 min read
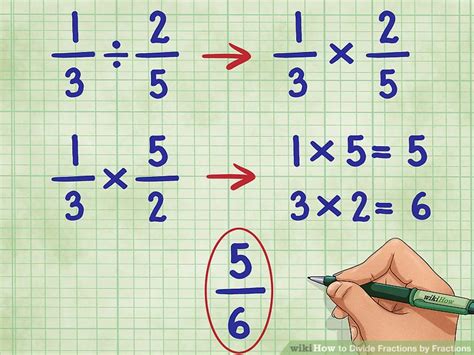
Table of Contents
1/3 Divided by 1/2 as a Fraction: A Comprehensive Guide
Understanding fractions and how to perform operations on them is a fundamental skill in mathematics. While seemingly simple, dividing fractions can be a source of confusion for many. This comprehensive guide will walk you through the process of dividing 1/3 by 1/2, explaining the underlying concepts and providing various approaches to solve the problem. We'll also explore related concepts and applications to ensure a thorough understanding.
Understanding Fraction Division
Before diving into the specific problem of 1/3 divided by 1/2, let's establish a solid foundation in fraction division. The core principle is that dividing by a fraction is the same as multiplying by its reciprocal. The reciprocal of a fraction is simply the fraction flipped upside down. For example, the reciprocal of 1/2 is 2/1 (or simply 2).
Key Concept: Dividing by a fraction is equivalent to multiplying by its reciprocal.
This principle simplifies the process significantly. Instead of directly dividing by a fraction, we can convert the division problem into a multiplication problem, making it easier to solve.
Solving 1/3 Divided by 1/2
Now, let's tackle the problem at hand: 1/3 divided by 1/2. Using the principle of reciprocals, we can rewrite the problem as follows:
1/3 ÷ 1/2 = 1/3 × 2/1
This simplifies to:
1/3 × 2/1 = (1 × 2) / (3 × 1) = 2/3
Therefore, 1/3 divided by 1/2 equals 2/3.
Visualizing Fraction Division
Visual representations can greatly aid in understanding fraction division. Imagine you have 1/3 of a pizza. If you want to divide this 1/3 into two equal parts (dividing by 1/2), you're effectively finding half of 1/3. This results in a larger fraction than the initial 1/3, which is represented by 2/3. You now have two smaller pieces making up a total of 2/3 of the pizza.
Alternative Methods for Solving Fraction Division
While the reciprocal method is the most efficient, let's explore alternative methods to solidify our understanding.
Method 1: Using Common Denominators
This method involves finding a common denominator for both fractions before performing the division. The common denominator for 1/3 and 1/2 is 6. Therefore, we rewrite the fractions:
1/3 = 2/6 1/2 = 3/6
Now, we can rewrite the division problem:
(2/6) ÷ (3/6)
Dividing the numerators and denominators separately:
2 ÷ 3 = 2/3 6 ÷ 6 = 1
So, (2/6) ÷ (3/6) = 2/3
This method confirms our previous result.
Method 2: Converting to Decimals (Less Precise)
We can convert the fractions to decimals before performing the division.
1/3 ≈ 0.3333 1/2 = 0.5
0.3333 ÷ 0.5 ≈ 0.6666
Converting this decimal back to a fraction approximates 2/3. However, this method is less precise than working directly with fractions, as it introduces rounding errors.
Applications of Fraction Division in Real Life
Fraction division isn't just a theoretical concept; it has numerous practical applications in everyday life and various professions:
-
Cooking and Baking: Recipes often require dividing ingredients into fractions. For example, if a recipe calls for 1/3 cup of sugar and you only want to make half the recipe, you need to divide 1/3 by 1/2 to find the amount of sugar needed.
-
Sewing and Tailoring: Cutting fabric for sewing projects frequently involves dividing lengths into fractions of yards or inches.
-
Construction and Engineering: Precise measurements and calculations are crucial in construction and engineering, where dividing fractions often determines the accurate dimensions and proportions of structures.
-
Finance and Budgeting: Allocating budgets and calculating percentages involve dividing fractions and decimals. For instance, determining a portion of a budget for a specific expense might necessitate fraction division.
-
Data Analysis: In data analysis, we often deal with proportions and percentages which necessitate fraction calculations, including division.
Expanding on Fraction Concepts: Mixed Numbers and Improper Fractions
To further enhance our understanding, let's briefly explore mixed numbers and improper fractions, which are closely related to the concept of fraction division.
Mixed Numbers: A mixed number combines a whole number and a fraction (e.g., 1 1/2).
Improper Fractions: An improper fraction has a numerator larger than or equal to its denominator (e.g., 5/4).
It's often necessary to convert between mixed numbers and improper fractions when performing operations such as division. For example, if we were dividing a mixed number by a fraction, we would first convert the mixed number into an improper fraction before applying the reciprocal method.
Advanced Fraction Operations: Complex Fractions and More
Building upon our knowledge of fraction division, we can explore more complex scenarios:
Complex Fractions: These fractions have a fraction in either the numerator, the denominator, or both. For instance, (1/3) / (1/2 + 1/4) would be a complex fraction. Solving these requires addressing the numerator and denominator separately before proceeding with the division.
Troubleshooting Common Mistakes in Fraction Division
Several common errors can occur when dealing with fraction division. Here are a few pitfalls to avoid:
-
Incorrectly applying the reciprocal: Remember to flip only the divisor (the fraction you're dividing by) and not the dividend (the fraction being divided).
-
Multiplying instead of dividing: The reciprocal method is a means to convert a division problem into multiplication, but remember to use multiplication after finding the reciprocal of the divisor.
-
Incorrect simplification: Always simplify the resulting fraction to its lowest terms by finding the greatest common divisor (GCD) of the numerator and denominator.
Conclusion: Mastering Fraction Division
Mastering fraction division is an essential skill with numerous real-world applications. By understanding the principle of reciprocals, employing various methods, and avoiding common mistakes, you can confidently tackle fraction division problems of varying complexities. This guide has provided a detailed walkthrough of dividing 1/3 by 1/2, along with broader insights into related fraction concepts. Continued practice and exploration of more complex examples will further solidify your understanding and build your problem-solving skills in this crucial area of mathematics. Remember, consistent practice is key to mastery.
Latest Posts
Latest Posts
-
Is Boiling Water A Chemical Reaction
Apr 02, 2025
-
1 Pint Is How Many Pounds
Apr 02, 2025
-
What Kingdom Do Humans Belong To
Apr 02, 2025
-
Is 1 2 An Irrational Number
Apr 02, 2025
-
5 Converted To A Decimal Is
Apr 02, 2025
Related Post
Thank you for visiting our website which covers about 1/3 Divided By 1/2 As A Fraction . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.
