Write 2 97 100 As A Decimal.
listenit
May 25, 2025 · 5 min read
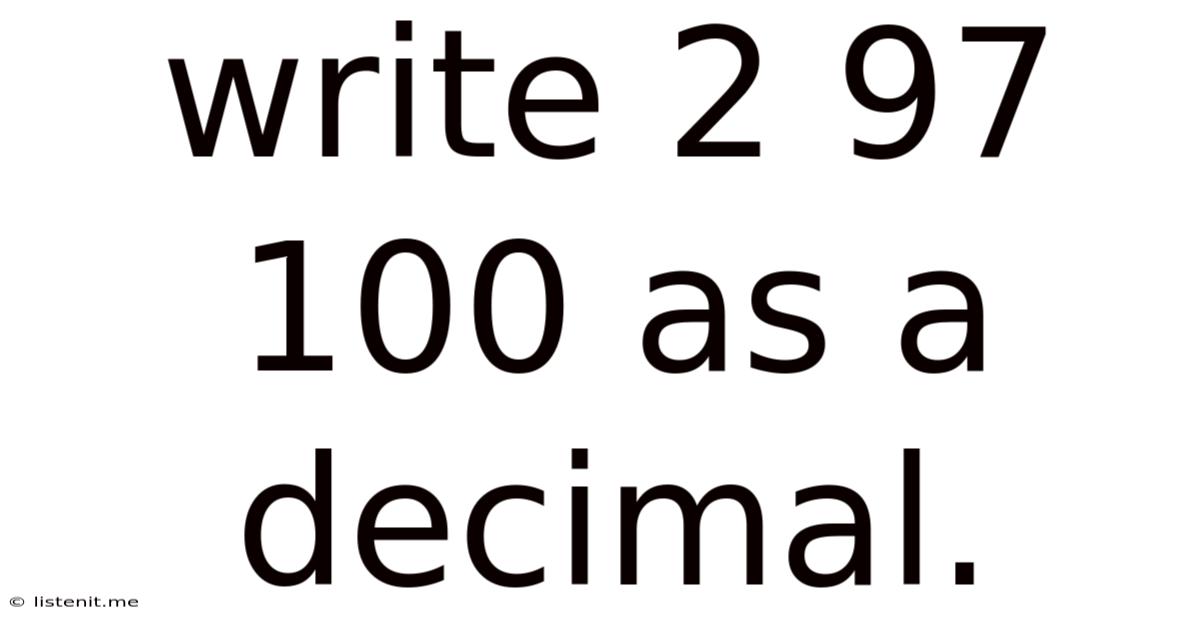
Table of Contents
Write 2 97 100 as a Decimal: A Comprehensive Guide
The seemingly simple question, "Write 2 97 100 as a decimal," opens a door to a deeper understanding of number systems and their representation. This comprehensive guide will not only answer this question directly but also delve into the underlying concepts, exploring various methods and addressing potential confusion along the way. We'll cover different approaches to converting mixed numbers to decimals, providing a thorough and accessible explanation suitable for a wide range of readers, from beginners to those seeking a refresher on fundamental mathematical principles.
Understanding the Number System
Before we tackle the conversion, let's solidify our understanding of the number system involved. The expression "2 97 100" represents a mixed number. A mixed number combines a whole number (2 in this case) and a fraction (97/100). The fraction indicates a part of a whole, where the numerator (97) represents the number of parts and the denominator (100) represents the total number of equal parts the whole is divided into.
Decimals, on the other hand, are another way of representing numbers, using a base-ten system. They use a decimal point (.) to separate the whole number part from the fractional part. The digits to the right of the decimal point represent tenths, hundredths, thousandths, and so on.
Method 1: Direct Conversion using Decimal Equivalents
The simplest method to convert 2 97/100 to a decimal utilizes the understanding of place value. Since the denominator is 100, we can directly interpret the fraction as representing hundredths. The numerator, 97, signifies 97 hundredths.
Therefore, 97/100 can be directly written as 0.97. Combining this with the whole number part, we arrive at the final answer:
2.97
Method 2: Long Division
While the previous method was straightforward due to the denominator being 100, let's consider a more general approach applicable to any fraction. Long division offers a systematic way to convert any fraction into a decimal.
To convert 97/100 using long division, we divide the numerator (97) by the denominator (100).
0.97
100 | 97.00
-900
700
-700
0
The result of the long division is 0.97. Adding the whole number part (2), we obtain the decimal representation:
2.97
This method reinforces the concept of a fraction representing division and provides a practical approach for fractions with denominators other than powers of 10.
Method 3: Converting to an Improper Fraction
Another approach involves first converting the mixed number into an improper fraction and then performing the division. An improper fraction is a fraction where the numerator is greater than or equal to the denominator.
To convert 2 97/100 to an improper fraction:
- Multiply the whole number by the denominator: 2 * 100 = 200
- Add the numerator: 200 + 97 = 297
- Keep the same denominator: The denominator remains 100.
This gives us the improper fraction 297/100. Now, we perform the long division:
2.97
100 | 297.00
-2000
970
-900
700
-700
0
The result, 2.97, is consistent with the previous methods. This approach is particularly useful when dealing with mixed numbers with larger whole number parts or fractions that aren't easily converted to decimals directly.
Practical Applications and Real-World Examples
Understanding the conversion of mixed numbers to decimals extends beyond theoretical mathematics. It has numerous practical applications in various fields:
- Finance: Calculating interest rates, discounts, or tax amounts often involves working with decimals and fractions. Converting mixed numbers to decimals streamlines these calculations.
- Measurement: Many measurements, especially in metric systems, utilize decimal representations. Converting mixed units to decimal equivalents facilitates accurate calculations and comparisons.
- Science: Scientific data often involves fractions and decimals. Converting mixed number representations to decimal form is crucial for data analysis and reporting.
- Engineering: Precision in engineering demands accurate calculations. Converting mixed numbers to decimals ensures accuracy in design, manufacturing, and construction.
- Everyday Life: From calculating tips at restaurants to measuring ingredients for cooking, understanding decimal representations is essential for everyday tasks.
Addressing Potential Confusion and Common Mistakes
While the conversion process is relatively straightforward, some common pitfalls can lead to errors. Let's address some potential sources of confusion:
- Incorrect placement of the decimal point: Ensure the decimal point is placed correctly after the whole number and before the fractional part. A misplaced decimal point drastically alters the value of the number.
- Misunderstanding of place value: A firm grasp of place value (ones, tenths, hundredths, etc.) is crucial for accurate conversions. Any misunderstanding of place value can lead to incorrect results.
- Errors in long division: Carefully perform the long division to avoid errors. Double-checking your work is crucial to ensure accuracy.
- Incorrect conversion to improper fractions: Ensure accurate multiplication and addition when converting mixed numbers to improper fractions before performing the division.
Expanding the Scope: Converting Fractions with Different Denominators
While we focused on a denominator of 100, the principles remain the same for fractions with other denominators. The key is to either:
- Simplify the fraction: Reduce the fraction to its simplest form before conversion. This can make the long division easier.
- Use long division: Long division is a universal method applicable to any fraction.
- Find an equivalent fraction with a denominator that is a power of 10: If possible, convert the fraction to an equivalent one with a denominator like 10, 100, 1000, etc., making direct conversion to a decimal simple.
Conclusion: Mastering Decimal Conversions
The conversion of 2 97/100 to its decimal equivalent, 2.97, serves as a foundation for understanding broader concepts in number systems and their representation. We explored three different methods—direct conversion, long division, and conversion to an improper fraction—each offering a valuable perspective on the process. Understanding these methods, along with their practical applications and the potential pitfalls, empowers you to confidently handle decimal conversions in various contexts. By mastering these skills, you enhance your mathematical proficiency and problem-solving capabilities, proving invaluable in academic, professional, and everyday scenarios. Remember, the key is understanding the underlying principles and choosing the method best suited to the given problem.
Latest Posts
Latest Posts
-
Ac Tonnage Calculator Per Square Meter
May 25, 2025
-
7 Years Ago Was What Year
May 25, 2025
-
1 4 Divided By 4 In Fraction
May 25, 2025
-
What Is 72 As A Fraction
May 25, 2025
-
Highest Common Factor Of 84 And 24
May 25, 2025
Related Post
Thank you for visiting our website which covers about Write 2 97 100 As A Decimal. . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.