Work Equals Change In Kinetic Energy
listenit
May 10, 2025 · 6 min read
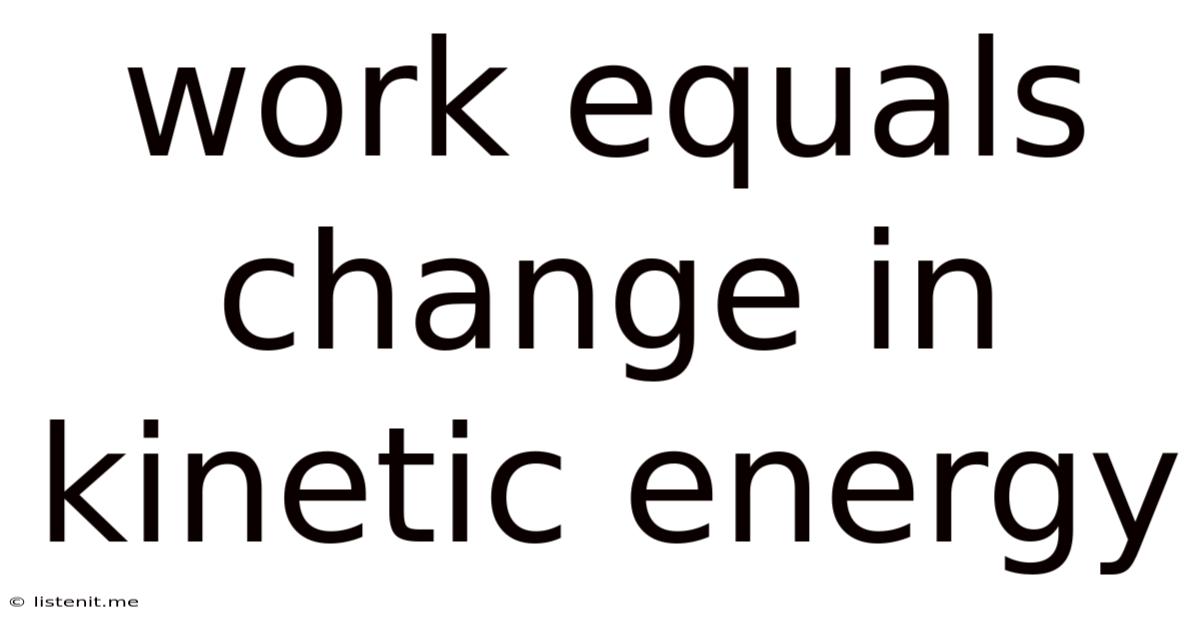
Table of Contents
Work Equals Change in Kinetic Energy: A Deep Dive into the Work-Energy Theorem
The Work-Energy Theorem is a fundamental principle in physics that elegantly connects the concepts of work and energy. It states that the net work done on an object is equal to the change in its kinetic energy. This seemingly simple statement underpins a vast array of physical phenomena, from the motion of projectiles to the operation of complex machinery. This article will delve deep into the Work-Energy Theorem, exploring its derivation, applications, and implications.
Understanding the Key Concepts: Work and Kinetic Energy
Before diving into the theorem itself, let's define its core components: work and kinetic energy.
What is Work?
In physics, work is not simply an activity; it's a precise quantity representing the energy transferred to or from an object via a force acting upon that object. Crucially, work is only done if the force causes a displacement of the object. The formula for work (W) is:
W = Fd cos θ
Where:
- F represents the magnitude of the force applied.
- d represents the magnitude of the displacement.
- θ represents the angle between the force vector and the displacement vector.
This equation highlights a crucial point: work is a scalar quantity, meaning it has magnitude but no direction. If the force and displacement are parallel (θ = 0°), the work done is maximized (W = Fd). If they are perpendicular (θ = 90°), no work is done (W = 0), even if a significant force is applied. Consider pushing a wall: you exert a force, but the wall doesn't move, resulting in zero work done.
What is Kinetic Energy?
Kinetic energy (KE) is the energy an object possesses due to its motion. A stationary object has zero kinetic energy. The faster an object moves and the greater its mass, the more kinetic energy it possesses. The formula for kinetic energy is:
KE = ½mv²
Where:
- m represents the mass of the object.
- v represents the velocity of the object.
Kinetic energy is also a scalar quantity, always positive (or zero). This means it doesn't have a direction associated with it.
Deriving the Work-Energy Theorem
The Work-Energy Theorem can be derived using Newton's second law of motion (F = ma) and a bit of calculus. Consider an object of mass 'm' moving along a straight line under the influence of a net force 'F'. Newton's second law states that:
F = ma
Where 'a' is the acceleration of the object. Acceleration is the rate of change of velocity (a = dv/dt), and velocity is the rate of change of displacement (v = dx/dt). We can rewrite Newton's second law as:
F = m(dv/dt)
Now, let's consider the work done by the net force over a small displacement 'dx':
dW = Fdx = m(dv/dt)dx
Since v = dx/dt, we can rearrange this as:
dW = m(dv/dt)(vdt)
This simplifies to:
dW = mvdv
To find the total work done, we integrate both sides from the initial velocity (vᵢ) to the final velocity (v<sub>f</sub>):
∫dW = ∫<sub>vᵢ</sub><sup>v<sub>f</sub></sup> mvdv
This integration yields:
W = ½mv<sub>f</sub>² - ½mvᵢ²
Notice that the right-hand side of the equation is simply the change in kinetic energy:
W = ΔKE
This is the Work-Energy Theorem. It states that the net work done on an object is equal to the change in its kinetic energy.
Applications of the Work-Energy Theorem
The Work-Energy Theorem finds widespread application in various fields of physics and engineering. Here are some notable examples:
1. Projectile Motion:
Consider a projectile launched upwards. Gravity does negative work on the projectile, decreasing its kinetic energy as it rises and converting it into potential energy. As the projectile falls, gravity does positive work, increasing its kinetic energy. The Work-Energy Theorem helps calculate the projectile's velocity at any point during its trajectory without explicitly using kinematic equations.
2. Mechanical Systems:
The theorem is essential in analyzing mechanical systems involving forces, displacements, and changes in speed. For example, in analyzing the motion of a roller coaster, the work done by gravity along the track can be directly linked to the changes in the coaster's kinetic energy. This can be used to determine the coaster's speed at various points along the track.
3. Collisions:
The Work-Energy Theorem proves valuable in understanding collisions. In an inelastic collision, some kinetic energy is lost (converted to heat, sound, etc.). The difference between the initial and final kinetic energies reflects the work done by non-conservative forces during the collision.
4. Friction:
Friction is a non-conservative force that always opposes motion. The work done by friction is always negative, meaning it reduces an object's kinetic energy. The Work-Energy Theorem allows us to calculate the energy loss due to friction, understanding the impact on the object's motion.
5. Power:
Power is the rate at which work is done (Power = Work/Time). Since work is equal to the change in kinetic energy, the power delivered to an object can also be related to its change in kinetic energy over time. This is essential in understanding engine performance and energy consumption in various systems.
Limitations and Considerations
While the Work-Energy Theorem is a powerful tool, it's crucial to acknowledge its limitations:
-
Conservative vs. Non-conservative Forces: The theorem is most straightforward when dealing with conservative forces (like gravity and elastic forces), where work done is path-independent. With non-conservative forces (like friction), the work done depends on the path taken. The theorem still applies but requires careful consideration of all forces involved.
-
Rotational Motion: The simple form of the Work-Energy Theorem presented above applies primarily to translational motion. For rotational motion, a more generalized version involving rotational kinetic energy and torque needs to be employed.
-
Relativistic Effects: At very high speeds (approaching the speed of light), relativistic effects become significant, and the classical Work-Energy Theorem needs modification to account for the changes in mass and energy described by Einstein's theory of relativity.
Conclusion: A Cornerstone of Physics
The Work-Energy Theorem stands as a cornerstone of classical mechanics, providing a powerful and elegant link between work and kinetic energy. Its simplicity belies its profound implications, offering a crucial tool for analyzing a vast range of physical phenomena. By understanding the principles outlined here, one gains a deeper appreciation of the fundamental interplay between force, energy, and motion in the world around us. Its widespread applicability, from analyzing simple projectiles to understanding complex mechanical systems, solidifies its importance in various scientific and engineering disciplines. While limitations exist, particularly when dealing with non-conservative forces and rotational motion, mastering the Work-Energy Theorem provides a crucial foundation for advanced studies in physics and related fields. Its versatility and relative simplicity make it an indispensable tool for any aspiring physicist or engineer.
Latest Posts
Latest Posts
-
How Much Is 185 Pounds In Kilos
May 10, 2025
-
What Is The Purpose Of A Spectroscope
May 10, 2025
-
How Are Force And Mass Related To Acceleration
May 10, 2025
-
What Is The Gcf Of 24 And 18
May 10, 2025
-
Y 1 2x 2 3 In Standard Form
May 10, 2025
Related Post
Thank you for visiting our website which covers about Work Equals Change In Kinetic Energy . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.