What's The Square Root Of 160
listenit
Apr 05, 2025 · 5 min read
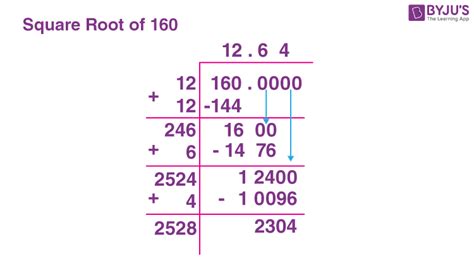
Table of Contents
What's the Square Root of 160? A Deep Dive into Square Roots and Simplification
The question, "What's the square root of 160?" might seem simple at first glance. A quick calculator search will give you a decimal approximation. But understanding the mathematics behind finding the square root, especially for non-perfect squares like 160, reveals a deeper appreciation for numerical manipulation and algebraic concepts. This article will explore not just the answer, but the process, offering a comprehensive guide to understanding square roots and simplifying radical expressions.
Understanding Square Roots
Before we tackle the square root of 160, let's establish a foundational understanding of what a square root actually is. The square root of a number is a value that, when multiplied by itself, equals the original number. For example:
- The square root of 9 (√9) is 3, because 3 x 3 = 9.
- The square root of 25 (√25) is 5, because 5 x 5 = 25.
These are examples of perfect squares, numbers that have whole number square roots. However, many numbers don't have whole number square roots. 160 falls into this category. This is where simplification comes in.
Finding the Square Root of 160: The Simplification Process
160 is not a perfect square. Therefore, its square root will be an irrational number – a number that cannot be expressed as a simple fraction. Instead of a precise decimal value, we aim for a simplified radical form. This involves finding the largest perfect square that is a factor of 160.
Let's break down 160 into its prime factors:
160 = 2 x 80 = 2 x 2 x 40 = 2 x 2 x 2 x 20 = 2 x 2 x 2 x 2 x 10 = 2 x 2 x 2 x 2 x 2 x 5 = 2<sup>5</sup> x 5
Notice that we have five factors of 2. We can rewrite this as:
160 = 2<sup>4</sup> x 2 x 5
Since 2<sup>4</sup> (or 16) is a perfect square (4 x 4 = 16), we can simplify the radical:
√160 = √(2<sup>4</sup> x 2 x 5) = √(2<sup>4</sup>) x √(2 x 5) = 4√10
Therefore, the simplified radical form of the square root of 160 is 4√10.
Decimal Approximation and the Calculator
While 4√10 is the precise, simplified form, you might need a decimal approximation for practical applications. Using a calculator, you'll find that:
√160 ≈ 12.649
Keep in mind that this is an approximation. The actual value of √160 is an irrational number with infinitely many non-repeating digits after the decimal point.
Why Simplify Radicals?
Simplifying radicals, as shown with the square root of 160, is crucial for several reasons:
- Precision: The simplified form (4√10) represents the exact value, unlike the decimal approximation which is inherently imprecise.
- Efficiency: Simplified radicals are often easier to work with in algebraic manipulations and calculations. Imagine trying to multiply or divide two lengthy decimal approximations; working with simplified radicals is far more manageable.
- Understanding: The simplified form provides insight into the number's composition and relationship to perfect squares.
Further Exploration of Square Roots
Understanding the square root of 160 opens the door to more complex concepts within mathematics:
Higher-Order Roots
Square roots are second-order roots. There are also cube roots (third-order roots), fourth roots, and so on. A cube root of a number is a value that, when multiplied by itself three times, equals the original number. Similarly, a fourth root is a value that, when multiplied by itself four times, equals the original number, and so on.
Imaginary Numbers
The square root of a negative number is not a real number. It falls into the realm of imaginary numbers, represented by the letter i. For example, √-1 = i. Imaginary numbers are essential in advanced mathematics, physics, and engineering.
Applications of Square Roots
Square roots find applications in numerous fields:
- Geometry: Calculating the lengths of sides in right-angled triangles using the Pythagorean theorem (a² + b² = c²) relies heavily on square roots.
- Physics: Many physical formulas, especially those involving distance, velocity, and acceleration, incorporate square roots.
- Engineering: Square roots are used in structural calculations, circuit analysis, and signal processing.
- Finance: Calculations related to compound interest and investment returns often involve square roots.
Practical Exercises: Testing your Understanding
To solidify your understanding, try simplifying the following square roots using the method described above:
- √128
- √288
- √108
- √72
- √75
By finding the largest perfect square factor of each number and simplifying, you'll reinforce your skills in working with radical expressions. Remember to express your answers in simplified radical form before resorting to a decimal approximation.
Conclusion: Mastering Square Roots
The seemingly simple question, "What's the square root of 160?", leads us on a journey into the fascinating world of square roots, radical simplification, and the broader landscape of number theory. By understanding the process of simplifying radicals and the concepts behind square roots, you gain a powerful tool for solving problems across various disciplines and a deeper appreciation for the beauty and precision of mathematics. Remember that consistent practice is key to mastering these concepts, so don't hesitate to explore further and tackle more complex problems. The journey towards mathematical proficiency is a continuous process of learning, exploring, and applying your knowledge.
Latest Posts
Latest Posts
-
A 20 Foot Ladder Is Set Up Against A Building
Apr 06, 2025
-
How Do You Do Shorthand Electron Configuration
Apr 06, 2025
-
Three Less Than Six Times A Number
Apr 06, 2025
-
Mass Of Hydrogen Atom In G
Apr 06, 2025
-
What Is 240 Inches In Feet
Apr 06, 2025
Related Post
Thank you for visiting our website which covers about What's The Square Root Of 160 . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.
