What Percentage Is 4 Out Of 12
listenit
May 25, 2025 · 5 min read
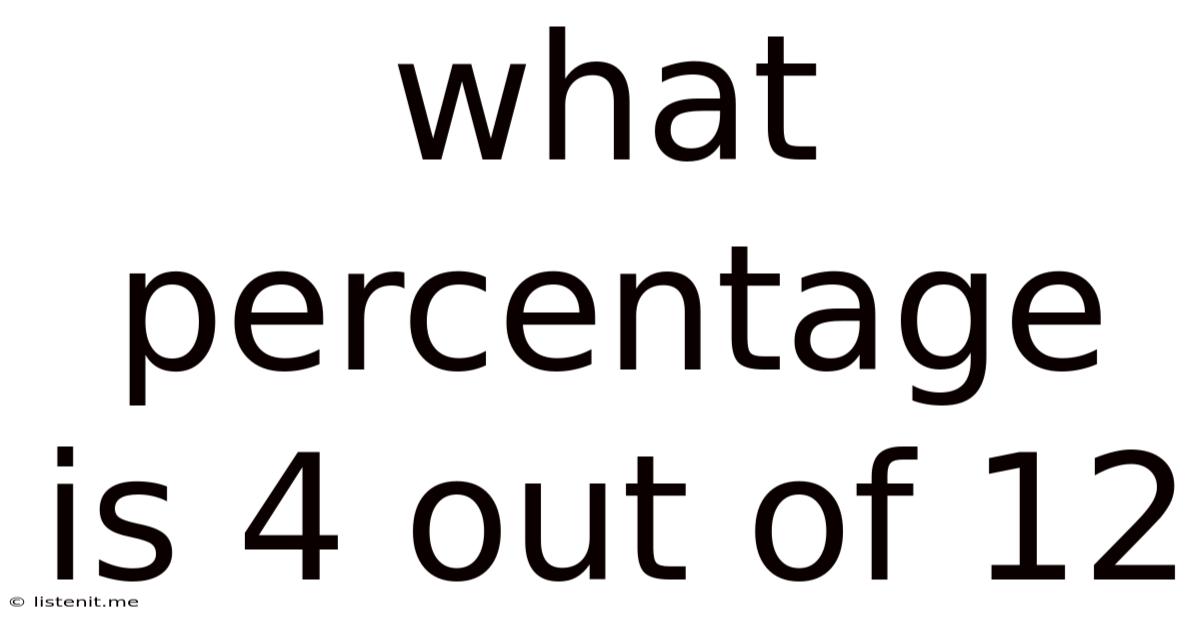
Table of Contents
What Percentage is 4 out of 12? A Comprehensive Guide to Percentage Calculations
Understanding percentages is a fundamental skill in many aspects of life, from calculating discounts and taxes to interpreting data and statistics. This comprehensive guide will delve into the question: "What percentage is 4 out of 12?" We'll not only answer this specific question but also explore the broader concept of percentage calculations, providing you with the tools and knowledge to tackle similar problems with confidence.
Understanding Percentages: The Basics
A percentage is a fraction or ratio expressed as a number out of 100. The symbol "%" represents "per cent," meaning "out of one hundred." Therefore, 50% means 50 out of 100, which can be simplified to 1/2 or 0.5.
Understanding this fundamental concept is crucial for grasping percentage calculations. We often use percentages to represent proportions or parts of a whole. For example, if you score 80% on a test, it means you answered 80 out of 100 questions correctly.
Calculating Percentages: The Formula
The basic formula for calculating a percentage is:
(Part / Whole) * 100% = Percentage
Where:
- Part: Represents the specific portion you're interested in.
- Whole: Represents the total or complete amount.
This formula can be applied to a wide range of scenarios.
Solving "What Percentage is 4 out of 12?"
Now, let's address the central question: What percentage is 4 out of 12?
Using the formula above:
(4 / 12) * 100% = Percentage
First, simplify the fraction 4/12. Both numbers are divisible by 4:
4/12 simplifies to 1/3
Now, calculate the percentage:
(1/3) * 100% ≈ 33.33%
Therefore, 4 out of 12 is approximately 33.33%.
Different Methods for Calculating Percentages
While the basic formula is straightforward, there are several ways to approach percentage calculations, each with its own advantages depending on the context.
Method 1: Using the Basic Formula (as shown above)
This is the most direct and universally applicable method. It’s reliable for any percentage calculation.
Method 2: Simplifying the Fraction First
As demonstrated in the example above, simplifying the fraction before multiplying by 100% can make the calculation easier, especially when dealing with larger numbers.
Method 3: Using a Calculator
Calculators are invaluable tools for percentage calculations, especially when dealing with complex fractions or decimals. Most calculators have a percentage function (%) that simplifies the process. Simply input (4 ÷ 12) x 100 and press the “%” button.
Method 4: Using Proportions
This method is particularly useful for solving problems where you need to find an unknown part or whole. You set up a proportion:
Part / Whole = Percentage / 100
For our example:
4 / 12 = x / 100
Solving for x (the percentage) gives you the same result: x ≈ 33.33%
Practical Applications of Percentage Calculations
Understanding percentage calculations is crucial in various real-world applications:
1. Financial Matters:
- Discounts: Calculating discounts on sale items. For example, a 20% discount on a $100 item means a saving of $20.
- Taxes: Determining the amount of sales tax or income tax owed.
- Interest Rates: Calculating interest earned on savings accounts or interest paid on loans.
- Investment Returns: Assessing the performance of investments.
2. Data Analysis and Statistics:
- Interpreting Survey Results: Understanding the proportion of respondents who chose a particular answer.
- Analyzing Market Trends: Assessing market share and growth rates.
- Calculating Probabilities: Expressing the likelihood of an event occurring.
3. Everyday Life:
- Tip Calculation: Determining the appropriate tip amount in a restaurant.
- Recipe Scaling: Adjusting ingredient quantities in a recipe.
- Grading Systems: Understanding your performance in school based on percentage scores.
Beyond the Basics: Advanced Percentage Calculations
While the basic formula covers most scenarios, some situations require more advanced techniques:
1. Percentage Increase and Decrease:
Calculating percentage changes requires a slightly different approach. The formula for percentage increase is:
[(New Value - Old Value) / Old Value] * 100%
And the formula for percentage decrease is:
[(Old Value - New Value) / Old Value] * 100%
For example, if a price increases from $50 to $60, the percentage increase is:
[(60 - 50) / 50] * 100% = 20%
2. Finding the Original Value:
If you know the percentage and the resulting value after a percentage increase or decrease, you can work backward to find the original value. This often involves using algebraic equations.
3. Percentage Points vs. Percentage Change:
It's crucial to distinguish between percentage points and percentage change. A change of 5 percentage points is different from a 5% change. For instance, if interest rates rise from 2% to 7%, that's a 5-percentage-point increase, but a 250% increase relative to the original rate.
Troubleshooting Common Percentage Calculation Mistakes
Many mistakes arise from misunderstandings of the basic formula or misinterpreting the problem. Here are some common pitfalls:
- Incorrectly identifying the 'part' and the 'whole': Double-check that you've correctly identified the portion you're interested in and the total amount.
- Forgetting to multiply by 100%: The final step of multiplying by 100% is crucial for converting the fraction into a percentage.
- Using the wrong formula for percentage increase/decrease: Make sure you use the appropriate formula for calculating percentage changes.
- Confusing percentage points with percentage change: Clearly understand the distinction between these two concepts.
Conclusion: Mastering Percentage Calculations
Understanding percentages is a versatile and valuable skill applicable across numerous fields. By mastering the basic formula and understanding the various methods of calculation, you can confidently tackle a wide range of percentage problems. This guide has provided you with the tools and knowledge to not only answer "What percentage is 4 out of 12?" but also to solve more complex percentage-related problems in your daily life, academic pursuits, or professional endeavors. Remember to practice regularly and utilize the various techniques discussed to build your proficiency and confidence in handling percentage calculations.
Latest Posts
Latest Posts
-
What Is Ten Percent Of One Million
May 25, 2025
-
What Is The Greatest Common Factor Of 40 And 30
May 25, 2025
-
What Is The Gcf Of 7 And 12
May 25, 2025
-
What Is 27 Out Of 35 As A Percentage
May 25, 2025
-
Greatest Common Factor Of 26 And 65
May 25, 2025
Related Post
Thank you for visiting our website which covers about What Percentage Is 4 Out Of 12 . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.