What Percent Of 50 Is 15
listenit
Apr 05, 2025 · 4 min read
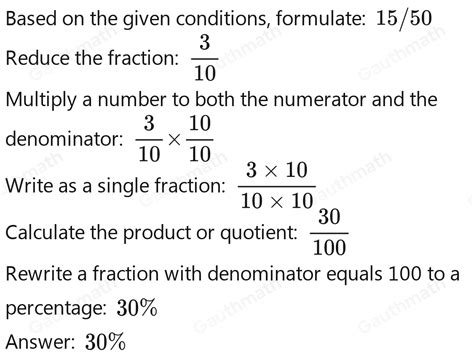
Table of Contents
What Percent of 50 is 15? A Deep Dive into Percentage Calculations
The question, "What percent of 50 is 15?" might seem simple at first glance. It's a fundamental percentage problem that appears frequently in everyday life, from calculating discounts and sales tax to understanding statistics and data analysis. However, understanding the underlying concepts and different methods for solving this problem is crucial for building a strong foundation in mathematics and problem-solving skills. This comprehensive guide will explore various approaches to solve this problem, delve into the theoretical background, and provide practical applications to solidify your understanding.
Understanding Percentages
Before we dive into the calculation, let's refresh our understanding of percentages. A percentage is a fraction or ratio expressed as a number out of 100. The symbol "%" represents "percent," meaning "out of 100." For example, 50% means 50 out of 100, which simplifies to 1/2 or 0.5.
Method 1: Using the Formula
The most direct way to solve "What percent of 50 is 15?" is using the basic percentage formula:
(Part / Whole) x 100 = Percentage
In this case:
- Part: 15 (the value we're interested in)
- Whole: 50 (the total value)
Plugging these values into the formula:
(15 / 50) x 100 = 30%
Therefore, 15 is 30% of 50.
Method 2: Setting up a Proportion
Another effective method involves setting up a proportion. A proportion is an equation stating that two ratios are equal. We can represent the problem as:
15/50 = x/100
Where 'x' represents the unknown percentage. To solve for 'x', we cross-multiply:
15 * 100 = 50 * x 1500 = 50x x = 1500 / 50 x = 30
Again, we find that 15 is 30% of 50.
Method 3: Using Decimal Equivalents
Percentages can be easily converted into decimals by dividing by 100. We can use this approach to solve the problem as follows:
First, express the percentage as a decimal: x/100 = x%
Then set up the equation:
x/100 * 50 = 15
Solve for x:
x = (15 * 100) / 50 x = 30
Hence, 15 is 30% of 50.
Understanding the Context: Real-World Applications
The ability to calculate percentages is essential in numerous real-world scenarios. Let's explore some practical examples:
-
Sales and Discounts: Imagine a store offers a 30% discount on an item originally priced at $50. Using our knowledge, we can quickly determine that the discount amount is $15 ($50 x 0.30 = $15).
-
Tax Calculations: Understanding percentages is crucial when calculating sales tax. If the sales tax rate is 6%, and you purchase an item for $50, you can easily calculate the tax amount: $50 x 0.06 = $3.
-
Grade Calculations: In education, percentages are used to represent grades. If you score 15 out of 50 points on a quiz, your grade is 30%.
-
Data Analysis: Percentages are frequently used in data analysis and statistics to represent proportions and trends. For example, if a survey shows that 15 out of 50 respondents prefer a particular brand, it indicates a 30% preference rate.
-
Financial Calculations: Percentages are fundamental in finance, used to calculate interest rates, returns on investments, and loan payments.
Expanding the Concept: Variations on the Problem
Let's expand on this core concept by exploring variations of the problem, strengthening our understanding of percentage calculations:
1. What is 30% of 50?
This is a direct application of the percentage formula:
30% of 50 = (30/100) * 50 = 15
2. 15 is what percent of 100?
Using the formula:
(15 / 100) * 100 = 15%
3. 15 is 30% of what number?
Here, we need to rearrange the formula:
(30/100) * x = 15 0.3x = 15 x = 15 / 0.3 x = 50
Advanced Applications and Problem-Solving Strategies
Beyond the basic calculations, understanding percentages becomes more critical when dealing with complex scenarios involving multiple percentages, discounts, and taxes. For instance, calculating the final price of an item after applying a series of discounts or taxes requires a systematic approach.
Example: A shirt is originally priced at $100. A 20% discount is applied, followed by a 5% sales tax on the discounted price. What is the final price?
- Discount: 20% of $100 = $20. Discounted price = $100 - $20 = $80.
- Sales Tax: 5% of $80 = $4.
- Final Price: $80 + $4 = $84.
Troubleshooting Common Errors
When working with percentages, several common errors can occur:
- Incorrect order of operations: Remember to follow the order of operations (PEMDAS/BODMAS).
- Improper decimal placement: Carefully convert percentages to decimals before performing calculations.
- Confusing percentage increase and decrease: Clearly distinguish between adding a percentage (increase) and subtracting a percentage (decrease).
- Rounding errors: Pay attention to significant figures and rounding rules to minimize errors.
Conclusion: Mastering Percentage Calculations
Mastering percentage calculations is a valuable skill with applications in various fields. By understanding the underlying principles, different methods of calculation, and common pitfalls, you can confidently tackle a wide range of percentage problems. Practice is key to solidifying your understanding, enabling you to quickly and accurately solve percentage problems in any context, from everyday financial transactions to complex data analysis. Remember to always check your work to minimize errors and ensure accuracy in your calculations. The seemingly simple question, "What percent of 50 is 15?" serves as a gateway to understanding a powerful and widely applicable mathematical concept.
Latest Posts
Latest Posts
-
Is Pi Over 2 Rational Or Irrational
Apr 06, 2025
-
Period 2 On The Periodic Table
Apr 06, 2025
-
Y 2x 3 Solve For Y
Apr 06, 2025
-
Photosynthesis Takes Place In Which Organelle
Apr 06, 2025
-
Daughter Cells Produced In Meiosis Are Identical
Apr 06, 2025
Related Post
Thank you for visiting our website which covers about What Percent Of 50 Is 15 . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.
