What Percent Is Equivalent To 1/25
listenit
May 25, 2025 · 4 min read
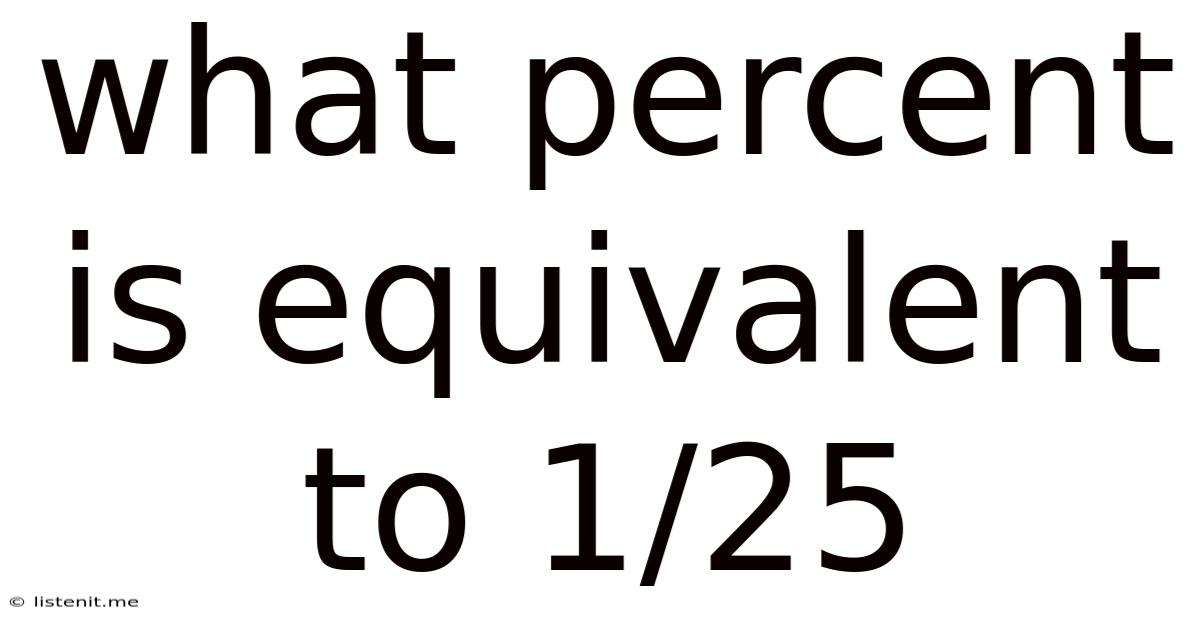
Table of Contents
What Percent is Equivalent to 1/25? A Deep Dive into Fractions, Decimals, and Percentages
The seemingly simple question, "What percent is equivalent to 1/25?", opens a door to a broader understanding of mathematical conversions and their practical applications. While the answer itself is straightforward, exploring the process reveals fundamental concepts crucial for various fields, from finance and statistics to everyday calculations. This article will not only answer the question directly but also delve into the underlying principles, providing you with a comprehensive understanding of fraction-to-percentage conversions and their significance.
Understanding the Basics: Fractions, Decimals, and Percentages
Before tackling the specific conversion, let's solidify our understanding of the three core concepts: fractions, decimals, and percentages.
Fractions: Representing Parts of a Whole
A fraction represents a part of a whole. It's expressed as a ratio of two numbers, the numerator (top number) and the denominator (bottom number). The numerator indicates the number of parts we have, while the denominator indicates the total number of equal parts the whole is divided into. For example, in the fraction 1/25, 1 represents the part we're interested in, and 25 represents the total number of equal parts.
Decimals: Expressing Fractions in Base 10
Decimals are another way to represent parts of a whole. They use the base-10 system, where each digit to the right of the decimal point represents a decreasing power of 10 (tenths, hundredths, thousandths, etc.). Decimals are particularly useful for calculations and comparisons.
Percentages: Expressing Fractions as Parts of 100
A percentage represents a fraction where the denominator is always 100. It indicates how many parts out of 100 we have. The percentage symbol "%" is used to denote a percentage. Percentages are widely used to express proportions, rates, and changes.
Converting 1/25 to a Percentage: The Step-by-Step Process
Now, let's address the core question: what percent is equivalent to 1/25? There are two main approaches:
Method 1: Converting the Fraction to a Decimal, Then to a Percentage
-
Convert the fraction to a decimal: To do this, divide the numerator (1) by the denominator (25): 1 ÷ 25 = 0.04
-
Convert the decimal to a percentage: Multiply the decimal by 100 and add the percentage symbol: 0.04 × 100 = 4%
Therefore, 1/25 is equivalent to 4%.
Method 2: Direct Conversion using Proportions
This method uses the understanding that a percentage is a fraction with a denominator of 100. We set up a proportion:
1/25 = x/100
To solve for x (the percentage), we cross-multiply:
25x = 100
x = 100 ÷ 25
x = 4
Therefore, 1/25 is equivalent to 4%.
Practical Applications of Fraction-to-Percentage Conversions
The ability to convert fractions to percentages is essential in numerous real-world scenarios:
-
Finance: Calculating interest rates, discounts, profit margins, and tax rates often involves converting fractions to percentages. For example, understanding that a 1/25 discount is a 4% discount can help consumers make informed purchasing decisions.
-
Statistics: Percentages are frequently used to represent data and probabilities. Converting fractions to percentages makes it easier to understand and communicate statistical findings. For instance, if 1 out of 25 people prefer a particular product, this represents a 4% preference rate.
-
Science: In scientific experiments, expressing results as percentages often enhances clarity and facilitates comparisons.
-
Everyday Life: Calculating tips, splitting bills, understanding sales, and interpreting survey results all benefit from the ability to convert fractions to percentages effortlessly.
Expanding on the Concept: Working with More Complex Fractions
While 1/25 is a relatively simple fraction, the principles discussed here extend to more complex fractions. Let's consider a few examples:
-
Converting 3/25 to a percentage:
- Convert to a decimal: 3 ÷ 25 = 0.12
- Convert to a percentage: 0.12 × 100 = 12%
-
Converting 7/50 to a percentage:
- Convert to a decimal: 7 ÷ 50 = 0.14
- Convert to a percentage: 0.14 × 100 = 14%
-
Converting a mixed fraction to a percentage:
Let's consider 2 1/4
- Convert the mixed fraction to an improper fraction: (2*4 +1)/4 = 9/4
- Convert to decimal: 9/4 = 2.25
- Convert to percentage: 2.25 * 100 = 225%
Troubleshooting Common Errors
While the conversion process is relatively straightforward, there are some common pitfalls to watch out for:
-
Incorrect division: Ensure you correctly divide the numerator by the denominator when converting a fraction to a decimal.
-
Forgetting to multiply by 100: Remember that to convert a decimal to a percentage, you must multiply by 100.
-
Misinterpreting percentages: Always remember that a percentage represents parts per hundred.
Conclusion: Mastering Fraction-to-Percentage Conversions
The ability to convert fractions to percentages is a fundamental mathematical skill with broad applications. Understanding the underlying principles, as outlined in this article, enables you to tackle various mathematical problems and interpret data effectively. Whether you are navigating financial transactions, analyzing statistical data, or simply solving everyday problems, mastering fraction-to-percentage conversions will empower you with essential numerical literacy. Remember the simple steps involved – divide the numerator by the denominator to get the decimal, then multiply by 100 to obtain the percentage. Practice consistently to build fluency and confidence in your mathematical abilities. By understanding these concepts, you can confidently tackle more complex percentage calculations and effectively apply this knowledge to various real-world scenarios.
Latest Posts
Latest Posts
-
How Many Days Since August 2
May 25, 2025
-
Greatest Common Factor Of 48 And 54
May 25, 2025
-
1978 To Now How Many Years
May 25, 2025
-
How To Measure For Tire Size
May 25, 2025
-
What Grade Is A 76 Out Of 100
May 25, 2025
Related Post
Thank you for visiting our website which covers about What Percent Is Equivalent To 1/25 . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.