What Percent Is 5 Out Of 30
listenit
May 25, 2025 · 4 min read
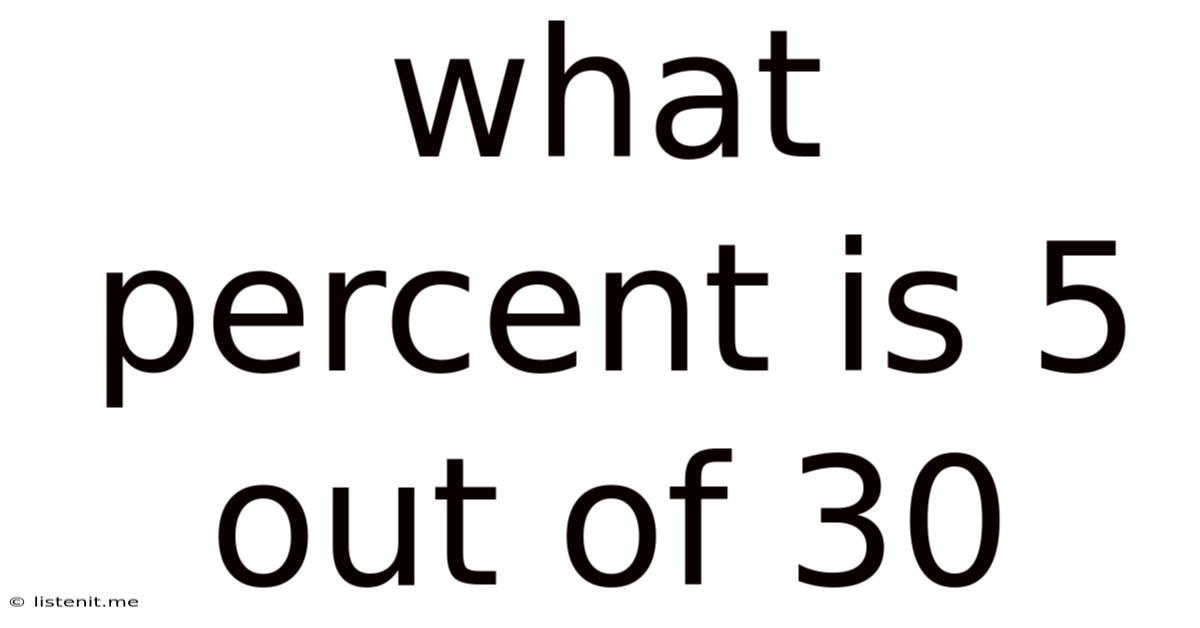
Table of Contents
What Percent is 5 out of 30? A Comprehensive Guide to Percentage Calculations
Understanding percentages is a fundamental skill applicable across various aspects of life, from calculating discounts and taxes to analyzing data and understanding statistics. This comprehensive guide will not only answer the question "What percent is 5 out of 30?" but will also equip you with the knowledge and tools to confidently tackle any percentage calculation.
Understanding Percentages: The Basics
A percentage represents a fraction of 100. The word "percent" literally means "out of 100" (per centum in Latin). Therefore, 50% means 50 out of 100, or 50/100, which simplifies to 1/2. Percentages are a convenient way to express proportions and ratios, making comparisons and interpretations easier.
Calculating Percentages: The Formula
The fundamental formula for calculating percentages is:
(Part / Whole) x 100 = Percentage
Where:
- Part: Represents the specific amount you're interested in expressing as a percentage.
- Whole: Represents the total amount.
- Percentage: The resulting value expressed as a percentage.
Solving "What Percent is 5 out of 30?"
Now, let's apply this formula to answer our main question: "What percent is 5 out of 30?"
In this case:
- Part = 5
- Whole = 30
Using the formula:
(5 / 30) x 100 = Percentage
-
Divide the part by the whole: 5 / 30 = 0.166666...
-
Multiply the result by 100: 0.166666... x 100 = 16.6666...%
-
Round to the desired precision: Rounding to two decimal places, we get 16.67%.
Therefore, 5 out of 30 is 16.67%.
Different Methods for Calculating Percentages
While the fundamental formula is always reliable, several methods can simplify percentage calculations, especially for mental math or quick estimations:
Method 1: Using Fractions
Converting the fraction to its simplest form can sometimes make the calculation easier.
5/30 simplifies to 1/6. To convert 1/6 to a percentage, you can either use the formula (1/6) x 100 = 16.67%, or use the knowledge that 1/6 is approximately 16.67%.
Method 2: Using Proportions
Setting up a proportion can be helpful, especially when dealing with more complex percentage problems. For example:
5/30 = x/100
Cross-multiplying, we get:
30x = 500
x = 500/30 = 16.67%
Method 3: Using a Calculator
Calculators are invaluable for quick and accurate percentage calculations, especially when dealing with larger numbers or more complex calculations involving multiple percentages.
Practical Applications of Percentage Calculations
Understanding percentage calculations is crucial in numerous real-world scenarios. Here are a few examples:
1. Financial Calculations:
- Interest Rates: Calculating interest earned on savings accounts or interest paid on loans.
- Discounts and Sales Tax: Determining the final price of an item after applying discounts or adding sales tax.
- Investment Returns: Measuring the profitability of investments.
- Budgeting: Tracking spending and allocating funds based on percentages of income.
2. Data Analysis and Statistics:
- Statistical Reports: Interpreting data presented as percentages in surveys, research papers, and market analysis.
- Probability: Calculating probabilities expressed as percentages.
- Data Visualization: Representing data visually using pie charts, bar graphs, and other charts that rely on percentages.
3. Academic Assessments:
- Calculating Grades: Determining final grades based on percentage scores on assignments and exams.
- Analyzing Test Results: Understanding student performance based on percentage scores.
4. Everyday Life:
- Tipping: Calculating tips based on a percentage of a restaurant bill.
- Recipe Conversions: Scaling recipes up or down based on percentages.
- Comparing Prices: Evaluating the best deals by comparing prices and discounts expressed as percentages.
Beyond the Basics: More Complex Percentage Problems
While calculating a simple percentage like "what percent is 5 out of 30" is straightforward, more complex scenarios require additional steps. Let's explore a few examples:
1. Finding the Percentage Increase or Decrease:
This involves calculating the percentage change between two values. The formula is:
(New Value - Old Value) / Old Value x 100 = Percentage Change
For example, if the price of an item increased from $20 to $25, the percentage increase is:
(25 - 20) / 20 x 100 = 25%
2. Finding a Percentage of a Number:
This involves calculating a portion of a number based on a given percentage. The formula is:
(Percentage/100) x Number = Result
For example, to find 20% of 150, you would calculate:
(20/100) x 150 = 30
3. Finding the Original Number:
This involves working backward from a percentage to find the original number. This often requires understanding the relationship between the part and the whole.
Mastering Percentages: Tips and Tricks
- Practice Regularly: The best way to master percentage calculations is through consistent practice.
- Understand the Concepts: Focus on understanding the underlying principles rather than just memorizing formulas.
- Use Different Methods: Experiment with different calculation methods to find the approach that suits you best.
- Check Your Work: Always double-check your calculations to ensure accuracy.
- Use Online Resources: Utilize online calculators and tutorials to aid in your learning and practice.
Conclusion
Understanding percentages is a valuable skill with far-reaching applications. By mastering the fundamental formula and exploring different calculation methods, you can confidently tackle various percentage problems, from simple calculations like "What percent is 5 out of 30?" to more complex scenarios involving percentage increases, decreases, and finding original values. Consistent practice and a solid understanding of the underlying concepts will pave the way for proficiency in this essential mathematical skill. Remember to always double-check your work and use various methods to ensure accuracy and build a strong understanding of this fundamental mathematical concept.
Latest Posts
Latest Posts
-
5 6 Divided By 3 4 As A Fraction
May 25, 2025
-
How Fast Is 70 Km An Hour
May 25, 2025
-
What Is The Prime Factorization Of 41
May 25, 2025
-
10 8 Kilometers Per Hour In Miles
May 25, 2025
-
How Many Days Since May 26th
May 25, 2025
Related Post
Thank you for visiting our website which covers about What Percent Is 5 Out Of 30 . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.