What Percent Is 27 Out Of 35
listenit
May 25, 2025 · 5 min read
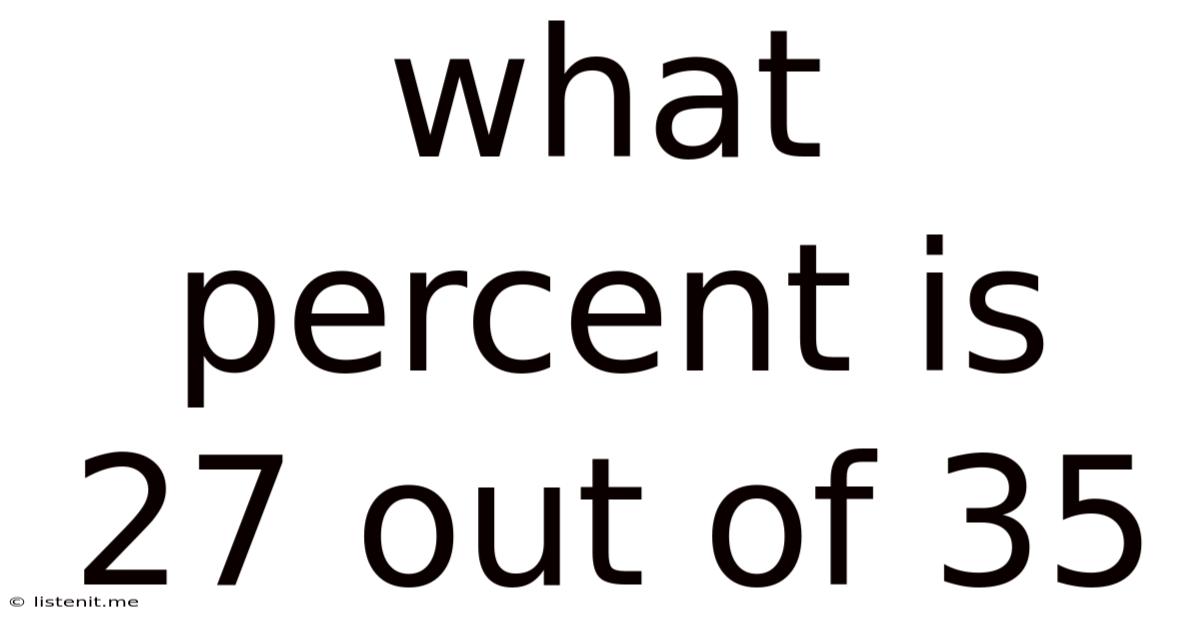
Table of Contents
What Percent is 27 out of 35? A Comprehensive Guide to Percentage Calculations
Determining what percentage 27 represents out of 35 is a fundamental mathematical operation with wide-ranging applications. Whether you're calculating grades, discounts, survey results, or analyzing financial data, understanding how to perform this calculation is crucial. This comprehensive guide will not only show you how to calculate this specific percentage but also equip you with the knowledge and understanding to tackle similar problems with ease.
Understanding Percentages
Before diving into the calculation, let's solidify our understanding of percentages. A percentage is a fraction or ratio expressed as a number out of 100. The symbol "%" represents "per cent," meaning "out of one hundred." Therefore, 50% means 50 out of 100, which is equivalent to ½ or 0.5.
Calculating the Percentage: The Step-by-Step Method
There are several ways to calculate what percent 27 is out of 35. We'll explore the most common and straightforward methods.
Method 1: Using the Formula
The fundamental formula for calculating percentages is:
(Part / Whole) x 100% = Percentage
In our case:
- Part: 27
- Whole: 35
Substituting these values into the formula:
(27 / 35) x 100% = 77.14% (approximately)
Therefore, 27 is approximately 77.14% of 35.
Method 2: Decimal Conversion and Multiplication
This method involves first converting the fraction into a decimal and then multiplying by 100%.
- Divide the part by the whole: 27 / 35 = 0.7714 (approximately)
- Multiply the decimal by 100%: 0.7714 x 100% = 77.14% (approximately)
Method 3: Using Proportions
Proportions offer a slightly different approach to solving percentage problems. We set up a proportion where we know three of the four values and solve for the unknown.
- Let 'x' represent the percentage we want to find.
- We can set up the proportion: 27/35 = x/100
To solve for 'x', we cross-multiply:
35x = 2700
Then, divide both sides by 35:
x = 2700 / 35 = 77.14 (approximately)
Therefore, x = 77.14%
Understanding the Result: 77.14%
The result, 77.14%, signifies that 27 represents approximately 77.14 out of every 100 parts of 35. This percentage provides a clear and concise way to represent the relationship between 27 and 35.
Practical Applications of Percentage Calculations
The ability to calculate percentages accurately is essential in many real-world situations. Here are just a few examples:
1. Academic Performance:
Calculating grades often involves determining the percentage of correct answers out of the total number of questions. For example, if a student answered 27 questions correctly out of 35, their grade would be 77.14%.
2. Financial Calculations:
Percentage calculations are fundamental in finance. Determining interest rates, discounts, taxes, profit margins, and returns on investment all rely heavily on percentage calculations. For instance, a 10% discount on a $35 item would be calculated as 10% of $35, which is $3.50.
3. Data Analysis and Statistics:
Percentages are frequently used to represent data in surveys, polls, and statistical analyses. Representing results as percentages allows for easy comparison and interpretation. For example, if 27 out of 35 respondents preferred a particular product, this could be expressed as a 77.14% preference rate.
4. Sales and Marketing:
Sales figures, conversion rates, and market share are often expressed as percentages. This allows businesses to track their progress, identify trends, and make informed decisions. For example, a 77.14% conversion rate means that 77.14% of website visitors completed a desired action, such as making a purchase.
5. Everyday Life:
Percentage calculations are also useful in everyday situations, such as calculating tips, splitting bills, or understanding sales promotions.
Beyond the Basics: Advanced Percentage Concepts
While calculating "what percent is 27 out of 35" provides a foundational understanding, let's explore some more advanced percentage concepts:
1. Calculating the Percentage Increase or Decrease:
This involves determining the percentage change between two values. The formula is:
[(New Value - Old Value) / Old Value] x 100%
For example, if a product's price increased from $35 to $45, the percentage increase would be:
[(45 - 35) / 35] x 100% = 28.57%
2. Finding the Original Value:
Sometimes, you know the percentage and the resulting value, but you need to find the original value. This requires working backward using the percentage formula.
For instance, if a product is currently priced at $27 after a 20% discount, the original price can be calculated as follows:
Let 'x' be the original price. Then, x - 0.20x = $27
Simplifying: 0.80x = $27
Solving for x: x = $27 / 0.80 = $33.75
The original price was $33.75.
3. Compound Percentages:
Compound percentages involve applying a percentage to a value repeatedly. This is common in compound interest calculations.
Tips for Mastering Percentage Calculations
- Practice regularly: The more you practice, the more confident and proficient you will become.
- Understand the concepts: Don't just memorize formulas; strive to understand the underlying principles.
- Use a calculator: For complex calculations, a calculator can significantly reduce the risk of errors.
- Check your work: Always double-check your calculations to ensure accuracy.
- Utilize online resources: Numerous online resources, including calculators and tutorials, can assist you in learning and practicing percentage calculations.
Conclusion: Mastering the Power of Percentages
Calculating what percent 27 is out of 35, and understanding percentages in general, is a vital skill across various disciplines. From academic achievements to financial planning and data analysis, the ability to perform accurate percentage calculations empowers informed decision-making and problem-solving. By mastering the techniques and concepts discussed in this guide, you can confidently tackle percentage-related problems and unlock the power of percentages in your daily life. Remember to practice regularly, utilize different methods, and check your work to reinforce your understanding and build accuracy. The ability to confidently work with percentages will prove invaluable in many aspects of your personal and professional life.
Latest Posts
Latest Posts
-
What Is Half Of 1 2 In Fraction
May 26, 2025
-
3 Feet Per Second To Mph
May 26, 2025
-
90 Billion Divided By 300 Million
May 26, 2025
-
How Many Hours Is In 10 Days
May 26, 2025
-
How To Find The Measurements Of A Triangle
May 26, 2025
Related Post
Thank you for visiting our website which covers about What Percent Is 27 Out Of 35 . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.