What Percent Is 20 Out Of 23
listenit
May 25, 2025 · 5 min read
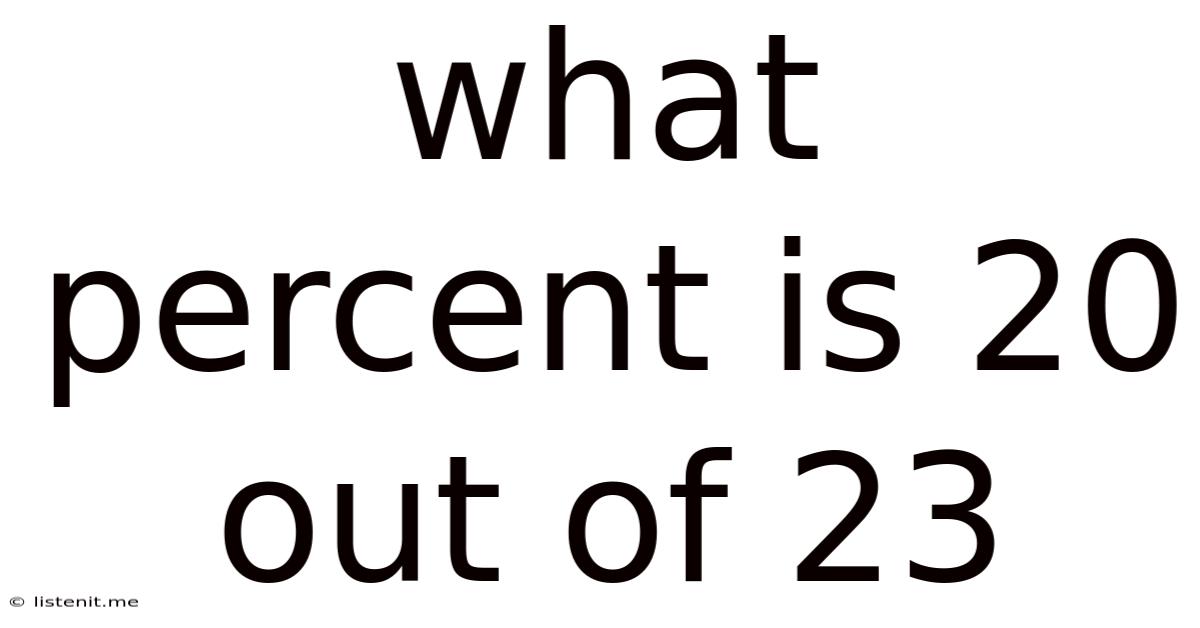
Table of Contents
What Percent is 20 out of 23? A Comprehensive Guide to Percentage Calculations
Determining what percentage 20 represents out of 23 involves a straightforward yet fundamental concept in mathematics: calculating percentages. This seemingly simple calculation has broad applications across various fields, from everyday finances to complex scientific analyses. This comprehensive guide will not only answer the question directly but will also equip you with the skills and understanding to tackle similar percentage problems independently. We'll explore the calculation itself, delve into the underlying principles, and examine practical applications to solidify your understanding.
Understanding Percentages
Before diving into the specific calculation of 20 out of 23, let's establish a clear understanding of percentages. A percentage is simply a fraction expressed as a part of 100. The symbol "%" represents "per hundred" or "out of 100." For example, 50% means 50 out of 100, which is equivalent to the fraction 50/100 or the decimal 0.5.
Calculating the Percentage: 20 out of 23
The core of the problem – "What percent is 20 out of 23?" – translates directly into a mathematical expression:
(20 / 23) * 100%
This formula works as follows:
-
The Fraction: We begin by representing the given values as a fraction. 20 is the part, and 23 is the whole. Therefore, our fraction is 20/23.
-
Converting to Decimal: To express this fraction as a percentage, we need to convert it to a decimal. We do this by dividing the numerator (20) by the denominator (23).
20 ÷ 23 ≈ 0.869565
-
Multiplying by 100: The decimal represents the portion of one. To express it as a percentage (a part of 100), we multiply the decimal by 100.
0.869565 * 100 ≈ 86.9565%
-
Rounding: Percentages are often rounded to a convenient number of decimal places. Rounding to two decimal places, we get:
86.96%
Therefore, 20 out of 23 is approximately 86.96%.
Different Methods for Calculating Percentages
While the above method is the most straightforward, there are alternative approaches to calculate percentages, each offering its own advantages depending on the context and available tools.
Method 2: Using Proportions
Percentages can also be solved using proportions. A proportion is an equation stating that two ratios are equal. We can set up a proportion to solve for the unknown percentage (x):
20/23 = x/100
To solve for x, we cross-multiply:
20 * 100 = 23 * x
2000 = 23x
x = 2000/23 ≈ 86.96%
This method provides a clear visual representation of the relationship between the parts and the whole.
Method 3: Using a Calculator or Spreadsheet Software
Modern calculators and spreadsheet software (like Microsoft Excel or Google Sheets) have built-in functions to calculate percentages effortlessly. These tools can significantly speed up the process, especially when dealing with numerous calculations. The exact function may vary depending on the software used, but it usually involves entering the fraction (20/23) and using a percentage function or operator.
Practical Applications of Percentage Calculations
The ability to calculate percentages is crucial across a wide range of disciplines and everyday life situations. Here are some examples:
-
Finance: Calculating interest rates, discounts, taxes, profit margins, and investment returns all rely on percentage calculations. Understanding percentages is essential for effective financial planning and decision-making. For instance, calculating the percentage of a discount on a sale item or determining the interest earned on a savings account both require percentage calculations.
-
Science: Percentages are frequently used in scientific research to express data, such as the percentage of a population exhibiting a particular trait or the percentage change in a measured variable over time. Statistics often relies heavily on percentages to present and analyze data effectively.
-
Education: Grade calculation, exam scores, and performance metrics in education often employ percentage calculations to evaluate student performance and progress.
-
Business: Companies use percentages to track sales growth, market share, customer satisfaction, and other key performance indicators (KPIs). Understanding percentages is crucial for strategic business decisions.
-
Everyday Life: Calculating tips in restaurants, determining the percentage of ingredients in a recipe, or understanding sales discounts at stores all involve percentage calculations.
Expanding on the Concept: Understanding Ratios and Proportions
The calculation of "what percent is 20 out of 23" is fundamentally about understanding ratios and proportions. A ratio is a comparison of two quantities, often expressed as a fraction. In this case, the ratio is 20:23. A proportion is an equation that states two ratios are equal. This is exactly what we used in Method 2 above, demonstrating the interconnectedness of these mathematical concepts.
Troubleshooting Common Percentage Calculation Errors
While percentage calculations are relatively straightforward, some common errors can occur:
-
Incorrect Order of Operations: When using calculators, ensure you follow the correct order of operations (PEMDAS/BODMAS). Dividing before multiplying is crucial in this calculation.
-
Misinterpretation of the Whole: Clearly identifying the "whole" value (the denominator in the fraction) is essential. Errors can occur if the wrong value is used as the denominator.
-
Rounding Errors: Rounding too aggressively early in the calculation can lead to inaccuracies in the final result. It's generally best to round only at the very end.
-
Incorrect Use of Percentage Symbol: Remember to include the "%" symbol after the final calculated value to correctly express it as a percentage.
Conclusion: Mastering Percentage Calculations
Understanding how to calculate percentages is a valuable skill with widespread applications. The seemingly simple problem of determining "what percent is 20 out of 23" provides a springboard for learning about ratios, proportions, and the broader significance of percentage calculations in various aspects of life and work. By mastering this fundamental mathematical concept, you'll enhance your problem-solving skills and improve your ability to interpret and analyze data effectively. Remember to practice regularly, utilizing different methods, and always double-check your work to ensure accuracy. The more you practice, the more confident and proficient you will become in handling percentage calculations.
Latest Posts
Latest Posts
-
What Is The Prime Factorization Of 93
May 25, 2025
-
Greatest Common Factor 8 And 10
May 25, 2025
-
How Many Days Since Nov 30
May 25, 2025
-
What Is The Greatest Common Factor Of 56 And 84
May 25, 2025
-
2 To The Power Of 41
May 25, 2025
Related Post
Thank you for visiting our website which covers about What Percent Is 20 Out Of 23 . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.