What Is The Percent Of 6/10
listenit
May 25, 2025 · 5 min read
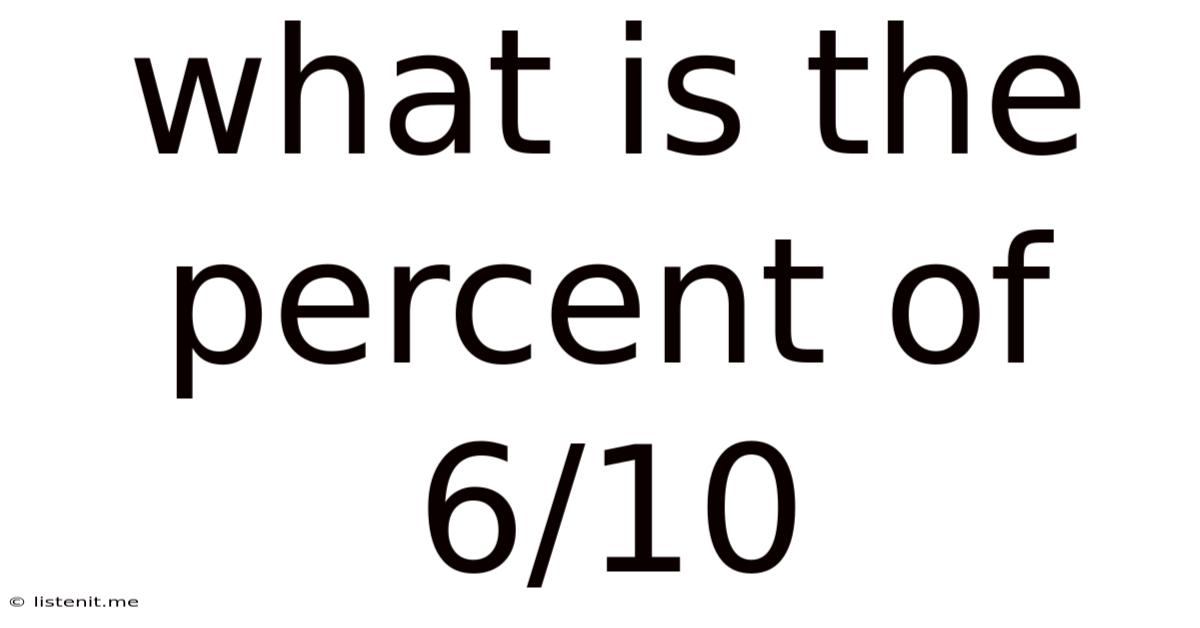
Table of Contents
What is the Percent of 6/10? A Comprehensive Guide to Fractions, Decimals, and Percentages
Understanding fractions, decimals, and percentages is fundamental to numerous aspects of life, from calculating discounts in a shop to understanding financial reports. This comprehensive guide delves into the intricacies of converting fractions to percentages, using the example of 6/10, and explores related concepts to solidify your understanding.
Understanding Fractions
A fraction represents a part of a whole. It's expressed as a ratio of two numbers: the numerator (top number) and the denominator (bottom number). In the fraction 6/10, 6 is the numerator and 10 is the denominator. This means we are considering 6 parts out of a total of 10 equal parts.
Types of Fractions
- Proper Fractions: The numerator is smaller than the denominator (e.g., 6/10, 1/2, 3/4).
- Improper Fractions: The numerator is equal to or larger than the denominator (e.g., 10/6, 5/2, 7/4). These represent a value greater than or equal to 1.
- Mixed Numbers: Combine a whole number and a proper fraction (e.g., 1 1/2, 2 3/4). These are equivalent to improper fractions.
Converting Fractions to Decimals
To convert a fraction to a decimal, you divide the numerator by the denominator. In our example:
6 ÷ 10 = 0.6
Therefore, the decimal equivalent of 6/10 is 0.6. This signifies that 6/10 represents six-tenths.
Converting Decimals to Percentages
Percentages express a fraction or decimal as a proportion of 100. To convert a decimal to a percentage, multiply the decimal by 100 and add the percent symbol (%).
0.6 x 100 = 60%
Therefore, 6/10 is equal to 60%.
Different Methods for Calculating Percentage
While the method above is straightforward, understanding alternative approaches can enhance your problem-solving skills and adaptability to various scenarios. Here are a few other methods for calculating percentages:
Method 1: Using Proportions
A proportion equates two ratios. To find the percentage, set up a proportion:
- Part/Whole = Percentage/100
In our case:
- 6/10 = x/100
Solving for 'x' (the percentage):
- 10x = 600
- x = 60
Therefore, 6/10 is 60%. This method is especially useful for mentally calculating percentages of larger numbers.
Method 2: Using the Decimal Method (as shown above)
This method involves first converting the fraction to a decimal and then multiplying by 100 to find the percentage. It's a quick and efficient method for most scenarios.
Method 3: Using Simplification
Simplifying the fraction before conversion can sometimes make the calculation easier. 6/10 simplifies to 3/5. Converting 3/5 to a decimal:
3 ÷ 5 = 0.6
Then, multiplying by 100:
0.6 x 100 = 60%
This highlights that simplifying fractions can streamline the percentage calculation process.
Practical Applications of Percentage Calculations
The ability to convert fractions to percentages is crucial in numerous real-world situations. Here are a few examples:
- Calculating Grades: If you answered 6 out of 10 questions correctly on a test, your score is 60%.
- Discounts and Sales: A 60% discount means you save 60% of the original price.
- Financial Calculations: Understanding percentages is essential for calculating interest rates, taxes, and investment returns.
- Data Analysis: Percentages are frequently used to represent proportions in data analysis and statistical reports.
- Probability and Statistics: Percentages play a vital role in expressing probabilities and interpreting statistical data.
Beyond 6/10: Exploring Other Fractions and Percentages
The principles outlined above can be applied to any fraction. Let's explore a few more examples:
- 1/2: This simplifies to 50%. Dividing 1 by 2 gives 0.5, which multiplied by 100 equals 50%.
- 3/4: This equals 75%. Dividing 3 by 4 gives 0.75, and multiplying by 100 results in 75%.
- 1/4: This simplifies to 25%. Dividing 1 by 4 gives 0.25, resulting in 25% when multiplied by 100.
- 2/3: This fraction is approximately 66.67%. Dividing 2 by 3 gives 0.6666..., which rounds to 0.67 and then to 66.67%.
These examples illustrate the versatile nature of fraction-to-percentage conversions.
Common Mistakes to Avoid
While the process is relatively straightforward, some common mistakes can lead to incorrect calculations. These include:
- Incorrect order of operations: Always divide the numerator by the denominator before multiplying by 100.
- Forgetting the percentage symbol: Always remember to include the "%" symbol to indicate that the number represents a percentage.
- Rounding errors: Be mindful of rounding errors, particularly when dealing with fractions that result in repeating decimals. Consider the context of the problem and determine an appropriate level of precision.
Mastering Percentages: Tips and Tricks
To become proficient in percentage calculations:
- Practice regularly: Consistent practice is key to mastering any mathematical concept.
- Use visual aids: Diagrams and charts can aid in understanding fractions and percentages.
- Utilize online calculators: Online calculators can verify your calculations and provide quick answers.
- Break down complex problems: Decompose complex problems into smaller, more manageable parts.
- Understand the underlying concepts: A strong grasp of fractions and decimals is crucial for working with percentages.
Conclusion: The Significance of Understanding Percentages
In conclusion, understanding how to convert fractions like 6/10 to percentages (60%) is a vital skill applicable across numerous disciplines. By mastering the techniques outlined in this guide, you'll enhance your problem-solving abilities and confidently tackle percentage-related challenges in various contexts, from academic pursuits to professional endeavors. Remember the steps, practice regularly, and you'll soon become proficient in this fundamental mathematical skill.
Latest Posts
Latest Posts
-
What Month Is 9 Months Before July
May 25, 2025
-
What Is 1 3 Of 1200
May 25, 2025
-
75 Out Of 80 As A Percentage
May 25, 2025
-
11 Out Of 12 In Percentage
May 25, 2025
-
What Is The Least Common Multiple Of 24 And 12
May 25, 2025
Related Post
Thank you for visiting our website which covers about What Is The Percent Of 6/10 . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.