What Is The Opposite Of 1 2
listenit
May 10, 2025 · 5 min read
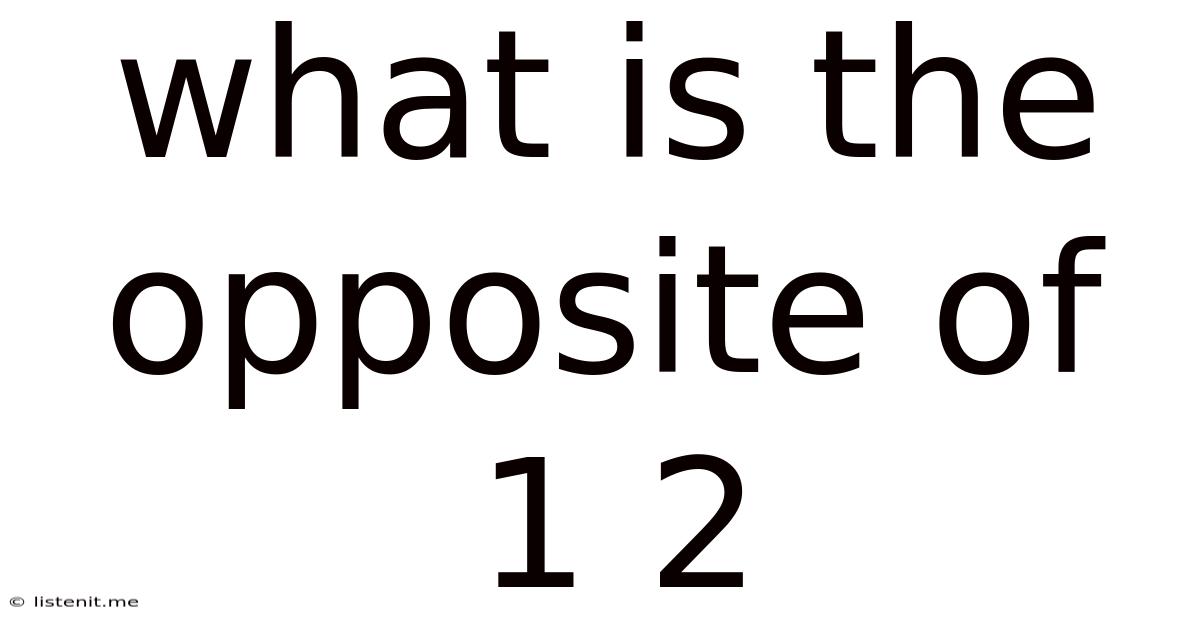
Table of Contents
What is the Opposite of 1, 2? Exploring Opposites in Different Contexts
The question "What is the opposite of 1, 2?" doesn't have a single, straightforward answer. The concept of "opposite" depends heavily on the context in which the numbers 1 and 2 are presented. To understand this, we need to explore several possible interpretations and their corresponding opposites.
1. Opposites in Numerical Order: The Concept of Negative Numbers
If we consider 1 and 2 as positive integers in a numerical sequence, a simple interpretation of their opposite would involve negative numbers. The opposite of 1 would be -1, and the opposite of 2 would be -2. This is based on the number line, where positive and negative numbers are symmetrically positioned around zero. Moving from 1 to -1, or 2 to -2, involves a reflection across the zero point.
Key Considerations:
- Magnitude vs. Sign: The opposite number retains the same magnitude (distance from zero) but changes its sign. The magnitude of 1 and -1 is the same: 1.
- Additive Inverse: In mathematics, the opposite of a number is also known as its additive inverse. Adding a number to its additive inverse always results in zero (e.g., 1 + (-1) = 0).
- Applications: This concept of opposites is crucial in various mathematical operations, including subtraction, solving equations, and working with vectors.
2. Opposites in Sequential Order: Predecessors and Successors
Another interpretation views 1 and 2 as positions within a sequence. In this case, the "opposite" might refer to the element immediately preceding or succeeding them.
- Predecessor: The opposite of 1 (considering its predecessor) could be argued as 0, although this breaks the pattern in a numerical sense. There isn’t a number before 1 in the set of Natural numbers.
- Successor: The opposite of 2 (considering its successor) is 3. Again, this is not a strict opposite in the mathematical sense, but an opposite in terms of positional relation within a sequence.
Key Considerations:
- Context Dependency: This interpretation relies heavily on the specific sequence being examined. The "opposite" changes if we're considering the sequence of even numbers, odd numbers, Fibonacci sequence, or any other specific series.
- Lack of Mathematical Rigor: This definition of "opposite" is less mathematically formal than the additive inverse.
3. Opposites in Symbolic Representation: Binary Opposites
The numbers 1 and 2 can be seen as symbolic representations. For example, in some systems (e.g., binary logic), 1 might represent 'true' and 0 might represent 'false'. While 2 doesn't have a direct equivalent in simple binary, extending the logic, we might explore the relationship of 1 and 2 in Boolean algebra or other systems.
- Binary Opposition: In literature and semiotics, binary opposition is a fundamental concept. It describes the contrasting relationship between two concepts which are mutually exclusive. While 1 and 2 aren't directly opposite in this scheme, we can extend it. For example, if 1 represents "on" and 2 represents "off" (a simplified system), their opposites would be "off" and "on" respectively. This again requires a predefined system for the interpretation to be meaningful.
Key Considerations:
- System-Specific: The interpretation of the opposite strongly depends on the symbolic system adopted. Different systems will lead to different answers.
- Extensibility: The concept can be extended to other systems beyond binary such as ternary, quaternary, or even more complex systems of symbolic representation.
4. Opposites in Set Theory: Complements and Subsets
In set theory, we can think of 1 and 2 as elements of a larger set. The concept of "opposite" might refer to the complement of a set or the relationship between subsets.
- Universal Set: If we consider a universal set U, and subsets containing 1 and 2, then the complement of these subsets could be considered an "opposite" but requires definition of the universal set.
- Subsets: If we have a set containing more elements, the opposite could imply a subset that does not include 1 and 2, but this also depends on the context of the set.
Key Considerations:
- Contextual Definition: The concept of "opposite" here strongly depends on the specific sets and their relationship within a larger universal set.
- Multiple Interpretations: The interpretation of "opposite" isn't unique and changes based on the definition of the sets involved.
5. Opposites in a Philosophical Sense: Duality and Contradiction
Moving beyond mathematical contexts, the question of opposites can be considered philosophically. The numbers 1 and 2 themselves represent a duality – two distinct entities. The opposite might refer to the concept of unity or singularity.
- Unity vs. Duality: The opposite of the plurality suggested by 1 and 2 could be seen as the concept of singularity or oneness – a single, unified entity.
- Contradiction: The concept of "opposite" might also be interpreted as a contradiction or a negation of the given numbers in a philosophical or logical sense. This would require a more abstract and context-dependent understanding.
Key Considerations:
- Abstract Nature: This interpretation delves into abstract philosophical concepts rather than concrete mathematical definitions.
- Subjective Interpretation: The "opposite" in this context will be influenced by individual philosophical perspectives.
Conclusion: The Importance of Context
In conclusion, there isn't a single definitive answer to the question "What is the opposite of 1, 2?". The concept of "opposite" is highly context-dependent. The answer varies depending on whether we consider numerical order, sequential order, symbolic representation, set theory, or even philosophical interpretations. Therefore, before determining the opposite of any numbers or concepts, careful consideration of the underlying context and the specific definition of "opposite" being employed is paramount. This ambiguity highlights the importance of clarity and precision in defining terms and establishing a common framework of understanding. The exploration of this seemingly simple question reveals a fascinating journey into the nuances of mathematics, logic, and philosophy.
Latest Posts
Latest Posts
-
Find The Gcf Of 28 And 42
May 10, 2025
-
What Is 25 Off Of 250
May 10, 2025
-
Eight Less Than A Number N Is At Least 10
May 10, 2025
-
Why Is Fresh Water A Limited Resource
May 10, 2025
-
The Transfer Of Heat Through Electromagnetic Waves
May 10, 2025
Related Post
Thank you for visiting our website which covers about What Is The Opposite Of 1 2 . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.