What Is The Mixed Number For 7/5
listenit
May 12, 2025 · 5 min read
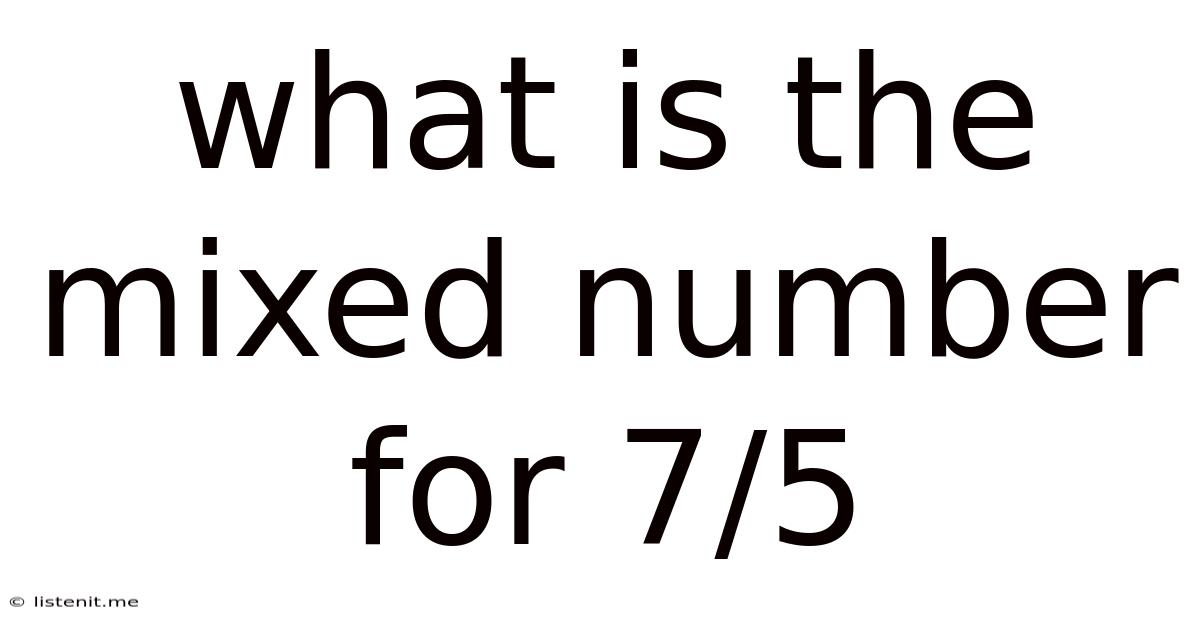
Table of Contents
What is the Mixed Number for 7/5? A Deep Dive into Fraction Conversion
Understanding fractions is a cornerstone of mathematics, vital for various applications from baking to advanced calculus. This article will thoroughly explore the process of converting an improper fraction, specifically 7/5, into its mixed number equivalent. We'll not only show how to do it, but also why the process works, delving into the underlying mathematical concepts and providing various examples to solidify your understanding. We'll also explore the broader context of fraction manipulation, touching upon applications and practical uses.
Understanding Fractions: A Quick Refresher
Before we dive into converting 7/5, let's quickly review the basics of fractions. A fraction represents a part of a whole. It consists of two main components:
- Numerator: The top number, indicating how many parts you have.
- Denominator: The bottom number, indicating how many equal parts the whole is divided into.
For example, in the fraction 3/4, the numerator (3) represents three parts, and the denominator (4) means the whole is divided into four equal parts.
Proper vs. Improper Fractions
Fractions are broadly categorized into two types:
-
Proper Fractions: A proper fraction has a numerator that is smaller than its denominator. For example, 1/2, 3/4, and 5/8 are proper fractions. They represent a value less than one whole.
-
Improper Fractions: An improper fraction has a numerator that is equal to or greater than its denominator. Examples include 7/5, 9/4, and 5/5. These represent a value equal to or greater than one whole.
Our focus is on improper fractions, specifically 7/5, which is an improper fraction because the numerator (7) is greater than the denominator (5).
Converting 7/5 to a Mixed Number
A mixed number combines a whole number and a proper fraction. Converting an improper fraction like 7/5 into a mixed number involves determining how many whole units are contained within the fraction and expressing the remaining portion as a proper fraction.
Here's how to convert 7/5:
-
Division: Divide the numerator (7) by the denominator (5). 7 ÷ 5 = 1 with a remainder of 2.
-
Whole Number: The quotient (1) becomes the whole number part of the mixed number. This represents the number of whole units contained in the improper fraction.
-
Proper Fraction: The remainder (2) becomes the numerator of the proper fraction, and the original denominator (5) remains the denominator. This gives us the proper fraction 2/5.
-
Mixed Number: Combine the whole number and the proper fraction to form the mixed number: 1 2/5.
Therefore, the mixed number equivalent of 7/5 is 1 2/5. This means that 7/5 represents one whole and two-fifths more.
Visualizing the Conversion: The Power of Representation
Imagine you have seven slices of pizza, and each pizza is cut into five equal slices. You can assemble one complete pizza (5 slices) and have two slices remaining. This visually represents the conversion of 7/5 to 1 2/5. The one whole pizza represents the whole number '1', and the remaining two slices out of five represent the fraction 2/5.
Practical Applications: Where do Mixed Numbers Shine?
Mixed numbers are incredibly useful in various real-world scenarios, particularly when dealing with quantities that are larger than one whole unit. Here are some examples:
-
Measurement: Imagine measuring ingredients for a recipe. If a recipe calls for 7/5 cups of flour, it's easier to understand and measure 1 2/5 cups.
-
Construction: In construction projects, dimensions are often expressed using mixed numbers. For example, a board might measure 2 3/4 feet long.
-
Time: Time is often expressed using mixed numbers. For instance, you might run for 1 1/2 hours.
-
Everyday Life: Many daily situations involve quantities that are not whole numbers, requiring the use of mixed numbers for precise representation.
Beyond 7/5: Converting Other Improper Fractions
The method described above for converting 7/5 to a mixed number applies to any improper fraction. Let's consider a few more examples:
-
11/3: 11 ÷ 3 = 3 with a remainder of 2. Therefore, 11/3 = 3 2/3.
-
17/6: 17 ÷ 6 = 2 with a remainder of 5. Therefore, 17/6 = 2 5/6.
-
22/7: 22 ÷ 7 = 3 with a remainder of 1. Therefore, 22/7 = 3 1/7.
Converting Mixed Numbers to Improper Fractions: The Reverse Process
It's equally important to understand the reverse process – converting a mixed number back into an improper fraction. This is often necessary in mathematical calculations. Here's how:
-
Multiply: Multiply the whole number by the denominator of the fraction.
-
Add: Add the result from step 1 to the numerator of the fraction.
-
Keep the Denominator: The denominator remains unchanged.
Let's convert 1 2/5 back into an improper fraction:
-
1 * 5 = 5
-
5 + 2 = 7
-
The denominator remains 5.
Therefore, 1 2/5 = 7/5. This confirms our initial conversion.
Simplifying Fractions: A Crucial Step
Once you have converted an improper fraction to a mixed number, it's often necessary to simplify the resulting fraction to its lowest terms. This makes the fraction easier to understand and work with. Simplification involves dividing both the numerator and the denominator by their greatest common divisor (GCD). For example, if you end up with a fraction like 6/12, you can simplify this to 1/2 by dividing both the numerator and denominator by 6 (their GCD).
Conclusion: Mastering Fraction Conversions
Converting improper fractions to mixed numbers is a fundamental skill in mathematics with far-reaching applications. Understanding this process, along with the ability to perform the reverse conversion, is essential for anyone working with fractions. Through practice and a clear grasp of the underlying concepts, you can confidently navigate the world of fractions and their various forms. Remember, consistent practice and visual aids can significantly enhance your understanding and mastery of this important mathematical concept. By applying these techniques and focusing on the practical applications of mixed numbers, you will strengthen your mathematical proficiency and broaden your problem-solving abilities.
Latest Posts
Related Post
Thank you for visiting our website which covers about What Is The Mixed Number For 7/5 . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.