What Is The Gcf Of 22 And 33
listenit
May 23, 2025 · 5 min read
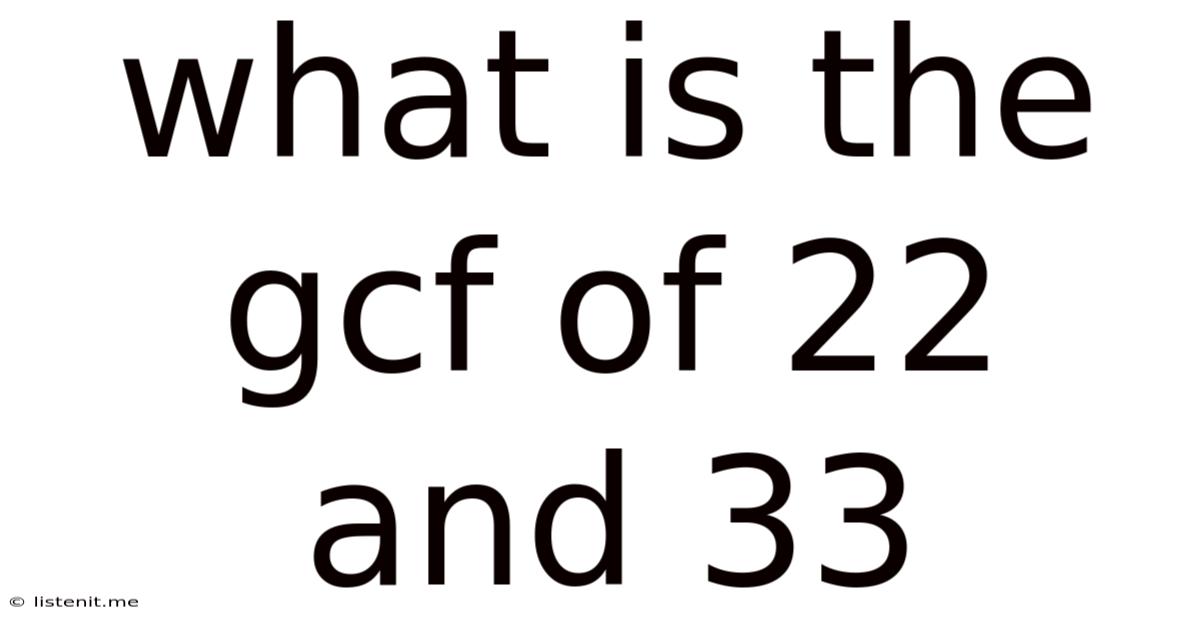
Table of Contents
What is the GCF of 22 and 33? A Deep Dive into Greatest Common Factors
Finding the greatest common factor (GCF) of two numbers might seem like a simple arithmetic task, but understanding the underlying concepts and different methods for calculating it can be surprisingly insightful. This article will explore the GCF of 22 and 33 in detail, explaining the various approaches and expanding on the broader implications of GCF in mathematics and beyond. We'll move beyond simply stating the answer and delve into the "why" and "how," making this a comprehensive guide for students, educators, and anyone curious about number theory.
Understanding Greatest Common Factors (GCF)
The greatest common factor (GCF), also known as the greatest common divisor (GCD), is the largest positive integer that divides each of the integers without leaving a remainder. In simpler terms, it's the biggest number that perfectly divides both numbers. This concept is fundamental in simplifying fractions, solving algebraic equations, and understanding the relationships between numbers.
Why is Finding the GCF Important?
The GCF has numerous applications across various mathematical fields and real-world scenarios. Some key uses include:
-
Simplifying Fractions: The GCF is crucial for reducing fractions to their simplest form. By dividing both the numerator and denominator by their GCF, we obtain an equivalent fraction with smaller, more manageable numbers. For example, the fraction 12/18 can be simplified to 2/3 by dividing both by their GCF, which is 6.
-
Solving Algebraic Equations: GCF plays a role in factoring algebraic expressions, simplifying equations, and finding solutions. Understanding GCF helps in identifying common terms that can be factored out, leading to simpler equations.
-
Understanding Number Relationships: Finding the GCF helps us understand the relationships between numbers. It reveals common divisors and provides insights into the structure of numbers.
Methods for Finding the GCF of 22 and 33
There are several effective ways to calculate the GCF of two numbers, including 22 and 33. Let's explore some of the most common methods:
1. Listing Factors Method
This method involves listing all the factors of each number and then identifying the largest common factor.
- Factors of 22: 1, 2, 11, 22
- Factors of 33: 1, 3, 11, 33
By comparing the lists, we can see that the common factors are 1 and 11. The greatest of these common factors is 11. Therefore, the GCF of 22 and 33 is 11.
2. Prime Factorization Method
This method involves finding the prime factorization of each number and then identifying the common prime factors raised to the lowest power.
- Prime factorization of 22: 2 x 11
- Prime factorization of 33: 3 x 11
The only common prime factor is 11. Therefore, the GCF of 22 and 33 is 11.
3. Euclidean Algorithm
The Euclidean algorithm is a highly efficient method, especially for larger numbers. It involves repeatedly applying the division algorithm until the remainder is 0. The last non-zero remainder is the GCF.
- Divide the larger number (33) by the smaller number (22): 33 ÷ 22 = 1 with a remainder of 11.
- Replace the larger number with the smaller number (22) and the smaller number with the remainder (11): 22 ÷ 11 = 2 with a remainder of 0.
- Since the remainder is 0, the GCF is the last non-zero remainder, which is 11.
GCF in Real-World Applications
Beyond the theoretical realm, the concept of GCF finds practical applications in several real-world scenarios:
-
Dividing Objects Evenly: Imagine you have 22 apples and 33 oranges, and you want to divide them into identical groups with the same number of apples and oranges in each group. The GCF (11) tells you that you can create 11 identical groups, each with 2 apples and 3 oranges.
-
Measurement Conversions: GCF can simplify conversion problems. For instance, if you need to convert inches to feet, understanding the GCF between the inch and foot measurements helps in simplifying the conversion process.
Expanding on the Concept: Least Common Multiple (LCM)
While this article focuses on the GCF, it's important to mention the closely related concept of the least common multiple (LCM). The LCM is the smallest positive integer that is divisible by both numbers. For 22 and 33, the LCM is 66.
The GCF and LCM are interconnected. For any two integers 'a' and 'b', the product of their GCF and LCM is equal to the product of the two numbers: GCF(a, b) x LCM(a, b) = a x b. In the case of 22 and 33: 11 x 66 = 22 x 33 = 726.
Conclusion: The Significance of Understanding GCF
The seemingly simple task of finding the greatest common factor of 22 and 33 reveals a wealth of mathematical concepts and practical applications. Understanding the various methods for calculating the GCF – listing factors, prime factorization, and the Euclidean algorithm – provides a strong foundation in number theory and problem-solving. The ability to efficiently find the GCF is not just an academic exercise; it's a valuable tool that enhances our understanding of numbers and their relationships, simplifying various tasks in mathematics and beyond. From simplifying fractions to solving real-world problems involving division and distribution, the GCF remains a fundamental concept with lasting significance. This deep dive illustrates that even seemingly basic mathematical concepts, like finding the GCF of 22 and 33, can open doors to a richer understanding of numbers and their properties.
Latest Posts
Latest Posts
-
What Is The Fraction Of 0 1
May 24, 2025
-
What Is The Greatest Common Factor Of 4 And 9
May 24, 2025
-
What Is The Gcf Of 8 And 15
May 24, 2025
-
How Old Am I Born In 1989
May 24, 2025
-
Write 4 27 100 As A Decimal
May 24, 2025
Related Post
Thank you for visiting our website which covers about What Is The Gcf Of 22 And 33 . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.