What Is The Equivalent Of 2/5
listenit
May 11, 2025 · 5 min read
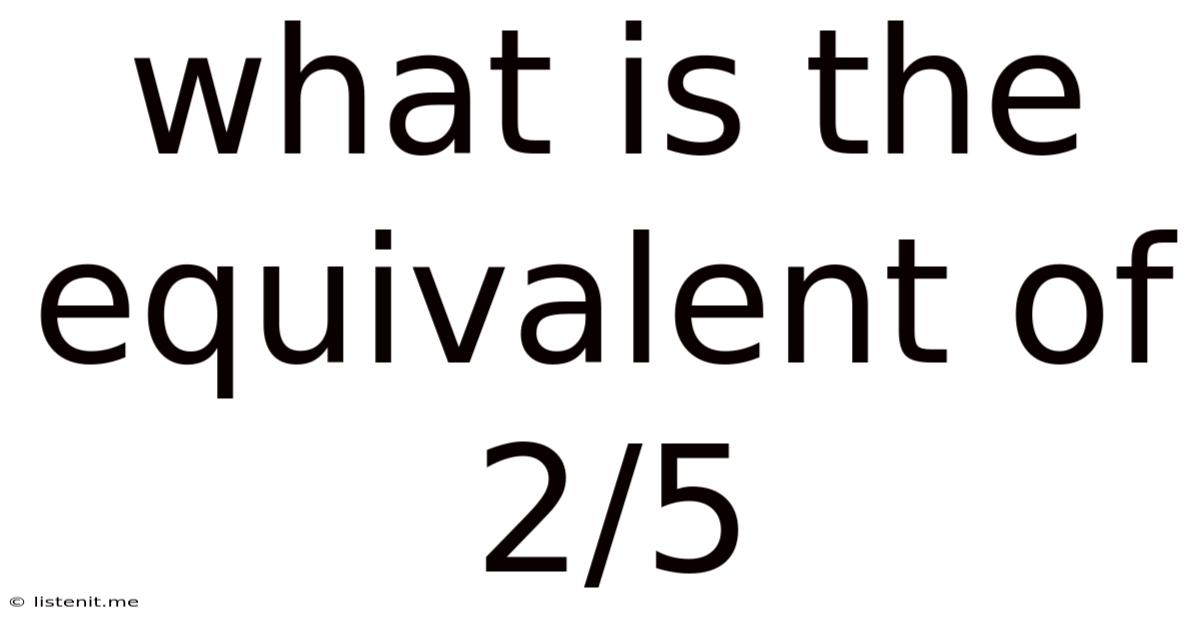
Table of Contents
What is the Equivalent of 2/5? Exploring Fractions, Decimals, and Percentages
Understanding fractions is a fundamental concept in mathematics, crucial for various applications in everyday life and advanced studies. This comprehensive guide delves into the multifaceted nature of the fraction 2/5, exploring its equivalent representations as decimals and percentages, along with practical examples and applications. We'll also touch upon the importance of understanding equivalent fractions in simplifying calculations and solving problems effectively.
Understanding the Fraction 2/5
The fraction 2/5 represents two parts out of a total of five equal parts. The number 2 is the numerator, indicating the number of parts we're considering, while 5 is the denominator, signifying the total number of equal parts that make up the whole. Understanding this basic structure is vital before exploring equivalent forms.
Visualizing 2/5
Imagine a pizza cut into five equal slices. If you eat two slices, you've consumed 2/5 of the pizza. This visual representation makes the fraction more concrete and easier to grasp. Similarly, you can visualize 2/5 using other shapes divided into five equal sections, with two sections shaded to represent the fraction.
Finding Equivalent Fractions
Equivalent fractions represent the same proportion or value, even though they appear different. To find an equivalent fraction for 2/5, you simply multiply both the numerator and the denominator by the same number. This is because multiplying both the top and the bottom of a fraction by the same number is essentially multiplying by 1 (e.g., 2/2 = 1, 3/3 = 1, etc.), which doesn't change the value of the fraction.
Let's find some equivalent fractions for 2/5:
- Multiply by 2: (2 x 2) / (5 x 2) = 4/10
- Multiply by 3: (2 x 3) / (5 x 3) = 6/15
- Multiply by 4: (2 x 4) / (5 x 4) = 8/20
- Multiply by 5: (2 x 5) / (5 x 5) = 10/25
- Multiply by 10: (2 x 10) / (5 x 10) = 20/50
All these fractions – 4/10, 6/15, 8/20, 10/25, 20/50, and so on – are equivalent to 2/5. They all represent the same portion of a whole.
Simplifying Fractions
The opposite of finding an equivalent fraction is simplifying a fraction. This involves dividing both the numerator and the denominator by their greatest common divisor (GCD). For example, the fraction 10/25 can be simplified to 2/5 by dividing both the numerator and denominator by 5 (their GCD). Simplifying fractions makes them easier to work with and understand. The fraction 2/5 is already in its simplest form because 2 and 5 have no common divisors other than 1.
Converting 2/5 to a Decimal
To convert a fraction to a decimal, simply divide the numerator by the denominator. In this case:
2 ÷ 5 = 0.4
Therefore, the decimal equivalent of 2/5 is 0.4.
Converting 2/5 to a Percentage
To convert a decimal to a percentage, multiply by 100 and add the "%" symbol. Since the decimal equivalent of 2/5 is 0.4:
0.4 x 100 = 40%
Therefore, the percentage equivalent of 2/5 is 40%. This means 2/5 represents 40 parts out of 100 equal parts.
Real-world Applications of 2/5 and Equivalent Fractions
Understanding 2/5 and its equivalents is essential in numerous real-world situations:
-
Shopping Discounts: A 40% discount on an item means you're paying 60% of the original price (100% - 40% = 60%). This is equivalent to paying 3/5 of the original price (since 60%/100% simplifies to 3/5).
-
Cooking and Baking: Recipes often use fractions. If a recipe calls for 2/5 of a cup of flour, you can use an equivalent amount, like 4/10 of a cup or 8/20 of a cup if needed.
-
Data Analysis: Representing data as fractions and percentages helps to understand and communicate proportions effectively. For example, if 40% of survey respondents answered "yes," this is equivalent to 2/5 of the respondents.
-
Probability and Statistics: Probabilities are often expressed as fractions or percentages. If the probability of an event is 2/5, it means there's a 40% chance of it happening.
-
Geometry and Measurement: Fractions are integral to geometric calculations, such as finding the area of a shape or the length of a line segment. Understanding equivalent fractions is important for solving various geometrical problems.
Beyond 2/5: Generalizing the Concept of Equivalent Fractions
The principles discussed for 2/5 apply to all fractions. Any fraction can be expressed in infinitely many equivalent forms by multiplying or dividing both the numerator and denominator by the same non-zero number. The ability to find equivalent fractions and simplify them is a crucial skill in simplifying mathematical expressions and solving complex problems.
Working with Different Denominators
When working with different fractions, finding a common denominator is often necessary before performing addition or subtraction. For example, adding 2/5 and 1/3 requires finding a common denominator, which in this case is 15. This allows us to rewrite the fractions as 6/15 and 5/15, respectively, making addition straightforward (6/15 + 5/15 = 11/15).
Conclusion: Mastering Fractions for Real-World Success
Understanding the concept of equivalent fractions, and specifically the various representations of 2/5, is a foundational skill that extends far beyond the classroom. From calculating discounts to understanding probabilities and interpreting data, the ability to work confidently with fractions, decimals, and percentages is essential for success in numerous aspects of life. By mastering these concepts, you equip yourself with the tools necessary to tackle various mathematical challenges and solve real-world problems efficiently. The examples provided in this guide highlight the practical importance of understanding equivalent fractions, empowering you to confidently apply these skills in various contexts. Remember, the ability to convert between fractions, decimals, and percentages is a versatile skill with wide-ranging applications in everyday life and beyond.
Latest Posts
Latest Posts
-
Is A Year One Trip Around The Sun
May 12, 2025
-
Greatest Common Factor 36 And 90
May 12, 2025
-
How Many Valence Electrons Does Platinum Have
May 12, 2025
-
Cells Communicate With One Another Via
May 12, 2025
-
Arrange The Following Elements In Order Of Decreasing Electronegativity
May 12, 2025
Related Post
Thank you for visiting our website which covers about What Is The Equivalent Of 2/5 . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.