What Is The Discriminant Of 3x 2 10x 2
listenit
May 10, 2025 · 4 min read
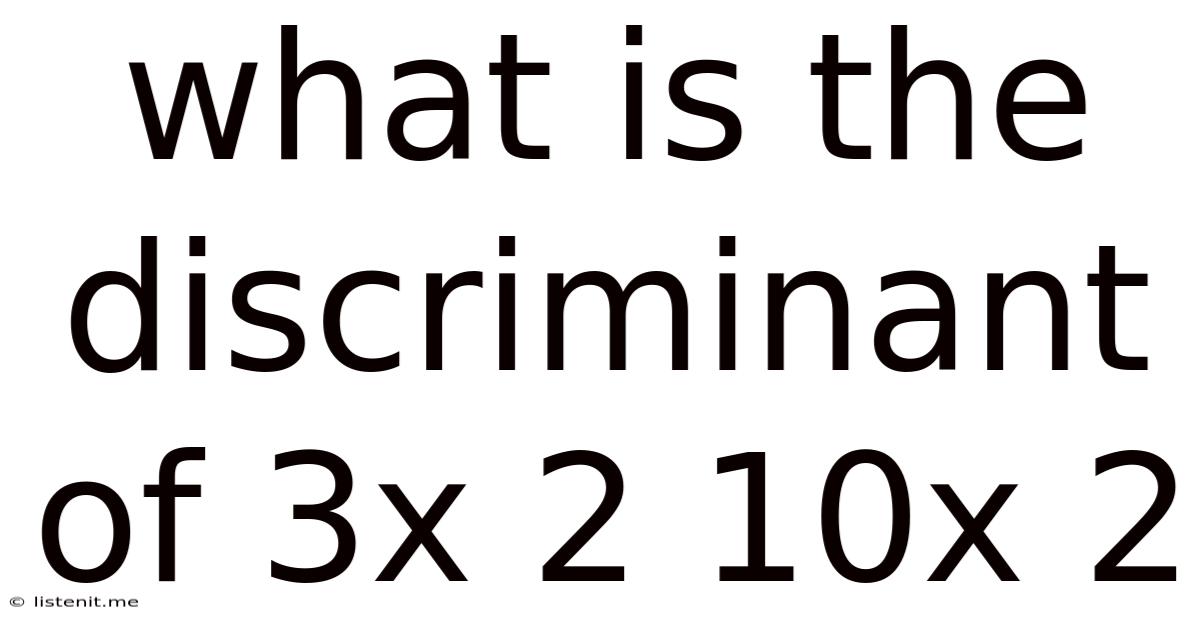
Table of Contents
What is the Discriminant of 3x² + 10x + 2? A Deep Dive into Quadratic Equations
The discriminant, a powerful tool in algebra, provides crucial insights into the nature of the solutions of a quadratic equation. Understanding the discriminant allows us to determine whether a quadratic equation has real or complex roots, and whether those roots are distinct or repeated. This article will delve into the concept of the discriminant, focusing specifically on the equation 3x² + 10x + 2 = 0, exploring its calculation, interpretation, and broader implications within the realm of quadratic equations.
Understanding Quadratic Equations and Their Roots
A quadratic equation is a polynomial equation of the second degree, generally expressed in the form:
ax² + bx + c = 0
where 'a', 'b', and 'c' are constants, and 'a' is not equal to zero. The solutions, or roots, of this equation represent the x-values where the quadratic function intersects the x-axis. These roots can be real numbers, imaginary numbers, or a combination of both.
Introducing the Discriminant: A Key to Understanding Roots
The discriminant (often denoted as Δ or D) is a part of the quadratic formula that determines the nature of the roots of a quadratic equation. It's calculated using the coefficients a, b, and c from the standard quadratic equation:
Δ = b² - 4ac
The value of the discriminant dictates the characteristics of the roots:
1. Δ > 0 (Discriminant is positive):
-
Two distinct real roots: The parabola intersects the x-axis at two different points. These roots can be found using the quadratic formula:
x = (-b ± √Δ) / 2a
2. Δ = 0 (Discriminant is zero):
-
One real root (repeated root): The parabola touches the x-axis at exactly one point. This represents a repeated or double root. The root can be calculated as:
x = -b / 2a
3. Δ < 0 (Discriminant is negative):
- Two complex conjugate roots: The parabola does not intersect the x-axis. The roots are complex numbers, which involve the imaginary unit 'i' (where i² = -1). These roots are always conjugate pairs, meaning they have the same real part but opposite imaginary parts. The quadratic formula still applies, resulting in complex roots.
Calculating the Discriminant for 3x² + 10x + 2 = 0
Now, let's apply this knowledge to the specific quadratic equation: 3x² + 10x + 2 = 0. By comparing this equation to the standard form ax² + bx + c = 0, we can identify the coefficients:
- a = 3
- b = 10
- c = 2
Substituting these values into the discriminant formula:
Δ = b² - 4ac = (10)² - 4 * (3) * (2) = 100 - 24 = 76
Interpreting the Result: Analyzing the Roots
Since the discriminant (Δ) is 76, which is greater than 0, we can conclude that the quadratic equation 3x² + 10x + 2 = 0 has two distinct real roots.
Finding the Actual Roots Using the Quadratic Formula
To find the exact values of these roots, we can utilize the quadratic formula:
x = (-b ± √Δ) / 2a
Substituting the values of a, b, and Δ:
x = (-10 ± √76) / (2 * 3)
Simplifying:
x = (-10 ± 2√19) / 6
Further simplification yields:
x₁ = (-5 + √19) / 3
x₂ = (-5 - √19) / 3
Therefore, the two distinct real roots of the equation 3x² + 10x + 2 = 0 are approximately x₁ ≈ -0.21 and x₂ ≈ -3.12.
Graphical Representation and Visualization
The graph of the quadratic function y = 3x² + 10x + 2 is a parabola that opens upwards (since a > 0). The fact that the discriminant is positive confirms that the parabola intersects the x-axis at two distinct points, corresponding to the two real roots we calculated. Visualizing this graphically reinforces the understanding of the relationship between the discriminant and the roots of the equation.
Applications of the Discriminant Beyond Simple Root Analysis
The discriminant's significance extends beyond simply determining the nature of roots. It plays a crucial role in various mathematical applications, including:
1. Determining the Tangency of Lines and Curves:
The discriminant can be used to analyze whether a line is tangent to a curve. If the discriminant of the resulting quadratic equation (formed by solving the system of equations) is zero, it indicates tangency.
2. Solving Systems of Equations:
When solving systems of equations involving quadratic and linear equations, the discriminant helps to determine the number of intersection points between the curves.
3. Optimization Problems:
In optimization problems, the discriminant can help determine the nature of critical points (maximum, minimum, or saddle points).
4. Nature of Conic Sections:
The discriminant plays a vital role in classifying conic sections (parabolas, ellipses, hyperbolas) based on their equations.
Conclusion: The Discriminant as a Fundamental Tool
The discriminant is a fundamental concept in algebra, providing a concise and efficient method for determining the nature and number of solutions to quadratic equations. Understanding the discriminant's implications is crucial not only for solving quadratic equations but also for broader applications in various mathematical contexts. The detailed analysis of the equation 3x² + 10x + 2 = 0 highlights the practical application of the discriminant and demonstrates its power as a vital tool in algebraic analysis. Its ability to predict the characteristics of roots without explicitly solving the equation makes it a cornerstone of quadratic equation analysis and beyond. Mastering the discriminant enhances problem-solving skills and provides a deeper understanding of the intricacies of quadratic functions and their behavior.
Latest Posts
Latest Posts
-
Describe Two Major Shortcomings Of Bohrs Model Of The Atom
May 10, 2025
-
Benefits Of Asexual Reproduction In Plants
May 10, 2025
-
The Average Kinetic Energy Of Water Molecules Decreases When
May 10, 2025
-
What Is The Amplitude Of A Transverse Wave
May 10, 2025
-
What Is The Special Property Of Water
May 10, 2025
Related Post
Thank you for visiting our website which covers about What Is The Discriminant Of 3x 2 10x 2 . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.