What Is The Decimal Form Of 5/9
listenit
May 12, 2025 · 4 min read
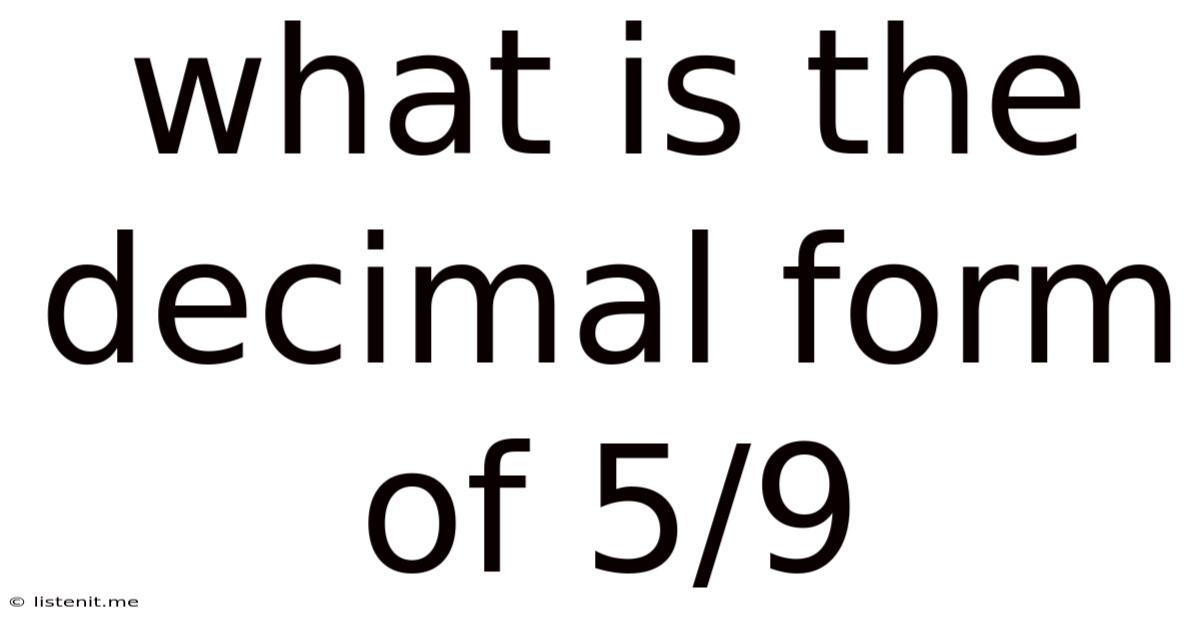
Table of Contents
What is the Decimal Form of 5/9? A Deep Dive into Fraction Conversion
The seemingly simple question, "What is the decimal form of 5/9?" opens a door to a broader understanding of fractions, decimals, and the fundamental principles of mathematics. While a quick calculation might provide the answer, exploring the why behind the conversion offers valuable insights into mathematical processes and strengthens foundational skills. This article will not only answer the question but will also delve into the methods used, explore alternative approaches, and discuss the broader implications of fraction-to-decimal conversions.
Understanding Fractions and Decimals
Before jumping into the conversion of 5/9, let's solidify our understanding of fractions and decimals.
Fractions: A fraction represents a part of a whole. It consists of two numbers: the numerator (the top number) and the denominator (the bottom number). The numerator indicates how many parts we have, and the denominator indicates how many equal parts the whole is divided into.
Decimals: Decimals are another way of representing parts of a whole. They use a base-ten system, where each place value to the right of the decimal point represents a power of ten (tenths, hundredths, thousandths, and so on).
Converting 5/9 to a Decimal: The Long Division Method
The most straightforward method to convert a fraction to a decimal is through long division. We divide the numerator (5) by the denominator (9).
0.555...
9 | 5.000
-4.5
0.50
-0.45
0.050
-0.045
0.005...
As you can see, the division results in a repeating decimal: 0.555... The digit 5 repeats infinitely. This is often represented as 0.5̅ (the bar over the 5 indicates the repeating digit).
Why the Repeating Decimal?
The reason we get a repeating decimal is because 9 is not a factor of 10 (or any power of 10). When the denominator of a fraction cannot be expressed as a product of 2s and 5s, the resulting decimal is usually a repeating or non-terminating decimal.
Alternative Methods for Conversion
While long division is the most common method, alternative approaches can provide a deeper understanding and sometimes offer a quicker solution.
Using a Calculator
A calculator provides a quick and efficient way to convert fractions to decimals. Simply enter 5 ÷ 9 and the calculator will display the decimal equivalent, likely showing 0.555555... or a rounded version. While convenient, this method lacks the educational benefit of understanding the underlying mathematical process.
Understanding the Relationship Between Fractions and Decimals
Understanding the relationship between fractions and decimals helps in visualizing and predicting the decimal equivalent. Consider this: 1/9 = 0.1̅, 2/9 = 0.2̅, 3/9 = 0.3̅, and so on. This pattern highlights the systematic nature of converting ninths to decimals. This approach allows you to quickly determine the decimal equivalent of 5/9 by recognizing the pattern.
The Significance of Repeating Decimals
Repeating decimals are a fascinating aspect of mathematics. They represent rational numbers – numbers that can be expressed as a fraction of two integers. Irrational numbers, like pi (π) or the square root of 2, have non-repeating, non-terminating decimals. The repeating nature of 0.5̅ signifies its rationality.
Practical Applications of Fraction-to-Decimal Conversions
The ability to convert fractions to decimals is crucial in various fields:
- Engineering and Science: Precise measurements and calculations often require decimal representation.
- Finance: Calculating interest rates, percentages, and profits often involves converting fractions to decimals.
- Computer Programming: Representing and manipulating numerical data within computer programs necessitates understanding fraction-to-decimal conversions.
- Everyday Life: From calculating tips in restaurants to dividing recipes, converting fractions to decimals helps in practical, daily calculations.
Beyond 5/9: Exploring Other Fraction Conversions
The principles discussed for converting 5/9 can be applied to other fractions. Understanding the long division method, recognizing patterns, and using calculators are all valuable tools for converting various fractions to their decimal equivalents. Consider these examples:
- 1/3: This results in a repeating decimal: 0.3̅
- 2/7: This also yields a repeating decimal: 0.285714̅
- 3/8: This gives a terminating decimal: 0.375 (because the denominator can be expressed as 2³)
Rounding and Approximation
In many practical applications, it's necessary to round repeating decimals to a certain number of decimal places. For example, 0.5̅ could be rounded to 0.56, 0.556, or more decimal places, depending on the required level of accuracy. It’s crucial to understand the implications of rounding and the potential introduction of errors in calculations.
Conclusion: Mastering Fraction-to-Decimal Conversions
Converting 5/9 to its decimal form – 0.5̅ – is more than just a simple calculation. It’s an opportunity to explore fundamental mathematical concepts, strengthen problem-solving skills, and appreciate the elegance and interconnectedness of mathematical systems. Understanding the different methods, recognizing patterns, and appreciating the significance of repeating decimals empowers you to tackle more complex mathematical challenges with confidence. This skill, fundamental as it may seem, is a cornerstone of mathematical literacy and finds widespread application in various fields. So, next time you encounter a fraction, remember the journey from fraction to decimal is an enriching mathematical adventure.
Latest Posts
Related Post
Thank you for visiting our website which covers about What Is The Decimal Form Of 5/9 . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.