What Is The Decimal For 2 3/4
listenit
May 12, 2025 · 5 min read
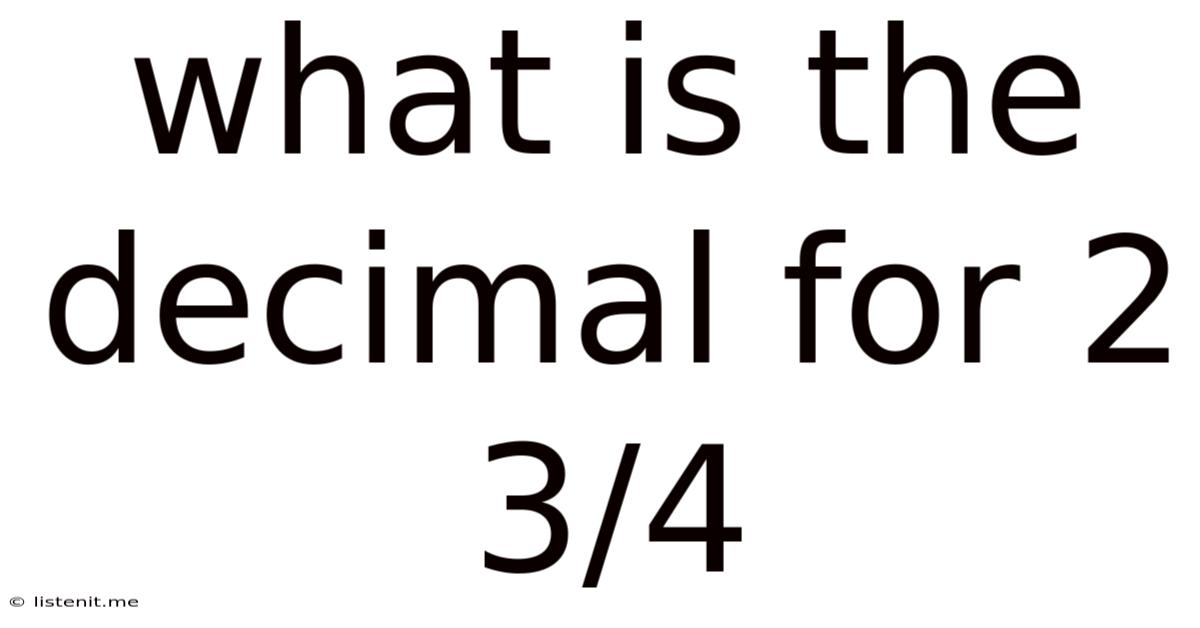
Table of Contents
What is the Decimal for 2 3/4? A Comprehensive Guide
The question, "What is the decimal for 2 3/4?" seems simple enough, but it opens a door to understanding fundamental concepts in mathematics, particularly the relationship between fractions and decimals. This comprehensive guide will not only answer this specific question but also delve into the broader context of fraction-to-decimal conversion, offering various methods and practical applications.
Understanding Fractions and Decimals
Before we tackle the conversion, let's clarify the basics. A fraction represents a part of a whole. It consists of a numerator (the top number) and a denominator (the bottom number). The numerator indicates how many parts you have, while the denominator indicates how many equal parts the whole is divided into. In our example, 2 3/4, we have a mixed fraction – a whole number (2) combined with a fraction (3/4).
A decimal, on the other hand, represents a number based on the powers of 10. Each digit to the right of the decimal point represents a fraction with a denominator that is a power of 10 (10, 100, 1000, etc.). For example, 0.1 is 1/10, 0.01 is 1/100, and so on.
Converting 2 3/4 to a Decimal: The Step-by-Step Approach
There are several ways to convert the mixed fraction 2 3/4 into its decimal equivalent. Let's explore the most common methods:
Method 1: Converting the Fraction to a Decimal, Then Adding the Whole Number
This is arguably the simplest approach. We first convert the fraction 3/4 into a decimal and then add the whole number 2.
-
Divide the numerator by the denominator: 3 ÷ 4 = 0.75
-
Add the whole number: 2 + 0.75 = 2.75
Therefore, the decimal equivalent of 2 3/4 is 2.75.
Method 2: Converting the Mixed Fraction to an Improper Fraction, Then to a Decimal
This method involves first transforming the mixed fraction into an improper fraction, where the numerator is greater than or equal to the denominator.
-
Convert to an improper fraction: To do this, multiply the whole number (2) by the denominator (4), add the numerator (3), and keep the same denominator. This gives us (2 * 4) + 3 = 11, so the improper fraction is 11/4.
-
Divide the numerator by the denominator: 11 ÷ 4 = 2.75
Again, the decimal equivalent of 2 3/4 is 2.75.
Method 3: Using Decimal Equivalents of Common Fractions
This method relies on memorizing the decimal equivalents of common fractions. Knowing that 1/4 = 0.25, we can easily deduce that 3/4 = 3 * 0.25 = 0.75. Adding the whole number 2, we get 2.75. This method is efficient for common fractions but less useful for more complex ones.
Practical Applications of Fraction-to-Decimal Conversions
The ability to convert fractions to decimals is crucial in numerous real-world applications:
-
Financial Calculations: Dealing with money often involves fractions of a dollar (cents). Converting fractions to decimals makes calculations simpler, like calculating discounts, taxes, or interest rates. For example, a 3/4 discount on a $100 item translates to a $75 discount (0.75 * $100).
-
Measurement and Engineering: In engineering and construction, precise measurements are vital. Fractions are frequently used in blueprints and designs, but decimals are often more convenient for calculations and computer-aided design (CAD) software.
-
Data Analysis and Statistics: Statistical analysis often involves working with data expressed as fractions or percentages. Converting fractions to decimals is essential for calculations and presenting data in various formats.
-
Cooking and Baking: Recipes often include fractional measurements. Converting these fractions to decimals can simplify the measuring process, especially when using digital scales.
-
Scientific Calculations: Many scientific formulas and calculations require numerical values expressed in decimal form. Converting fractional measurements to decimal values is a necessary step in many scientific experiments and computations.
Beyond 2 3/4: Converting Other Fractions to Decimals
The methods described above can be applied to convert any fraction to its decimal equivalent. However, some fractions result in terminating decimals (like 2.75), while others result in repeating decimals (decimals with a pattern of digits that repeat indefinitely).
For example:
- 1/2 = 0.5 (terminating decimal)
- 1/3 = 0.3333... (repeating decimal, often represented as 0.3̅)
- 1/7 = 0.142857142857... (repeating decimal, often represented as 0.1̅4̅2̅8̅5̅7̅)
Repeating decimals can be represented using a bar above the repeating digits, as shown above. In some applications, it's sufficient to round repeating decimals to a certain number of decimal places.
Mastering Fraction-Decimal Conversions: Tips and Practice
Becoming proficient in fraction-to-decimal conversions requires practice. Here are some tips:
-
Memorize common fraction-decimal equivalents: Knowing the decimal equivalents of fractions like 1/2, 1/4, 1/3, 1/5, and 1/10 will significantly speed up your calculations.
-
Practice regularly: Work through various examples, including mixed fractions and improper fractions.
-
Use different methods: Try all the methods described above to find the one you're most comfortable with.
-
Check your work: Always double-check your calculations to ensure accuracy. You can use a calculator to verify your answers, but understanding the underlying process is crucial.
-
Focus on understanding, not just memorization: While memorization can be helpful, a deeper understanding of the concepts of fractions and decimals will make you a more confident and capable mathematician.
Conclusion: The Importance of Understanding Decimal Equivalents
The simple question of finding the decimal equivalent of 2 3/4 has led us on a journey through the fundamental concepts of fractions and decimals, highlighting their interrelationship and practical applications. Mastering the conversion process is not just about obtaining a numerical answer; it's about developing a deeper understanding of mathematical principles and their relevance in various fields. By consistently practicing and applying these methods, you'll strengthen your mathematical skills and improve your ability to tackle more complex problems involving fractions and decimals. The ability to seamlessly convert between fractions and decimals is a valuable asset in numerous academic and professional contexts, making it a skill worth investing time and effort in mastering.
Latest Posts
Latest Posts
-
Find The Value Of X In The Figure
May 12, 2025
-
Lighting A Match Chemical Or Physical Change
May 12, 2025
-
Greatest Common Factor Of 56 And 16
May 12, 2025
-
Why Was Wegeners Theory Of Continental Drift Rejected
May 12, 2025
-
Is Mixing Sugar And Water A Chemical Change
May 12, 2025
Related Post
Thank you for visiting our website which covers about What Is The Decimal For 2 3/4 . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.