What Is Molar Volume Of Gas At Stp
listenit
Apr 04, 2025 · 6 min read
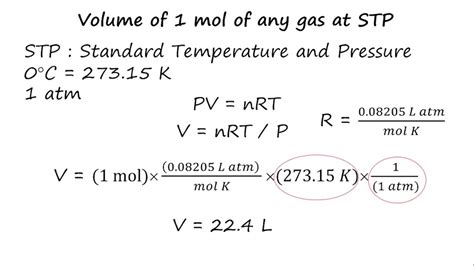
Table of Contents
What is the Molar Volume of a Gas at STP? A Comprehensive Guide
The molar volume of a gas at standard temperature and pressure (STP) is a fundamental concept in chemistry, crucial for understanding gas behavior and stoichiometric calculations. This comprehensive guide will delve into the definition, calculation, significance, and applications of molar volume, ensuring a thorough understanding of this vital concept.
Understanding Standard Temperature and Pressure (STP)
Before diving into the molar volume, it's essential to define STP. While there have been slight variations throughout history, the most commonly accepted definition today is:
- Temperature: 273.15 Kelvin (0° Celsius or 32° Fahrenheit)
- Pressure: 1 atmosphere (atm) or 101.325 kilopascals (kPa)
These conditions represent a readily reproducible and standardized set of parameters for comparing the behavior of gases. It's crucial to note that other definitions of STP exist, particularly older ones, so always check the specific definition being used in a given context. This article will adhere to the modern definition stated above.
Defining Molar Volume
The molar volume of a gas is defined as the volume occupied by one mole of that gas under specified conditions of temperature and pressure. At STP, this volume is remarkably consistent for ideal gases. The concept rests on the ideal gas law, which provides a theoretical model for the behavior of gases.
The Ideal Gas Law and its Role in Molar Volume Calculation
The ideal gas law is a mathematical equation that relates pressure (P), volume (V), number of moles (n), and temperature (T) of an ideal gas:
PV = nRT
Where:
- P is the pressure of the gas
- V is the volume of the gas
- n is the number of moles of the gas
- R is the ideal gas constant
- T is the temperature of the gas
The ideal gas constant (R) is a proportionality constant that depends on the units used for pressure, volume, and temperature. Common values for R include:
- 0.0821 L·atm/mol·K (liters-atmospheres per mole-Kelvin)
- 8.314 J/mol·K (Joules per mole-Kelvin)
This equation is fundamental to understanding the molar volume at STP. If we set n = 1 mole (representing one mole of gas), and substitute the STP values for P and T, we can calculate the volume (V).
Calculating the Molar Volume of a Gas at STP
Let's calculate the molar volume (V<sub>m</sub>) of an ideal gas at STP using the ideal gas law:
- Set n = 1 mole (we want the volume of one mole of gas).
- Substitute STP values: P = 1 atm, T = 273.15 K.
- Choose the appropriate value of R: We'll use R = 0.0821 L·atm/mol·K for convenience, as it aligns with the units of pressure and volume we're using.
Now we can rearrange the ideal gas law to solve for V:
V = nRT/P
Substituting the values:
V<sub>m</sub> = (1 mol) * (0.0821 L·atm/mol·K) * (273.15 K) / (1 atm)
V<sub>m</sub> ≈ 22.4 L
Therefore, the molar volume of an ideal gas at STP is approximately 22.4 liters per mole. This means that one mole of any ideal gas will occupy a volume of approximately 22.4 liters under standard temperature and pressure conditions.
Limitations of the Ideal Gas Law and Molar Volume at STP
It's crucial to remember that the ideal gas law is a model. Real gases deviate from ideal behavior, particularly at high pressures and low temperatures. Intermolecular forces and the finite volume of gas molecules become significant factors under these conditions, causing deviations from the predicted molar volume of 22.4 L/mol.
The approximation of 22.4 L/mol is most accurate for gases at relatively low pressures and high temperatures where intermolecular forces are minimized. For real gases under non-ideal conditions, more sophisticated equations of state, like the van der Waals equation, are necessary for accurate volume predictions.
Significance and Applications of Molar Volume
The molar volume at STP has significant practical applications in various areas of chemistry:
-
Stoichiometric Calculations: Molar volume provides a convenient conversion factor between the volume and moles of a gas in stoichiometry problems. It simplifies calculations involving gas-phase reactions.
-
Gas Density Determination: Knowing the molar volume allows for easy calculation of gas density at STP. Gas density (ρ) can be calculated using the formula: ρ = molar mass / molar volume.
-
Gas Law Problems: The concept of molar volume is fundamental to solving problems involving the ideal gas law, and helps in visualizing the quantities involved in gaseous systems.
-
Environmental Science: The molar volume is relevant to calculations involving atmospheric gases and pollution estimations.
-
Industrial Chemistry: In various industrial processes involving gases, understanding molar volume is critical for process control and optimization.
Beyond STP: Molar Volume at Other Conditions
The molar volume isn't restricted to STP. Using the ideal gas law, we can calculate the molar volume under any set of temperature and pressure conditions. Simply substitute the desired values of P and T into the ideal gas law equation and solve for V. Remember that significant deviations from ideality can be expected at high pressures and low temperatures.
Comparing Ideal and Real Gases: A Closer Look at Deviations
Real gases deviate from ideal behavior due to two primary factors:
-
Intermolecular Forces: Attractive forces between gas molecules cause them to be slightly closer together than predicted by the ideal gas law. This reduces the volume occupied by the gas.
-
Molecular Volume: Gas molecules themselves occupy a finite volume. In the ideal gas model, molecules are treated as point particles with no volume. However, in real gases, the molecular volume becomes significant, especially at high pressures. This effect increases the volume occupied by the gas.
These deviations are generally small at low pressures and high temperatures, which explains why the 22.4 L/mol approximation works reasonably well under STP conditions for many gases. However, at higher pressures or lower temperatures, these effects become more significant, resulting in greater deviations from ideality.
Advanced Concepts and Further Exploration
For a more in-depth understanding, further exploration into the following concepts is recommended:
-
Compressibility Factor (Z): This factor quantifies the deviation of real gases from ideal behavior. Z = PV/nRT, where Z = 1 for an ideal gas.
-
Van der Waals Equation: This equation accounts for intermolecular forces and molecular volume, providing a more accurate description of real gas behavior.
-
Virial Equations of State: These are more complex equations that offer even greater accuracy in predicting the behavior of real gases.
-
Corresponding States Principle: This principle relates the properties of different gases at corresponding states (e.g., reduced temperature and pressure).
Conclusion
The molar volume of a gas at STP, approximately 22.4 L/mol, is a cornerstone concept in chemistry. While based on the ideal gas law, it serves as a practical approximation for various calculations involving gases under standard conditions. Understanding its limitations and the factors affecting deviations from ideality is vital for accurate calculations and a comprehensive understanding of gas behavior. By grasping the fundamental principles discussed here and venturing into the advanced concepts mentioned, you can develop a strong foundation in the realm of gas laws and their applications.
Latest Posts
Latest Posts
-
Least Common Multiple Of 7 2 And 3
Apr 04, 2025
-
What Is The Purpose Of The Experiment
Apr 04, 2025
-
Why Must The Remainder Be Less Than The Divisor
Apr 04, 2025
-
How Many Inches In Two Yards
Apr 04, 2025
-
What Is 9 10 In Decimal Form
Apr 04, 2025
Related Post
Thank you for visiting our website which covers about What Is Molar Volume Of Gas At Stp . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.
