What Is 90 Percent Of 30
listenit
Apr 03, 2025 · 5 min read
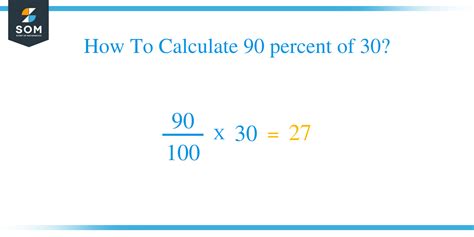
Table of Contents
What is 90 Percent of 30? A Deep Dive into Percentages and Their Applications
Finding 90 percent of 30 might seem like a simple arithmetic problem, suitable only for elementary school. However, understanding how to calculate percentages and their real-world applications is crucial for various aspects of life, from managing personal finances to understanding complex data in professional settings. This article delves into the calculation of 90% of 30, explaining the process in detail, exploring different methods, and highlighting the broader significance of percentage calculations in everyday life.
Calculating 90% of 30: The Basics
The most straightforward method to calculate 90% of 30 involves converting the percentage into a decimal and then multiplying it by the number. Here's how:
-
Convert the percentage to a decimal: To convert 90% to a decimal, divide it by 100: 90% ÷ 100 = 0.9
-
Multiply the decimal by the number: Multiply the decimal (0.9) by 30: 0.9 x 30 = 27
Therefore, 90% of 30 is 27.
Alternative Methods for Calculating Percentages
While the above method is the most common and arguably the easiest, there are other approaches you can use to calculate percentages, each with its own advantages depending on the context and your mathematical comfort level.
Method 2: Using Fractions
Percentages can also be expressed as fractions. 90% can be written as 90/100, which simplifies to 9/10. To find 90% of 30 using this method:
-
Express the percentage as a fraction: 90% = 90/100 = 9/10
-
Multiply the fraction by the number: (9/10) x 30 = 270/10 = 27
This method is particularly useful when dealing with percentages that are easily expressed as simple fractions.
Method 3: Finding 10% and Scaling Up
A helpful trick for mental math, especially with multiples of 10, is to first calculate 10% and then scale it up.
-
Find 10% of 30: 10% of 30 is (10/100) x 30 = 3
-
Scale up to 90%: Since 90% is nine times 10%, multiply the result by 9: 3 x 9 = 27
This method can be faster for mental calculations, particularly when dealing with percentages that are multiples of 10.
Real-World Applications of Percentage Calculations
The ability to calculate percentages isn't confined to mathematical exercises; it's a fundamental skill with far-reaching applications in various aspects of daily life. Here are some examples:
1. Personal Finance:
- Discounts and Sales: Understanding percentages is essential for taking advantage of sales and discounts. If a store offers a 20% discount on a $100 item, you can quickly calculate the final price by finding 80% of $100.
- Interest Rates: Calculating interest on loans, savings accounts, and credit cards involves working with percentages. Understanding interest rates helps in making informed financial decisions.
- Taxes: Taxes are often calculated as a percentage of income or the value of goods and services. Knowing how to calculate percentages ensures you understand your tax obligations.
- Tips and Gratuities: Calculating tips in restaurants or other service-based industries often involves determining a percentage of the total bill.
2. Business and Commerce:
- Profit Margins: Businesses use percentages to calculate profit margins—the difference between the cost of goods and the selling price.
- Market Share: Companies track their market share, which is expressed as a percentage of the total market.
- Growth Rates: Businesses analyze growth rates, such as revenue growth or customer acquisition, which are typically expressed as percentages.
- Sales Performance: Sales targets and performance are frequently measured as percentages of overall goals.
3. Science and Data Analysis:
- Statistical Analysis: Percentages are frequently used to represent data in statistical analysis, allowing for easy comparison and interpretation of results.
- Experimental Results: In scientific experiments, results are often expressed as percentages to show the success rate or the proportion of a sample exhibiting a particular characteristic.
- Data Representation: Graphs and charts often use percentages to visually represent data proportions, making it easier to understand trends and patterns.
4. Everyday Life:
- Recipe Scaling: Adjusting recipes to serve more or fewer people involves using percentages to scale the ingredient quantities.
- Measurement Conversions: Converting between different units of measurement sometimes involves percentage calculations.
- Surveys and Polls: Results from surveys and polls are usually presented as percentages, representing the proportion of respondents holding specific views.
Beyond the Basics: Advanced Percentage Calculations
While calculating 90% of 30 is a simple problem, understanding percentages goes beyond basic calculations. More complex scenarios might involve:
- Calculating the percentage increase or decrease: This involves finding the difference between two values and expressing it as a percentage of the original value.
- Finding the original value given a percentage: If you know the final value after a percentage increase or decrease, you can work backward to find the original value.
- Working with compound percentages: Compound percentages involve applying a percentage repeatedly over time, such as with compound interest.
- Solving percentage problems with multiple variables: More complex problems might involve multiple percentages or unknown values.
Conclusion: The Importance of Mastering Percentages
The ability to calculate percentages accurately and efficiently is a valuable skill applicable in numerous situations. Understanding the different methods for calculating percentages, from basic multiplication to using fractions, empowers you to tackle problems in personal finance, business, science, and everyday life with confidence. While seemingly simple, the calculation of 90% of 30 serves as a gateway to understanding a wider world of numerical relationships and their practical implications. Mastering this fundamental concept lays a solid foundation for tackling more complex percentage-based problems and enhances your ability to interpret and utilize data effectively.
Latest Posts
Latest Posts
-
What Is The Absolute Value Of 10
Apr 04, 2025
-
What Are The Functions Of A Fruit
Apr 04, 2025
-
Is Wood Rotting A Physical Or Chemical Property
Apr 04, 2025
-
What Is Anything That Takes Up Space And Has Mass
Apr 04, 2025
-
Number Of Valence Electrons Of Potassium
Apr 04, 2025
Related Post
Thank you for visiting our website which covers about What Is 90 Percent Of 30 . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.
