What Is 9 To The Power Of 0
listenit
Apr 02, 2025 · 5 min read
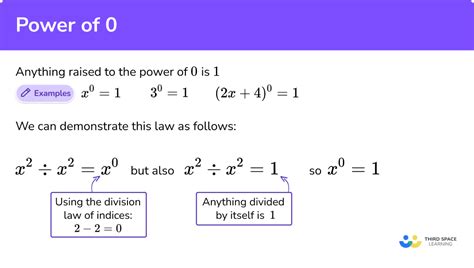
Table of Contents
What is 9 to the Power of 0? Unlocking the Mystery of Exponents
The question, "What is 9 to the power of 0?" might seem deceptively simple. It's a fundamental concept in mathematics, yet it often trips up students and even seasoned mathematicians when they first encounter it. This comprehensive guide will not only answer this question definitively but also explore the underlying principles of exponents, providing you with a solid understanding of this crucial mathematical concept. We'll delve into the reasoning behind the seemingly counterintuitive rule, explore its practical applications, and address common misconceptions.
Understanding Exponents: The Foundation
Before we tackle 9 to the power of 0, let's build a strong foundation by reviewing the basics of exponents. An exponent, also known as a power or index, indicates how many times a number (the base) is multiplied by itself.
For example:
- 9² (9 to the power of 2, or 9 squared): This means 9 multiplied by itself once: 9 x 9 = 81
- 9³ (9 to the power of 3, or 9 cubed): This means 9 multiplied by itself twice: 9 x 9 x 9 = 729
- 9⁴ (9 to the power of 4): This means 9 multiplied by itself three times: 9 x 9 x 9 x 9 = 6561
Notice a pattern? As the exponent increases by one, we multiply the result by the base (9) again. This pattern is crucial for understanding what happens when the exponent is 0.
The Zero Exponent Rule: Why it Works
The rule states that any non-zero number raised to the power of 0 equals 1. Therefore, 9⁰ = 1. But why? This isn't intuitively obvious, and understanding the reason behind this rule is key to mastering exponents.
Let's consider the following pattern, using 9 as our base:
- 9⁴ = 6561
- 9³ = 729 (6561 / 9)
- 9² = 81 (729 / 9)
- 9¹ = 9 (81 / 9)
Notice that as the exponent decreases by 1, we divide the previous result by the base (9). Continuing this pattern logically:
- 9⁰ = 9 / 9 = 1
This consistent pattern demonstrates why 9⁰ must equal 1. This isn't a magical rule; it's a consequence of maintaining the consistent relationship between exponents and their corresponding values.
Beyond the Pattern: A More Formal Explanation
While the pattern is a helpful illustration, a more formal mathematical explanation involves the properties of exponents. Specifically, the property of exponent division:
a<sup>m</sup> / a<sup>n</sup> = a<sup>(m-n)</sup>
Let's apply this property to our problem:
Consider 9¹ / 9¹. This simplifies to:
- 9<sup>(1-1)</sup> = 9⁰
Since 9¹ / 9¹ = 9 / 9 = 1, it logically follows that 9⁰ = 1.
This formal approach solidifies the understanding of the zero exponent rule, demonstrating its logical consistency within the broader framework of exponent properties.
Addressing Common Misconceptions
Several common misconceptions surround the zero exponent rule. Let's address some of them:
Misconception 1: 0⁰ is undefined.
This is true. The expression 0⁰ is considered an indeterminate form. The reason for this is complex and involves limits and calculus. Essentially, there's no consistent definition for 0⁰ that aligns with other mathematical properties. This is different from a non-zero number raised to the power of 0, which is always 1.
Misconception 2: Any number raised to the power of 0 is 0.
This is incorrect. Only 0 raised to the power of 0 is undefined; any non-zero number raised to the power of 0 is 1.
Misconception 3: The rule is arbitrary and made up.
This is also incorrect. The rule is derived logically from the consistent properties of exponents, as demonstrated above. It's not an arbitrary invention but a direct consequence of the established mathematical framework.
Practical Applications of the Zero Exponent Rule
The zero exponent rule is not just a theoretical concept; it has significant practical applications across various fields, including:
- Algebra: Simplifying algebraic expressions involving exponents often utilizes this rule.
- Calculus: The rule plays a role in various calculus concepts, particularly when dealing with limits and derivatives.
- Computer Science: In programming and algorithms involving exponential functions, understanding the zero exponent rule is crucial.
- Physics and Engineering: Many scientific formulas involve exponents, and the zero exponent rule is essential for correct calculations.
Expanding on Exponents: Negative Exponents
Understanding the zero exponent rule provides a smooth transition to grasping negative exponents. Let's continue the pattern from before:
- 9⁴ = 6561
- 9³ = 729
- 9² = 81
- 9¹ = 9
- 9⁰ = 1
- 9⁻¹ = 1/9 (1/9)
- 9⁻² = 1/81 (1/9 * 1/9)
- 9⁻³ = 1/729 (1/81 * 1/9)
This reveals that a negative exponent indicates the reciprocal of the base raised to the positive exponent. Therefore, 9⁻² = 1/9² = 1/81.
This consistency in patterns reinforces the interconnectedness of mathematical rules and showcases the elegant structure of exponent properties.
Conclusion: Mastering the Power of Zero
The answer to "What is 9 to the power of 0?" is definitively 1. This isn't a random assignment; it's a logical consequence of the consistent properties of exponents. Understanding the zero exponent rule requires grasping the fundamental principles of exponents, recognizing patterns, and appreciating the underlying mathematical framework. By overcoming common misconceptions and appreciating its wide-ranging applications, you'll solidify your understanding of this crucial concept, empowering you to tackle more complex mathematical challenges with confidence. The power of zero is not nothingness; it's the foundation of a consistent and powerful mathematical system.
Latest Posts
Latest Posts
-
How Much 70 Fahrenheit In Celsius
Apr 03, 2025
-
All Living Things Are Made Of What
Apr 03, 2025
-
The Movement Of Water Through A Selectively Permeable Membrane
Apr 03, 2025
-
What Percent Is 3 Out Of 20
Apr 03, 2025
-
500 Ml Of Water Is How Many Ounces
Apr 03, 2025
Related Post
Thank you for visiting our website which covers about What Is 9 To The Power Of 0 . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.
