What Is 8/25 As A Percent
listenit
May 09, 2025 · 4 min read
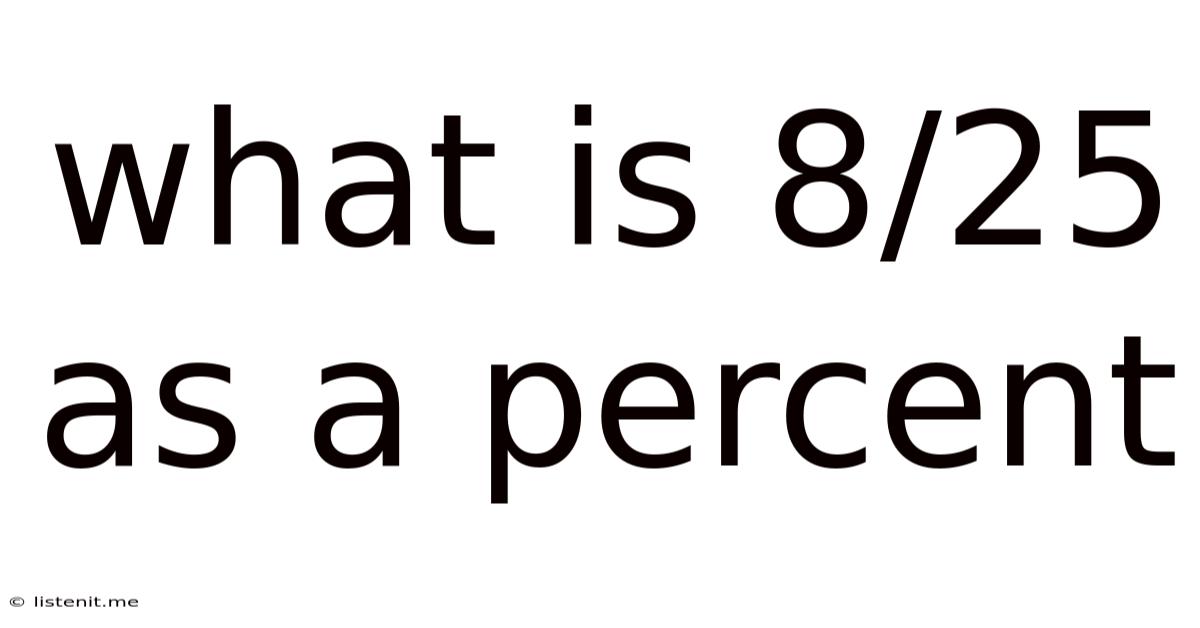
Table of Contents
What is 8/25 as a Percent? A Comprehensive Guide
Converting fractions to percentages is a fundamental skill in mathematics with widespread applications in everyday life, from calculating discounts and interest rates to understanding statistical data and analyzing financial reports. This comprehensive guide will delve into the process of converting the fraction 8/25 into a percentage, explaining the underlying principles and providing practical examples to solidify your understanding. We'll also explore various methods for tackling similar fraction-to-percentage conversions, equipping you with the knowledge to tackle a broad range of problems.
Understanding Fractions and Percentages
Before diving into the conversion, let's briefly review the concepts of fractions and percentages.
Fractions: A fraction represents a part of a whole. It's expressed as a ratio of two numbers: the numerator (top number) and the denominator (bottom number). The numerator indicates the number of parts we have, while the denominator indicates the total number of parts the whole is divided into. For example, in the fraction 8/25, 8 represents the parts we have, and 25 represents the total number of equal parts.
Percentages: A percentage is a way of expressing a number as a fraction of 100. The symbol "%" represents "per hundred" or "out of 100." Percentages are widely used to represent proportions, rates, and changes. For instance, 25% means 25 out of 100, or 25/100.
Method 1: Converting the Fraction to a Decimal, Then to a Percentage
This is arguably the most common and straightforward method. It involves two steps:
Step 1: Convert the fraction to a decimal. To do this, we divide the numerator (8) by the denominator (25):
8 ÷ 25 = 0.32
Step 2: Convert the decimal to a percentage. To convert a decimal to a percentage, we multiply the decimal by 100 and add the "%" symbol:
0.32 × 100 = 32%
Therefore, 8/25 is equal to 32%.
Method 2: Finding an Equivalent Fraction with a Denominator of 100
This method leverages the definition of a percentage as a fraction out of 100. We aim to find an equivalent fraction to 8/25 that has a denominator of 100.
To achieve this, we ask: "What number, when multiplied by 25, gives us 100?" The answer is 4 (25 x 4 = 100).
Since we multiply the denominator by 4, we must also multiply the numerator by 4 to maintain the equivalence of the fraction:
(8 × 4) / (25 × 4) = 32/100
Since 32/100 means 32 out of 100, this is equivalent to 32%.
Method 3: Using Proportions
This method utilizes the concept of proportions to solve the problem. We can set up a proportion to find the percentage:
8/25 = x/100
To solve for 'x' (the percentage), we cross-multiply:
25x = 800
Then, divide both sides by 25:
x = 800 ÷ 25 = 32
Therefore, x = 32, meaning 8/25 is equal to 32%.
Practical Applications of Fraction to Percentage Conversion
The ability to convert fractions to percentages is crucial in numerous real-world scenarios:
-
Calculating Discounts: A store offers a 20% discount on an item. If the original price is $50, the discount amount is (20/100) * $50 = $10. This involves converting the percentage (20%) back into a fraction to perform the calculation.
-
Understanding Interest Rates: Interest rates on loans and savings accounts are often expressed as percentages. Converting these percentages to fractions allows for accurate calculations of interest earned or paid.
-
Analyzing Statistical Data: Statistical data is frequently presented using percentages to represent proportions within a population or sample. Understanding how to convert fractions representing parts of the whole into percentages aids in the interpretation of this data.
-
Financial Reporting: Financial statements, such as income statements and balance sheets, often utilize percentages to present financial information in a more easily understandable format. Converting relevant fractions into percentages is vital for analysis and interpretation.
Advanced Techniques and Considerations
While the methods above are sufficient for most basic conversions, understanding some more advanced concepts can be beneficial:
-
Recurring Decimals: Some fractions, when converted to decimals, result in recurring decimals (e.g., 1/3 = 0.333...). When converting these to percentages, you might need to round to a certain number of decimal places for practical purposes.
-
Complex Fractions: Complex fractions (fractions within fractions) require an extra step before conversion to a percentage. Simplify the complex fraction first to obtain a single fraction before applying the conversion methods discussed above.
-
Using Calculators: Most calculators have a percentage function that simplifies the conversion process. Entering the fraction as a division (e.g., 8 ÷ 25) and then using the percentage function will directly give you the answer.
Conclusion: Mastering Fraction-to-Percentage Conversions
Converting fractions to percentages is a valuable skill applicable across various fields. Understanding the different methods – converting to decimals, finding equivalent fractions with a denominator of 100, and using proportions – provides flexibility and a deeper comprehension of the underlying mathematical principles. By mastering these techniques, you'll be well-equipped to tackle a wide range of problems involving fractions and percentages, enhancing your problem-solving abilities in both academic and real-world contexts. Remember to practice regularly to solidify your understanding and improve your speed and accuracy in performing these conversions. With consistent effort, you'll find these conversions become second nature.
Latest Posts
Latest Posts
-
How Radical Was The American Revolution
May 09, 2025
-
Are Bases Used In Cleaning Products
May 09, 2025
-
Whats A 12 Out Of 18
May 09, 2025
-
Which Of The Following Represent The Lewis Structure For N
May 09, 2025
-
How Many Combinations With 4 Items
May 09, 2025
Related Post
Thank you for visiting our website which covers about What Is 8/25 As A Percent . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.