What Is 5.8 As A Fraction
listenit
May 25, 2025 · 4 min read
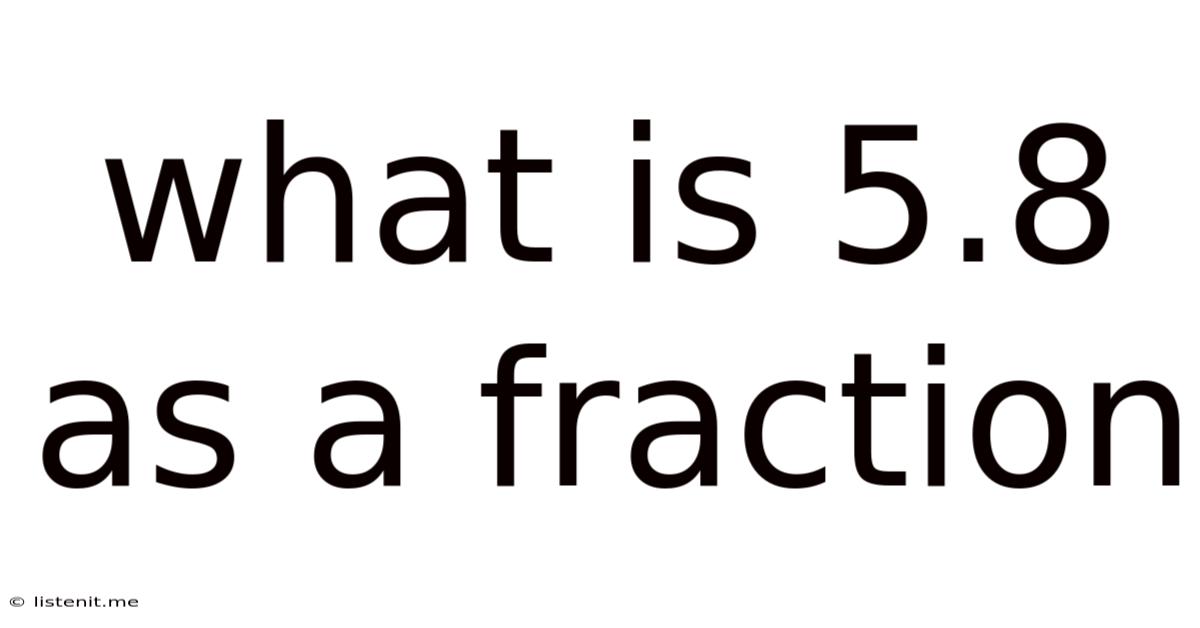
Table of Contents
What is 5.8 as a Fraction? A Comprehensive Guide
Understanding how to convert decimals to fractions is a fundamental skill in mathematics. This comprehensive guide will walk you through the process of converting the decimal 5.8 into a fraction, explaining the steps involved and providing additional context to solidify your understanding. We'll also explore related concepts and offer practical examples to enhance your learning experience.
Understanding Decimals and Fractions
Before diving into the conversion, let's briefly review the definitions of decimals and fractions.
Decimals: Decimals are a way of representing numbers that are not whole numbers. They use a decimal point to separate the whole number part from the fractional part. For example, in the number 5.8, the '5' represents the whole number part, and the '.8' represents the fractional part (eight-tenths).
Fractions: Fractions represent parts of a whole. They are expressed as a ratio of two numbers: the numerator (the top number) and the denominator (the bottom number). The denominator indicates the total number of equal parts the whole is divided into, and the numerator indicates how many of those parts are being considered. For instance, ½ represents one out of two equal parts.
Converting 5.8 to a Fraction: Step-by-Step Guide
The conversion of 5.8 to a fraction involves these key steps:
Step 1: Identify the Whole Number and Decimal Part
In the decimal 5.8, the whole number part is 5, and the decimal part is 0.8.
Step 2: Convert the Decimal Part to a Fraction
The decimal part, 0.8, can be written as a fraction by placing the digits after the decimal point over a denominator that is a power of 10. Since there's one digit after the decimal point, the denominator will be 10:
0.8 = 8/10
Step 3: Simplify the Fraction
The fraction 8/10 can be simplified by finding the greatest common divisor (GCD) of the numerator (8) and the denominator (10). The GCD of 8 and 10 is 2. Divide both the numerator and the denominator by the GCD:
8 ÷ 2 = 4 10 ÷ 2 = 5
This simplifies the fraction to 4/5.
Step 4: Combine the Whole Number and the Simplified Fraction
Now, combine the whole number (5) with the simplified fraction (4/5):
5 + 4/5 = 5 ⁴⁄₅
Therefore, 5.8 as a fraction is 5 ⁴⁄₅.
Alternative Method: Using Place Value
Another approach to convert 5.8 to a fraction involves understanding place value. The digit 8 is in the tenths place, meaning it represents 8/10. Therefore, 5.8 can be directly written as:
5 + 8/10
Then, simplify the fraction as shown in the previous method to arrive at 5 ⁴⁄₅.
Working with Other Decimals
Let's explore converting other decimals to fractions to further solidify your understanding.
Example 1: Converting 2.35 to a fraction
- Identify the whole number and decimal part: Whole number = 2, Decimal part = 0.35
- Convert the decimal part to a fraction: 0.35 = 35/100
- Simplify the fraction: The GCD of 35 and 100 is 5. 35 ÷ 5 = 7, 100 ÷ 5 = 20. The simplified fraction is 7/20.
- Combine the whole number and the simplified fraction: 2 + 7/20 = 2 ⁷⁄₂₀
Therefore, 2.35 as a fraction is 2 ⁷⁄₂₀.
Example 2: Converting 0.125 to a fraction
- Identify the whole number and decimal part: Whole number = 0, Decimal part = 0.125
- Convert the decimal part to a fraction: 0.125 = 125/1000
- Simplify the fraction: The GCD of 125 and 1000 is 125. 125 ÷ 125 = 1, 1000 ÷ 125 = 8. The simplified fraction is 1/8.
- Combine the whole number and the simplified fraction: 0 + 1/8 = 1/8
Therefore, 0.125 as a fraction is 1/8.
Example 3: Converting a repeating decimal to a fraction
Repeating decimals require a slightly different approach. Let's say we want to convert 0.333... (0.3 repeating) to a fraction. We can represent this as 'x':
x = 0.333...
Multiplying by 10:
10x = 3.333...
Subtracting the first equation from the second:
10x - x = 3.333... - 0.333...
9x = 3
x = 3/9
Simplifying: x = 1/3
Therefore, 0.333... as a fraction is 1/3. Similar techniques can be applied to other repeating decimals.
Importance of Understanding Decimal to Fraction Conversions
The ability to convert decimals to fractions is crucial for several reasons:
-
Problem-solving: Many mathematical problems require working with fractions, and understanding this conversion allows you to solve problems involving decimals more efficiently.
-
Accuracy: Fractions often provide a more accurate representation of numbers than decimals, especially when dealing with irrational numbers (like pi) or repeating decimals.
-
Understanding Ratios and Proportions: Fractions are fundamental to understanding ratios and proportions, which are used extensively in various fields, including science, engineering, and finance.
-
Real-world applications: Converting decimals to fractions is applicable in everyday life scenarios, such as measuring ingredients in cooking or calculating proportions in construction.
Conclusion
Converting decimals to fractions is a straightforward process once you understand the underlying principles. This guide has detailed the steps involved, provided examples, and highlighted the importance of this conversion in mathematics and beyond. By mastering this skill, you'll enhance your mathematical proficiency and broaden your ability to tackle a wider range of problems effectively. Remember to always simplify your fractions to their lowest terms for the most accurate and efficient representation.
Latest Posts
Latest Posts
-
Perimeter Of A Half Circle Calculator
May 25, 2025
-
How Many Months Is 18 Months
May 25, 2025
-
If Someone Is Born In 1987 How Old Are They
May 25, 2025
-
Greatest Common Factor For 12 And 48
May 25, 2025
-
How Many Days Is 630 Hours
May 25, 2025
Related Post
Thank you for visiting our website which covers about What Is 5.8 As A Fraction . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.