What Is 4 To The 5 Power
listenit
Apr 03, 2025 · 4 min read
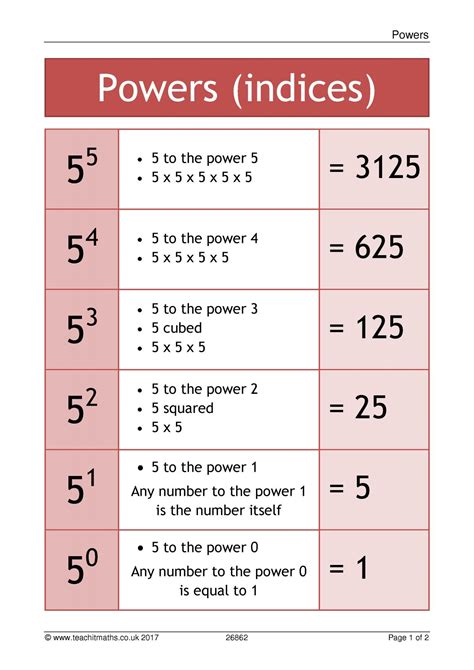
Table of Contents
What is 4 to the 5th Power? A Deep Dive into Exponents and Their Applications
Understanding exponents is fundamental to mathematics and numerous scientific fields. This comprehensive guide will explore the concept of "4 to the 5th power," or 4⁵, delving into its calculation, practical applications, and the broader context of exponential notation. We'll also touch upon related concepts like scientific notation and logarithmic scales to provide a holistic understanding.
Understanding Exponents: A Quick Refresher
Before we delve into the specifics of 4⁵, let's solidify our understanding of exponents. An exponent, also known as a power or index, indicates how many times a base number is multiplied by itself. It's written as a superscript to the right of the base number. For example, in the expression 4⁵, '4' is the base, and '5' is the exponent. This means 4 is multiplied by itself 5 times.
General Form: bⁿ = b × b × b × ... × b (n times), where 'b' is the base and 'n' is the exponent.
Key Terms to Remember
- Base: The number being multiplied repeatedly.
- Exponent: The number indicating how many times the base is multiplied by itself.
- Power: Another term for exponent.
Calculating 4 to the 5th Power (4⁵)
Now, let's calculate 4⁵. This simply means multiplying 4 by itself five times:
4⁵ = 4 × 4 × 4 × 4 × 4 = 1024
Therefore, 4 to the 5th power is 1024. This seemingly simple calculation has significant implications across various fields.
Real-World Applications of Exponents and 4 to the 5th Power
The concept of exponents, and specifically 4⁵, appears in diverse areas, often implicitly. Let's explore some examples:
1. Compound Interest Calculations
Imagine investing $1000 at an annual interest rate of 4%, compounded annually. After 5 years, the amount in your account would involve calculations using exponents:
Principal amount (P) = $1000 Interest rate (r) = 4% = 0.04 Number of years (n) = 5
Future Value (FV) = P (1 + r)ⁿ = $1000 (1 + 0.04)⁵ = $1000 (1.04)⁵ ≈ $1216.65
While this example doesn't directly use 4⁵, it highlights the power of exponential growth. The exponential function models how quickly your money grows over time.
2. Computer Science and Data Storage
In computer science, powers of 2 are prevalent. While not directly related to 4⁵, understanding exponents is essential for grasping concepts like memory addressing (powers of 2 represent memory locations), network addressing (IP addresses), and data compression algorithms. For instance, the number of possible combinations from a 10-bit binary number is 2¹⁰ = 1024, showcasing the direct relationship between powers of 2 and binary systems used in computing.
3. Population Growth Models
Exponential growth models are often used to predict population increases or decreases. The basic formula often involves an exponential term where the base represents the growth factor and the exponent signifies the number of periods (years, generations, etc.). While a growth factor of 4 might not be common for human populations, understanding exponential growth remains critical for analyzing various population dynamics. The 4⁵ calculation helps us understand the potential for rapid growth when the base is greater than 1.
4. Scientific Notation
Scientific notation is a way to represent very large or very small numbers concisely. It uses exponents of 10. For instance, 1024 can be written as 1.024 x 10³. Understanding exponents is crucial to manipulating and understanding numbers in scientific notation, frequently used in fields like astronomy and physics.
5. Logarithmic Scales
Logarithmic scales are used to represent data that spans several orders of magnitude. A classic example is the Richter scale for earthquake magnitude. Logarithmic scales utilize the inverse of exponential functions, emphasizing the relationship between exponents and logarithms.
Expanding on Exponential Concepts
Let's further explore some important aspects of working with exponents:
1. Properties of Exponents
Several rules govern operations with exponents:
- Product of Powers: aᵐ × aⁿ = aᵐ⁺ⁿ
- Quotient of Powers: aᵐ / aⁿ = aᵐ⁻ⁿ
- Power of a Power: (aᵐ)ⁿ = aᵐⁿ
- Power of a Product: (ab)ⁿ = aⁿbⁿ
- Power of a Quotient: (a/b)ⁿ = aⁿ/bⁿ (b ≠ 0)
Understanding these rules is crucial for simplifying complex exponential expressions.
2. Negative Exponents
A negative exponent indicates the reciprocal of the base raised to the positive exponent:
a⁻ⁿ = 1/aⁿ
For example, 4⁻² = 1/4² = 1/16. Negative exponents are often used in scientific notation to represent small numbers.
3. Fractional Exponents
Fractional exponents represent roots. For example, a^(1/n) is the nth root of 'a'.
a^(m/n) = (ⁿ√a)ᵐ
For example, 4^(3/2) = (√4)³ = 2³ = 8.
Conclusion: The Significance of 4 to the 5th Power and Beyond
While seemingly a simple calculation, 4⁵ = 1024 serves as a gateway to understanding the broader world of exponents. From compound interest to data storage, population modeling to scientific notation, exponents are fundamental mathematical tools with extensive applications. Mastering the concepts discussed here will enhance your ability to interpret and work with data in various fields. Remember, the seemingly simple calculation of 4 to the 5th power provides a foundational understanding that unlocks a deeper appreciation for the power and versatility of exponential functions. The principles discussed here are crucial for anyone looking to develop a strong mathematical foundation and a deeper understanding of data-driven applications in numerous disciplines.
Latest Posts
Latest Posts
-
What Is The Greatest Common Factor Of 36 And 60
Apr 04, 2025
-
How Do You Graph Y 3x 4
Apr 04, 2025
-
Is Ch3oh An Acid Or Base
Apr 04, 2025
-
How Many Isotopes Does Argon Have
Apr 04, 2025
-
What Is 2 To The 6th Power
Apr 04, 2025
Related Post
Thank you for visiting our website which covers about What Is 4 To The 5 Power . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.
