What Is 4 Divided By 1/6
listenit
May 23, 2025 · 5 min read
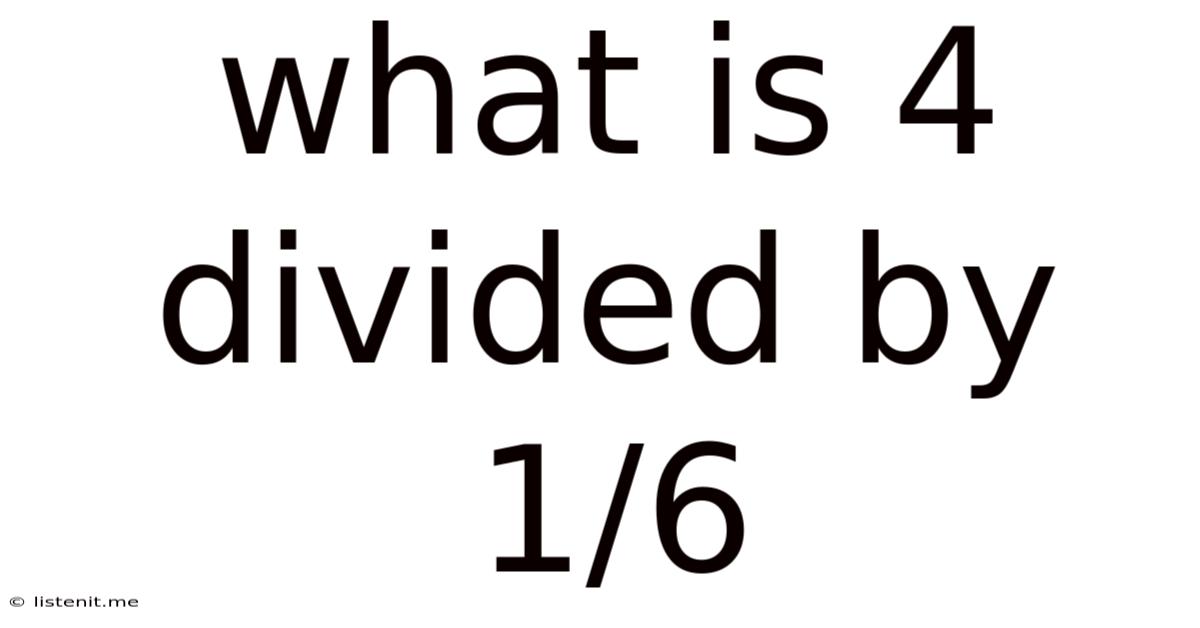
Table of Contents
What is 4 Divided by 1/6? A Deep Dive into Fraction Division
The seemingly simple question, "What is 4 divided by 1/6?", often trips up students and adults alike. While the answer itself is straightforward once you understand the underlying principles, the process of arriving at that answer offers a valuable opportunity to explore the intricacies of fraction division. This article will not only provide the solution but will also delve deep into the conceptual understanding, different methods of solving the problem, and real-world applications of this type of calculation.
Understanding Fraction Division: The "Invert and Multiply" Rule
The core concept behind dividing by a fraction is the reciprocal. The reciprocal of a fraction is simply the fraction flipped upside down. For instance, the reciprocal of 1/6 is 6/1 (or simply 6). This leads us to the well-known "invert and multiply" rule: To divide by a fraction, you multiply by its reciprocal.
This rule isn't arbitrary; it stems from the fundamental definition of division. Division asks, "How many times does the divisor fit into the dividend?" When dividing by a fraction, we're asking how many times a fraction fits into a whole number (or another fraction). Multiplying by the reciprocal elegantly addresses this question.
Solving 4 Divided by 1/6: Step-by-Step
Let's apply the "invert and multiply" rule to solve our problem:
1. Identify the dividend and divisor:
- Dividend: 4
- Divisor: 1/6
2. Find the reciprocal of the divisor:
The reciprocal of 1/6 is 6/1, or simply 6.
3. Multiply the dividend by the reciprocal of the divisor:
4 ÷ (1/6) = 4 × 6 = 24
Therefore, 4 divided by 1/6 is 24.
Visualizing the Solution
While the "invert and multiply" rule provides a quick and efficient method, visualizing the problem can enhance understanding. Imagine you have 4 pizzas, and you want to divide each pizza into slices of 1/6. How many slices will you have in total?
You'll have 6 slices per pizza, and with 4 pizzas, you'll have 6 slices/pizza * 4 pizzas = 24 slices. This visual representation directly mirrors the mathematical operation.
Alternative Methods: Using Complex Fractions
Another approach involves expressing the division as a complex fraction:
4 ÷ (1/6) = 4 / (1/6)
To simplify a complex fraction, you multiply both the numerator and the denominator by the reciprocal of the denominator:
(4 / (1/6)) × (6/1) / (6/1) = (4 × 6) / (1 × 1) = 24/1 = 24
This method demonstrates the same underlying principle—multiplying by the reciprocal—but from a different perspective.
Expanding the Concept: Dividing Fractions by Fractions
Let's extend our understanding to scenarios where both the dividend and the divisor are fractions. For example: (2/3) ÷ (1/4).
Following the "invert and multiply" rule:
(2/3) ÷ (1/4) = (2/3) × (4/1) = 8/3
This simplifies to 2 and 2/3. The process remains identical; the only difference lies in working with fractions throughout the calculation.
Real-World Applications: Where Fraction Division is Used
Fraction division isn't just a theoretical exercise; it appears in many practical situations:
-
Cooking and Baking: Recipes often require dividing ingredients into fractions. For example, if a recipe calls for 1/2 cup of flour and you want to make 1/4 of the recipe, you'd need to calculate (1/2) ÷ 4 = 1/8 cup of flour.
-
Sewing and Tailoring: Cutting fabric requires precise measurements. If you need to cut a piece of fabric that is 1/3 of a yard long and you have 2/3 of a yard of fabric, you'd perform (2/3) ÷ (1/3) to determine how many pieces you can cut.
-
Construction and Engineering: Precise measurements are crucial in construction. Dividing lengths, areas, and volumes frequently involves fractions. For example, calculating the number of tiles needed for a section of a wall might involve dividing a total area by the area of a single tile.
-
Data Analysis: Working with datasets often involves dividing proportions or percentages, which can be expressed as fractions. Analyzing survey results or comparing the sizes of different data groups regularly involves fraction division.
Common Mistakes and How to Avoid Them
Several common errors can occur when performing fraction division:
-
Forgetting to invert the divisor: The most frequent mistake is failing to find the reciprocal of the divisor before multiplying. Always remember the crucial step of inverting the fraction you are dividing by.
-
Incorrect multiplication of fractions: Once you have inverted, it's essential to correctly multiply the numerators and denominators. Double-check your work to avoid errors in fraction multiplication.
-
Simplifying fractions incorrectly: After performing the multiplication, make sure you correctly simplify the resulting fraction to its lowest terms.
Strengthening Your Fraction Skills: Practice and Resources
Mastering fraction division requires consistent practice. Start with simple problems and gradually increase the complexity. Many online resources and textbooks offer exercises and explanations to aid your learning.
Focus on understanding the underlying concepts, not just memorizing the rules. By grasping the meaning of division and the concept of reciprocals, you can confidently tackle various fraction division problems and apply your skills in real-world situations.
Conclusion: Embracing the Power of Fraction Division
While seemingly straightforward, the question "What is 4 divided by 1/6?" opens up a world of understanding regarding fraction manipulation. By grasping the "invert and multiply" rule and its underlying rationale, you gain a valuable skill applicable in numerous contexts. Mastering fraction division empowers you to tackle more complex mathematical problems and confidently apply this essential skill to various real-world scenarios. Remember to practice consistently and utilize available resources to reinforce your learning and build a strong mathematical foundation. The more you practice, the more confident and proficient you will become in handling fraction division.
Latest Posts
Latest Posts
-
Opportunity Cost Of Security Deposit Calculator
May 23, 2025
-
50 Out Of 70 As A Percentage
May 23, 2025
-
When Is 7 Months From Now
May 23, 2025
-
100 To The Power Of 5
May 23, 2025
-
How Many Hours In 1 Mile
May 23, 2025
Related Post
Thank you for visiting our website which covers about What Is 4 Divided By 1/6 . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.