What Is 30 Percent Of 40 Dollars
listenit
May 24, 2025 · 5 min read
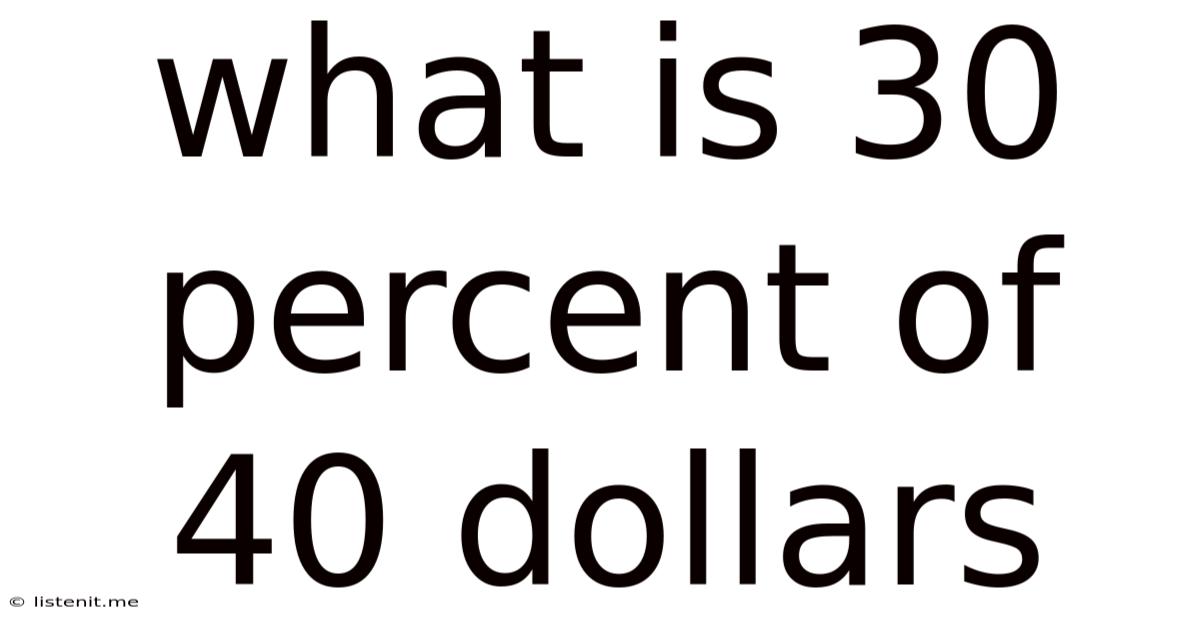
Table of Contents
What is 30 Percent of 40 Dollars? A Deep Dive into Percentage Calculations
Calculating percentages is a fundamental skill in various aspects of life, from managing personal finances to understanding business metrics. This article will not only answer the question, "What is 30 percent of 40 dollars?" but also delve into the underlying principles of percentage calculations, explore different methods for solving such problems, and demonstrate practical applications in everyday scenarios.
Understanding Percentages
A percentage is a fraction or ratio expressed as a number out of 100. The symbol "%" represents "percent," meaning "out of 100." Therefore, 30% means 30 out of 100, or 30/100, which simplifies to 3/10. This fundamental understanding is crucial for solving percentage problems.
Calculating 30% of 40 Dollars: Method 1 - Using Fractions
The most straightforward method involves converting the percentage to a fraction and then multiplying it by the total amount.
Steps:
- Convert the percentage to a fraction: 30% = 30/100 = 3/10
- Multiply the fraction by the total amount: (3/10) * $40 = $12
Therefore, 30% of 40 dollars is $12.
Calculating 30% of 40 Dollars: Method 2 - Using Decimal Equivalents
Another common approach involves converting the percentage to its decimal equivalent before performing the multiplication.
Steps:
- Convert the percentage to a decimal: 30% = 30 ÷ 100 = 0.30
- Multiply the decimal by the total amount: 0.30 * $40 = $12
Again, the answer is $12.
Calculating 30% of 40 Dollars: Method 3 - Using Proportions
This method utilizes the concept of proportions, setting up an equation to solve for the unknown value.
Steps:
- Set up a proportion: x / $40 = 30 / 100
- Cross-multiply: 100x = 30 * $40
- Solve for x: 100x = $1200 => x = $1200 / 100 => x = $12
The result, as expected, is $12.
Practical Applications of Percentage Calculations
The ability to calculate percentages has wide-ranging applications in numerous fields:
1. Personal Finance
- Discounts: Calculating discounts on sale items is a prime example. If a $40 shirt is discounted by 30%, you save $12, paying only $28.
- Taxes: Determining sales tax or income tax involves calculating percentages. For example, if the sales tax is 6%, you'd calculate 6% of the purchase price.
- Tips: Calculating a tip at a restaurant usually involves determining a percentage of the total bill. A 15% tip on a $50 meal would be $7.50.
- Savings and Investments: Understanding interest rates on savings accounts or investment returns relies heavily on percentage calculations.
2. Business and Economics
- Profit Margins: Businesses calculate profit margins as a percentage of revenue to assess their profitability.
- Market Share: Companies track their market share as a percentage of the total market size.
- Growth Rates: Economic growth is often expressed as a percentage increase or decrease.
- Inflation: The rate of inflation, representing the increase in the general price level, is expressed as a percentage.
3. Science and Statistics
- Data Analysis: Percentages are frequently used to represent data in various charts and graphs, such as pie charts and bar graphs.
- Probability: Probability calculations often involve percentages to express the likelihood of an event occurring.
- Scientific Measurements: Many scientific measurements use percentages to represent ratios or proportions.
Advanced Percentage Calculations: Beyond the Basics
While calculating 30% of 40 dollars is relatively simple, more complex scenarios require additional steps and understanding.
1. Calculating Percentage Increase or Decrease
Determining the percentage increase or decrease between two values is a common task. For instance, if a stock price increases from $20 to $25, the percentage increase is calculated as follows:
- Find the difference: $25 - $20 = $5
- Divide the difference by the original value: $5 / $20 = 0.25
- Multiply by 100 to express as a percentage: 0.25 * 100 = 25%
The stock price increased by 25%.
2. Finding the Original Value
Sometimes, you know the percentage and the resulting value, and you need to find the original value. For example, if 30% of a number is $12, what is the original number?
- Set up an equation: 0.30 * x = $12
- Solve for x: x = $12 / 0.30 = $40
The original number is $40.
3. Calculating Percentage Change with Multiple Changes
When dealing with multiple percentage changes, the calculations aren't as straightforward as simply adding or subtracting the percentages. For example, a 10% increase followed by a 10% decrease does not result in the original value. You must calculate each change sequentially.
Mastering Percentage Calculations: Tips and Tricks
- Practice regularly: The more you practice, the more comfortable you'll become with percentage calculations.
- Use a calculator: For more complex calculations, a calculator can save time and prevent errors.
- Understand the underlying principles: A strong grasp of fractions and decimals is essential.
- Break down complex problems: Divide larger problems into smaller, manageable steps.
- Check your work: Always double-check your calculations to ensure accuracy.
Conclusion
Understanding percentage calculations is a vital life skill with numerous applications. The seemingly simple question, "What is 30 percent of 40 dollars?" serves as a gateway to exploring a wide range of percentage-based problems. By mastering the various methods and understanding the underlying principles, you can confidently tackle percentage calculations in various contexts, from managing personal finances to interpreting business data and more. Remember to practice regularly, utilize tools where necessary, and always double-check your work to ensure accuracy. This will significantly improve your problem-solving skills and enhance your overall understanding of numbers and their real-world applications.
Latest Posts
Latest Posts
-
What Day Will It Be 28 Days From Now
May 24, 2025
-
What Is The Least Common Multiple Of 11 And 9
May 24, 2025
-
5 1 6 As An Improper Fraction
May 24, 2025
-
6 To The Power Of 30
May 24, 2025
-
If I M 57 What Year Was I Born
May 24, 2025
Related Post
Thank you for visiting our website which covers about What Is 30 Percent Of 40 Dollars . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.